AP Board 9th Class Maths Solutions Chapter 4 Lines and Angles Ex 4.2

AP State Syllabus AP Board 9th Class Maths Solutions Chapter 4 Lines and Angles Ex 4.2 Textbook Questions and Answers.

AP State Syllabus 9th Class Maths Solutions 4th Lesson Lines and Angles Exercise 4.2

Question 1.
In the given figure three lines \(\overline{\mathrm{AB}}\) , \(\overline{\mathrm{CD}}\) and \(\overline{\mathrm{EF}}\) intersecting at ‘O’. Find the values of x, y and z, it is being given that x : y : z = 2 : 3 : 5

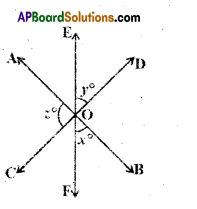
Solution:
From the figure
2x + 2y + 2z = 360°
⇒ x + y + z = 180°
x : y : z = 2 : 3 : 5
Sum of the terms of the ratio
= 2 + 3 + 5 = 10

Question 2.
Find the value of x in the following figures.
i)

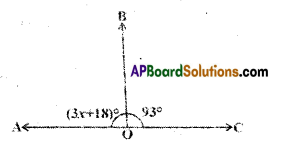
Solution:
From the figure
3x + 18= 180° – 93° (∵ linear pair )
3x + 18 = 87
3x = 87- 18 = 69
∴ x = \(\frac{69}{3}\) = 23

ii)

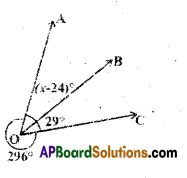
Solution:
From the figure
(x – 24)° + 29° + 296° = 360“
(∵ complete angle)
x + 301° = 360°
∴ x = 360° – 301° = 59°

iii)

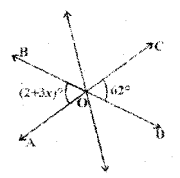
Solution:
From the figure
2 + 3x = 62
(∵ vertically opposite angles)
3x = 62 – 2
∴ 3x = 60° ⇒ x = \(\frac{60}{3}\)
∴ x = 20°

iv)

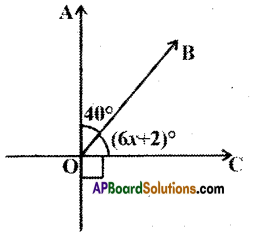
Solution:
From the figure
40 + (6x + 2) = 90°
(∵ complementary angles)
6x = 90° – 42°
6x = 48
x = \(\frac{48}{6}\) = 8°

Question 3.
In the given figure lines \(\overline{\mathrm{AB}}\) and \(\overline{\mathrm{CD}}\) intersect at ’O’. If ∠AOC + ∠BOE = 70° and ∠BOD = 40°, find ∠BOE and reflex ∠COE.

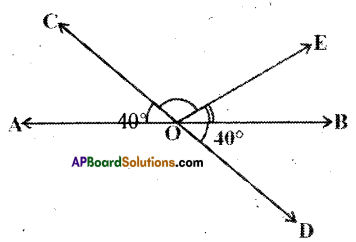
Solution:
Given that ∠AOC +∠BOE = 70°
∠BOD = 40°
∠AOC = 40°
(∵ ∠AOC, ∠BOD are vertically opposite angles)
∴ 40° + ∠BOE = 70°
⇒ ∠BOE = 70° – 40° = 30°
Also ∠AOC + ∠COE +∠BOE = 180°
( ∵ AB is a line)
⇒ 40° + ∠COE + 30° = 180°
⇒ ∠COE = 180° -70° = 110°
∴ Reflex ∠COE = 110°

Question 4.
In the given figure lines \(\overline{\mathrm{XY}}\) and \(\overline{\mathrm{MN}}\) . intersect at O. If ∠POY = 90° and a : b = 2:3, find c.

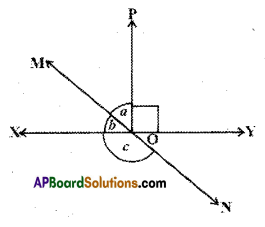
Solution:
Given that XY and MN are lines.
∠POY = 90°
a : b = 2 : 3
From the figure a + b = 90°
Sum of the terms of the ratio a : b
= 2 + 3 = 5
∴ b = \(\frac{3}{5}\) x 90° = 54°
From the figure b + c = 180°
(∵ linear pair of angles)
54° + c = 180°
c = 180°-54° = 126°

Question 5.
In the given figure ∠PQR = ∠PRQ, then prove that ∠PQS = ∠PRT.

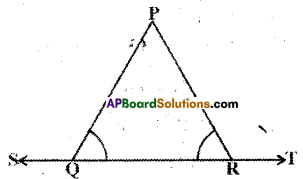
Solution:
Given that ∠PQR = ∠PRQ
From the figure
∠PQR + ∠PQS = 180° ………….. (1)
∠PRQ + ∠PRT = 180° …………..(2)
From (1) and (2)
∠PQR + ∠PQS = ∠PRQ + ∠PRT
But ∠PQR = ∠PRQ
So ∠PQS = ∠PRT
Hence proved.

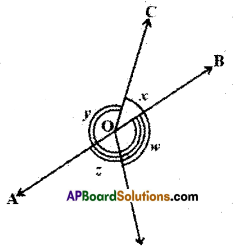
Question 6.
In the given figure, if x + y = w + z, then prove that AOB is a line.

Solution:
Given that x + y = w + z = k say
From the figure
x + y + z + w = 360°
(∵ Angle around a point)
Also x + y = z + w
∴ x + y = z + w = \(\frac{360^{\circ}}{2}\)
∴ x + y = z + w = 180°

OR

k + k = 360°
2k = 360°
k = \(\frac{360^{\circ}}{2}\)

(i.e.) (x,y) and (z, w) are pairs of adjacent angles whose sum is 180°.
In other words (x, y) and (z, w) are linear pair of angles ⇒ AOB is a line.

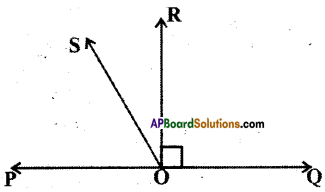
Question 7.
In the given figure \(\overline{\mathrm{PQ}}\) is a line. Ray \(\overline{\mathrm{OR}}\) is perpendicular to line \(\overline{\mathrm{PQ}}\).\(\overline{\mathrm{OS}}\) os is another ray lying between rays \(\overline{\mathrm{OP}}\) and \(\overline{\mathrm{OR}}\) Prove that

Solution:
Given : OR ⊥ PQ ⇒ ∠ROQ = 90°
To prove: ∠ROS = \(\frac{1}{2}\) (∠QOS – ∠POS)
Solution:
Proof: From the figure
∠ROS = ∠QOS – ∠QOR ……………(1)
∠ROS = ∠ROP – ∠POS ……………..(2)
Adding (1) and (2)
∠ROS + ∠ROS = ∠QOS – ∠QOR +∠ROP – ∠POS [ ∵ ∠QOR = ∠ROP = 90° given]
⇒ 2∠ROS = ∠QOS – ∠POS
⇒ ∠ROS = \(\frac{1}{2}\) [∠QOS – ∠POS]
Hence proved.

Question 8.
It is given that ∠XYZ = 64° and XY is produced to point P. A ray \(\overline{\mathrm{YQ}}\) bisects ∠ZYP. Dräw a figure from the given Information. Find ∠XYQ and reflex ∠QYP.
Solution:

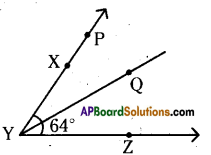
∠XYQ = 32°
∠QYP = 32°