AP State Syllabus SSC 10th Class Maths Solutions 13th Lesson Probability InText Questions
AP State Board Syllabus AP SSC 10th Class Maths Textbook Solutions Chapter 13 Probability InText Questions and Answers.
10th Class Maths 13th Lesson Probability InText Questions and Answers
Do This
(Page No. 307)
Outcomes of which of the following experiments are equally likely ?
Question 1.
Getting a digit 1, 2, 3, 4, 5 or 6 when a die is rolled.
Answer:
Equally likely.
![]()
Question 2.
Picking a different colour ball from a bag of 5 red balls, 4 blue balls and 1 black ball.
Note: Picking two different colour balls …………..
i.e., picking a red or blue or black ball from a …………
Answer:
Not equally likely.
Question 3.
Winning in a game of carrom.
Answer:
Equally likely.
Question 4.
Units place of a two digit number selected may be 0, 1, 2, 3, 4, 5, 6, 7, 8 or 9.
Answer:
Equally likely.
Question 5.
Picking a different colour ball from a bag of 10 red balls, 10 blue balls and 10 black balls.
Answer:
Equally likely.
Question 6.
a) Raining on a particular day of July.
Answer:
Not equally likely.
b) Are the outcomes of every experiment equally likely?
Answer:
Outcomes of all experiments need not necessarily be equally likely.
c) Give examples of 5 experiments that have equally likely outcomes and five more examples that do not have equally likely outcomes.
Answer:
Equally likely events:
- Getting an even or odd number when a die is rolled.
- Getting tail or head when a coin is tossed.
- Getting an even or odd number when a card is drawn at random from a pack of cards numbered from 1 to 10.
- Drawing a green or black ball from a bag containing 8 green balls and 8 black balls.
- Selecting a boy or girl from a class of 20 boys and 20 girls.
- Drawing a red or black card from a deck of cards.
Events which are not equally likely:
- Getting a prime or composite number when a die is thrown.
- Getting an even or odd number when a card is drawn at random from a pack of cards numbered from 1 to 5.
- Getting a number which is a multiple of 3 or not a multiple of 3 from numbers 1, 2, …… 10.
- Getting a number less than 5 or greater than 5.
- Drawing a white ball or green ball from a bag containing 5 green balls and 8 white balls.
![]()
Question 7.
Think of 5 situations with equally likely events and find the sample space. (Page No. 309)
Answer:
a) Tossing a coin: Getting a tail or head when a coin is tossed.
Sample space = {T, H}.
b) Getting an even or odd number when a die is rolled.
Sample space = (1, 2, 3, 4, 5, 6}.
c) Winning a game of shuttle.
Sample space = (win, loss}.
d) Picking a black or blue ball from a bag containing 3 blue balls and 3 blackballs = {blue, black}.
e) Drawing a blue coloured card or black coloured card from a deck of cards = {black, red}.
Question 8.
i) Is getting a head complementary to getting a tail? Give reasons. (Page No. 311)
Answer:
Number of outcomes favourable to head = 1
Probability of getting a head = \(\frac{1}{2}\) [P(E)]
Number of outcomes not favourable to head = 1
Probability of not getting a head = \(\frac{1}{2}\) [P(\(\overline{\mathrm{E}}\))]
Now P(E) + P(\(\overline{\mathrm{E}}\)) = \(\frac{1}{2}\) + \(\frac{1}{2}\) = 1
∴ Getting a head is complementary to getting a tail.
ii) In case of a die is getting a 1 comple-mentary to events getting 2, 3, 4, 5, 6? Give reasons for your answer.
Answer:
Yes. Complementary events.
∵ Probability of getting 1 = \(\frac{1}{6}\) [P(E)]
Probability of getting 2, 3, 4, 5, 6 = P(E) = P(\(\overline{\mathrm{E}}\)) = \(\frac{5}{6}\)
P(E) + P(\(\overline{\mathrm{E}}\)) = \(\frac{1}{6}\) + \(\frac{5}{6}\) = \(\frac{6}{6}\) = 1
iii) Write of five new pair of events that are complementary.
Answer:
- When a dice is thrown, getting an even number is complementary to getting an odd number.
- Drawing a red card from a deck of cards is complementary to getting a black card.
- Getting an even number is complementary to getting an odd number from numbers 1, 2, ….. 8.
- Getting a Sunday is complementary to getting any day other than Sunday in a week.
- Winning a running race is complementary to loosing it.
![]()
Try This
Question 1.
A child has a dice whose six faces show the letters A, B, C, D, E and F. The dice is thrown once. What is the probability of getting (i) A? (ii) D? (Page No. 312)
Answer:
Total number of outcomes (A, B, C, D, E and F) = 6.
i) Number of favourable outcomes to A = 1
Probability of getting A =
P(A) = \(\frac{\text { No.of favourable outcomesto } \mathrm{A}}{\text { No.of all possible outcomes }}\) = \(\frac{1}{6}\)
ii) No. of outcomes favourable to D = 1
Probability of getting D
= \(\frac{\text { No.of outcomes favourble to } \mathrm{D}}{\text { All possible outcomes }}\) = \(\frac{1}{6}\)
Question 2.
Which of the following cannot be the probability of an event? (Page No. 312)
(a) 2.3
(b) -1.5
(c) 15%
(d) 0.7
Answer:
a) 2.3 – Not possible
b) -1.5 – Not possible
c) 15% – May be the probability
d) 0.7 – May be the probability
Question 3.
You have a single deck of well shuffled cards. Then, what is the probability that the card drawn will be a queen? (Page No. 313)
Answer:
Number of all possible outcomes = 4 × 13 = 1 × 52 = 52
Number of outcomes favourable to Queen = 4 [♥ Q, ♦ Q, ♠ Q, ♣ Q]
∴ Probability P(E) = \(\frac{\text { No. of favourable outcomes }}{\text { Total no. of outcomes }}\)
= \(\frac{4}{52}\) = \(\frac{1}{13}\)
![]()
Question 4.
What is the probability that it is a face card? (Page No. 314)
Answer:
Face cards are J, Q, K.
∴ Number of outcomes favourable to face card = 4 × 3 = 12
No. of all possible outcomes = 52
P(E) = \(\frac{\text { No. of favourable outcomes }}{\text { Total no. of outcomes }}\)
= \(\frac{12}{52}\) = \(\frac{3}{13}\)
Question 5.
What is the probability that it is a spade? (Page No. 314)
Answer:
Number of spade cards = 13
Total number of cards = 52
Probability
= \(\frac{\text { Number of outcomes favourable to spades }}{\text { Number of all outcomes }}\)
= \(\frac{13}{52}\) = \(\frac{1}{4}\)
Question 6.
What is the probability that is the face card of spades? (Page No. 314)
Answer:
Number of outcomes favourable to face cards of spades = (K, Q, J) = 3
Number of all outcomes = 52
P(E) = \(\frac{3}{52}\)
Question 7.
What is the probability it is not a face card? (Page No. 314)
Answer:
Probability of a face card = \(\frac{12}{52}\) from (1)
∴ Probability that the card is not a face card
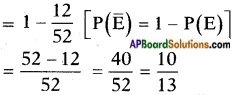
(or)
Number of favourable outcomes = 4 × 10 = 40
Number of all outcomes = 52
∴ Probability
= \(\frac{\text { No. of favourable outcomes }}{\text { Total no. of outcomes }}\)
= \(\frac{40}{52}\) = \(\frac{10}{13}\)
![]()
Think & Discuss
(Page No. 312)
Question 1.
Why is tossing a coin considered to be a fair way of deciding which team should get the ball at the beginning of any game?
Answer:
Probability of getting a head is \(\frac{1}{2}\) and of a tail is \(\frac{1}{2}\) are equal.
Hence tossing a coin is a fair way.
Question 2.
Can \(\frac{7}{2}\) be the probability of an event? Explain.
Answer:
\(\frac{7}{2}\) can’t be the probability of any event.
Since probability of any event should lie between 0 and 1.
Question 3.
Which of the following arguments are correct and which are not correct? Give reasons.
i) If two coins are tossed simultaneously, there are three possible outcomes – two heads, two tails or one of each. Therefore, for each of these outcomes, the probability is \(\frac{1}{3}\).
Answer:
False.
Reason:
All possible outcomes are 4
HH, HT, TH, TT
Thus, probability of two heads = \(\frac{1}{4}\)
Probability of two tails = \(\frac{1}{4}\)
Probability of one each = \(\frac{2}{4}\) = \(\frac{1}{2}\).
![]()
ii) If a dice is thrown, there are two possible outcomes – an odd number or an even number. Therefore, the probability of getting an odd number is \(\frac{1}{2}\).
Answer:
True.
Reason:
All possible outcomes = (1, 2, 3, 4, 5, 6) = 6
Outcomes favourable to an odd number (1, 3, 5) = 3
Outcomes favourable to an even number = (2, 4, 6) = 3
∴ Probability (odd number)
= \(\frac{\text { No. of favourable outcomes }}{\text { Total no. of outcomes }}\)
= \(\frac{3}{6}\) = \(\frac{1}{2}\).