AP State Syllabus SSC 10th Class Maths Solutions 9th Lesson Tangents and Secants to a Circle Optional Exercise
AP State Board Syllabus AP SSC 10th Class Maths Textbook Solutions Chapter 9 Tangents and Secants to a Circle Optional Exercise Textbook Questions and Answers.
10th Class Maths 9th Lesson Tangents and Secants to a Circle Optional Exercise Textbook Questions and Answers
Question 1.
Prove that the angle between the two tangents drawn from an external point to a circle is supplementary to the angle subtended by the line – segment joining the points of contact at the centre.
Answer:
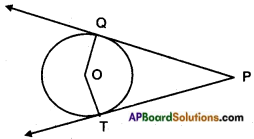
Given: A circle with centre ‘O’.
Two tangents \(\overleftrightarrow{\mathrm{PQ}}\) and \(\overleftrightarrow{\mathrm{PT}}\) from an external point P. Let Q, T be the points of contact.
R.T.P: ∠P and ∠QOT are supplementary.
Proof: OQ ⊥ PQ
[∵ radius is perpendicular to the tangent at the point of contact] also OT ⊥ PT
∴ ∠OQP + ∠OTP = 90° + 90° = 180° Nowin oPQOT,
∠OTP + ∠TPQ + ∠PQO + ∠QOT
= 360° (angle sum property)
180° + ∠P + ∠QOT = 360°
∠P + ∠QOT = 360°- 180° = 180° Hence proved. (Q.E.D.)
![]()
Question 2.
PQ is a chord of length 8 cm of a circle of radius 5 cm. The tangents at P and Q intersect at a point T (See figure). Find the length of TP.
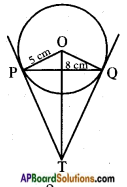
Answer:
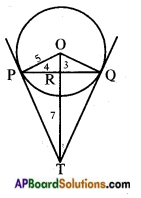
Given: PQ = 8
⇒ PR = 4
⇒ PO2 = PR2 + OR2
⇒ 25 = 16 + OR2
⇒ OR = 3
Now let RT = x and PT in △OPT, ∠P = 90°
∴ OT is hypotenuse.
∴ OT2 = OP2 + PT2
(Pythagoras theorem)
(3 + x)2 = 52 + y2 …….. (1)
and in △PRT, ∠R = 90°
∴ \(\overline{\mathrm{PT}}\) is hypotenuse.
∴ PT2 = PR2 + RT2
y2 = 42 + x2 …….. (2)
Now putting the value of y2 = 42 + x2 in equation (1) we got
(3 + x)2 = 52 + x2 + 42
9 + x2 + 6x = 25 + 16 + x2
6x = 25 + 16 – 9 = 25 + 7 = 32
⇒ x = \(\frac{32}{6}\) = \(\frac{16}{3}\)
Now from equation (2), we get

![]()
Question 3.
Prove that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
Answer:
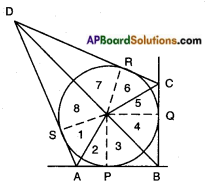
Given: Let a circle with centre ‘O’ touches the sides AB, BC, CD and DA of a quadrilateral ABCD at the points P, Q, R and S respectively.
R.T.P: ∠AOB + ∠COD = 180°
∠AOD + ∠BOC = 180°
Construction: Join OP, OQ, OR and OS.
Proof: Since the two tangents drawn from an external point of a circle subtend equal angles.
At the centre,
∴ ∠1 = ∠2
∠3 = ∠4 (from figure)
∠5 = ∠6
∠7 = ∠8
Now, ∠1 + ∠2 + ∠3 + ∠4 + ∠5 + ∠6 + ∠7 + ∠8 = 360°
[∵ Sum of all the angles around a point is 360°]
So, 2 (∠2 + ∠3 + ∠6 + ∠7) = 360°
and 2 (∠1 + ∠8 + ∠4 + ∠5) = 360°
(∠2 + ∠3) + (∠6 + ∠7) = \(\frac{360}{2}\) = 180°
Also, (∠1 + ∠8) + (∠4 + ∠5) = \(\frac{360}{2}\) = 180°
So, ∠AOB + ∠COD = 180°
[∵ ∠2 + ∠3 = ∠AOB;
∠6 + ∠7 = ∠COD
∠1 + ∠8 = ∠AOD
and ∠4 + ∠5 = ∠BOC [from fig.]]
and ∠AOD + ∠BOC = 180°
![]()
Question 4.
Draw a line segment AB of length 8 cm. Taking A as centre, draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Construct tangents to each circle from the centre of the other circle.
Answer:
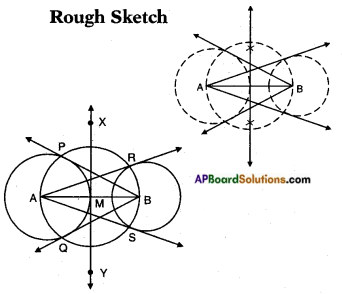
Steps of construction:
- Draw a line segment AB of length 8 cm.
- With A and B as centres and 4 cm, 3 cm as radius draw two circles.
- Draw the perpendicular bisectors \(\stackrel{\leftrightarrow}{\mathrm{XY}}\) of AB. Let \(\stackrel{\leftrightarrow}{\mathrm{XY}}\) and AB meet at M.
- Taking M as centre and MA or MB as radius draw a circle which cuts the circle with centre A at P and Q and circle with centre B at R, S.
- Join BP, BQ and AR, AS.
Question 5.
Let ABC be a right triangle in which AB = 6 cm, BC = 8 cm and ∠B = 90°. BD is the perpendicular from B on AC. The circle through B, C, D is drawn. Construct the tangents from A to this circle.
Answer:
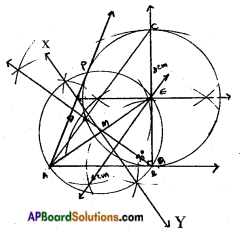
Steps of construction:
- Draw AABC such that AB = 6 cm; ∠B = 90° and BC – 8 cm.
- Drop a perpendicular BD from B on AC.
- Draw the circumcircle to ABCD. Let ‘E’ be its centre.
- Join AE and draw its perpendicular bisector \(\stackrel{\leftrightarrow}{\mathrm{XY}}\). Let it meet AE at M.
- Taking M as centre and MA or ME as radius draw a circle, which’ cuts the circumcircle of △BCD at P and B.
- Join AP and extend AB, which are the required tangents.
![]()
Question 6.
Find the area of the shaded region in the figure, given in which two circles with centres A and B touch each other at the point C. If AC = 8 cm. and AB = 3 cm.
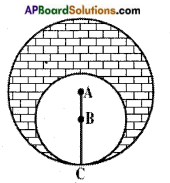
Answer:
Given: Two circles with centres A and B, whose radii are 8 cm and 5 cm.
[∵ AC = 8 cm, AB = 3 cm ⇒ BC = 8 – 3 = 5 cm]
Area of the shaded region = (Area of the larger circle) – (Area of the smaller circle)
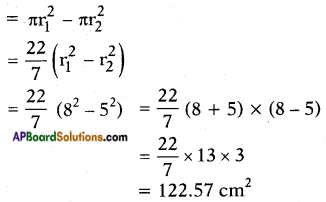
Question 7.
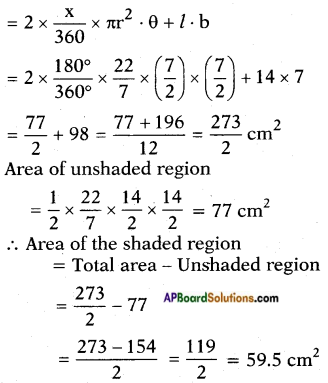
ABCD is a rectangle with AB = 14 cm. and BC = 7 cm. Taking DC, BC and AD as diameters, three semicircles are drawn as shown in the figure. Find the area of the shaded region.
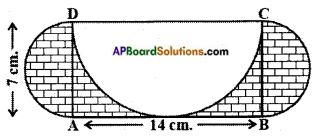
Answer:
Given AB = 14 cm, AD = BC = 7 cm Area of the shaded and unshaded region
= (2 × Area of the semi-circles with AD as diameter) + Area of the rectangle