Students get through Maths 1A Important Questions Inter 1st Year Maths 1A Trigonometric Equations Important Questions which are most likely to be asked in the exam.
Intermediate 1st Year Maths 1A Trigonometric Equations Important Questions
Question 1.
Solve sin x = \(\frac{1}{\sqrt{2}}\)
Solution:
sin x = \(\frac{1}{\sqrt{2}}\) = sin \(\frac{\pi}{4}\) and \(\frac{\pi}{4}\) ∈ [-\(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
∴ x = \(\frac{\pi}{4}\) is the principal solution and
x = nπ + (-1)n \(\frac{\pi}{4}\), n ∈ Z is the general solution.
![]()
Question 2.
Solve sin 2θ = \(\frac{\sqrt{5}-1}{4}\)
Solution:
sin 2θ = \(\frac{\sqrt{5}-1}{4}\) = sin 18° = sin(\(\frac{\pi}{10}\)) and \(\frac{\pi}{10}\) ∈ [-\(\frac{\pi}{2}\), \(\frac{\pi}{2}\)]
⇒ 2θ = \(\frac{\pi}{10}\) and hence θ = \(\frac{\pi}{20}\) is the principal solution.
General solution is given by
2θ = nπ + (-1)n \(\frac{\pi}{10}\), n ∈ Z (or)
θ = n\(\frac{\pi}{2}\) + (-1)n \(\frac{\pi}{20}\), n ∈ Z
Question 3.
Solve tan2θ = 3
Solution:
tan2θ = 3 = tan θ = ±\(\sqrt{3}\) = tan (±\(\frac{\pi}{3}\))
and ± \(\frac{\pi}{3}\) ∈ (-\(\frac{\pi}{2}\), \(\frac{\pi}{2}\))
∴ θ = ± \(\frac{\pi}{3}\) are the principal solutions of the given equation.
General solution is given by nπ ± \(\frac{\pi}{3}\), n ∈ Z
Question 4.
Solve 3 cosec x = 4 sin x
Solution:
3 cosec x = 4 sin x
⇔ \(\frac{3}{\sin x}\) = 4 sin x
⇒ sin2x = \(\frac{3}{4}\)
⇔ sin x = ± \(\frac{\sqrt{3}}{2}\)
∴ Principal solutions are x = ± \(\frac{\pi}{3}\)
General solutions are given by x = nπ ± \(\frac{\pi}{3}\), n ∈ Z
Question 5.
If x is acute and sin (x + 10°) = cos (3x – 68°), find x in degrees.
Solution:
Given sin (x + 10°) = cos (3x – 68°)
⇔ sin(x + 10°) = sin (90° + 3x – 68°) = sin (22° + 3x)
∴ x + 10° = n(180°) + (-1 )n (22° + 3x)
If n = 2k, k ∈ Z then
x + 10° = (2k) (180°) + (220 + 3x)
⇒ 2x = -k(360°) – 12°
⇒ x = \(\frac{-k\left(360^{\circ}\right)-12^{\circ}}{2}\)
= -k(180°) – 6°
This is not valid because for any integral k, x is not acute
If n = 2k + 1, then
x + 10° = (2k + 1) 180° – (22°+ 3x)
⇒ 4x = (2k + 1) 180° – 32°
⇒ x = (2k + 1) 45° – 8°
Now k = 0 ⇒ x = 37°
For other integral values of k, x is not acute
∴ The only solution is x = 37°
Question 6.
Solve cos 3θ = sin 2θ
Solution:
cos 3θ = sin 2θ = cas (\(\frac{\pi}{2}\) – 2θ)
⇒ 3θ = 2nπ ± (\(\frac{\pi}{2}\) – 2θ), n ∈ Z
⇒ 3θ = 2nπ + (\(\frac{\pi}{2}\) – 2θ) (or)
3θ = 2nπ – (\(\frac{\pi}{2}\) – 2θ)
⇒ 5θ = 2nπ + \(\frac{\pi}{2}\) (or) θ = 2nπ – \(\frac{\pi}{2}\)
⇒ θ = (4n + 1) \(\frac{\pi}{10}\), n ∈ Z (or)
θ = (4n – 1) \(\frac{\pi}{2}\), n ∈ Z
Question 7.
Solve 7 sin2θ + 3cos2θ = 4
Solution:
Given that
7 sin2θ + 3 cos2θ = 4
⇒ 7 sin2θ + 3(1 – sin2θ) = 4
⇒ 4sin2θ = 1
⇒ sin θ = ± \(\frac{1}{2}\)
∴ Principal solutions are θ = ± \(\frac{\pi}{6}\) and the general solution is given by
θ = nπ ± \(\frac{\pi}{6}\), n ∈ z.
Question 8.
Solve 2 cos2θ – \(\sqrt{3}\) sin θ + 1 = 0
Solution:
2 cos2θ – \(\sqrt{3}\) sin θ + 1 = 0
⇒ 2(1 – sin2θ) – \(\sqrt{3}\) sin θ + 1 = 0
⇒ 2 sin2θ + \(\sqrt{3}\) sin θ – 3 = 0
⇒ (2 sin θ – \(\sqrt{3}\))(sin θ + \(\sqrt{3}\)) = 0
⇒ sin θ = \(\frac{\sqrt{3}}{2}\) and sin θ ≠ \(\sqrt{3}\)
∴ sin θ = \(\frac{\sqrt{3}}{2}\) = sin (\(\frac{\pi}{3}\))
∴ θ = \(\frac{\pi}{3}\) is the principal solution and general solution is given by
θ = nπ + (-1)n \(\frac{\pi}{3}\), n ∈ Z.
Question 9.
Find all values of x ≠ 0 in (-π, π) satisfying the equation 81+cosx+cos2x+…… = 43.
Solution:
If cos x = ±1, the sum
1 + cos x + cos2 x + …………… ∞ does not converse.
Assume |cos x| < 1.
Then 1 + cos x + cos2 x + …………… ∞
= \(\frac{1}{1-\cos x}\)
(∵ a + ar + ar2 + ……………. ∞ = \(\frac{a}{1-r}\), if |r| < 1)
Now 81+cosx+cos2x+…………. = 43
⇒ (8)\(\frac{1}{1-\cos x}\) = 82
⇔ \(\frac{1}{1-\cos x}\) = 2 = cos x = \(\frac{1}{2}\)
⇔ x = \(\frac{\pi}{3}\) or –\(\frac{\pi}{3}\) (∵ x ∈ (-π, π))
Question 10.
Solve tan θ + 3 cot θ = 5 sec θ
Solution:
tan θ + 3 cot θ = 5 sec θ is valid only when
cos θ ≠ 0 and sin θ ≠ 0
⇒ \(\frac{\sin \theta}{\cos \theta}\) + 3\(\frac{\cos \theta}{\sin \theta}\) =\(\frac{5}{\cos \theta}\)
⇒ sin2θ+ 3cos2θ= 5 sin θ
⇒ sin2θ + 3(1 – sin2θ) – 5 sin θ = 0
⇒ 2 sin2θ + 5 sin θ – 3 = 0
⇒ 2 sin2θ + 6sin θ – sin θ – 3 = 0
⇒ 2 sin θ(sin θ + 3) – 1 (sin θ + 3)= 0
⇒ (2 sin θ – 1)(sin θ + 3) = 0
⇒ sin θ = \(\frac{1}{2}\) (∵sin θ ≠ -3)
∴ sin = \(\frac{1}{2}\) = sin (\(\frac{\pi}{6}\))
∴ Principal solution is θ = \(\frac{\pi}{6}\) and general solution is nπ + (-1)n\(\frac{\pi}{6}\), n ∈ Z.
Question 11.
Solve 1 + sin 2θ = 3 sin θ cos θ. [Mar 11]
Solution:
1 + sin2θ = 3 sin θ cas θ
Dividing by cos2θ, ∵ cos θ ≠ 0
sec2θ + tan2θ = 3 tan θ
⇒ (1 + tan2θ) + tan2θ – 3 tan θ = 0
⇒ 2tan2θ – 3 tanθ + 1 = 0
⇒ 2tan2θ – 2 tan θ – tan θ + 1 = 0
⇒ 2 tan θ (tan θ – 1) – (tan θ – 1) = 0
⇒ (tan θ – 1)(2 tan θ – 1) = 0
∴ tan θ = 1 (or) tan θ = \(\frac{1}{2}\)
Now tan θ = 1 = tan \(\frac{\pi}{4}\)
∴ Principal solution θ = \(\frac{\pi}{4}\) and general solution is given by
θ = nπ + \(\frac{\pi}{6}\), n ∈ Z
let α be the principal solution of tan θ = \(\frac{1}{2}\)
Then the general solution is given by θ = nπ + α, n ∈ Z
Question 12.
Solve \(\sqrt{2}\) (sin x + cos x) = \(\sqrt{3}\) (A.P) [Mar. 16, 15, May 12, 08]
Solution:
Given that \(\sqrt{2}\) (sin x + cos x) = \(\sqrt{3}\)
⇔ sin x + cos x = \(\sqrt{\frac{3}{2}}\)
By dividing both sides by \(\sqrt{2}\), we get
\(\frac{1}{\sqrt{2}}\) sin x + \(\frac{1}{\sqrt{2}}\) cos x = \(\sqrt{\frac{3}{2}}\)
⇒ sin x. sin \(\frac{\pi}{4}\) + cos x. cos \(\frac{\pi}{4}\) = \(\sqrt{\frac{3}{2}}\)
⇒ cos (x – \(\frac{\pi}{4}\)) = \(\sqrt{\frac{3}{2}}\) = cos (\(\frac{\pi}{6}\))
Principal solution is x – \(\frac{\pi}{4}\) = \(\frac{\pi}{6}\) (i.e.,) x = \(\frac{5 \pi}{12}\)
General solution is given by
x – \(\frac{\pi}{4}\) = 2nπ ± \(\frac{\pi}{6}\), n ∈ Z
⇒ x = 2nπ + latex]\frac{5 \pi}{12}[/latex] (or) x = 2nπ + \(\frac{\pi}{12}\), n ∈ Z
Question 13.
Find general solution of θ which satisfies both the equations sinθ = –\(\frac{1}{2}\) and cosθ = –\(\frac{\sqrt{3}}{2}\)
Solution:
Given sin θ = –\(\frac{1}{2}\) = – sin \(\frac{\pi}{6}\)
= sin (π + \(\frac{\pi}{6}\)) = sin (2π – \(\frac{\pi}{6}\))
= sin \(\frac{7\pi}{6}\) = sin \(\frac{11\pi}{6}\)
∴ Considering only angles in (0, 2π), the only values of θ satisfying sin θ = –\(\frac{1}{2}\) are \(\frac{7\pi}{6}\) or \(\frac{11\pi}{6}\)
cos θ = –\(\frac{\sqrt{3}}{2}\) = – cos\(\frac{\pi}{6}\)
= cos (π – \(\frac{\pi}{6}\)) or cos (π + \(\frac{\pi}{6}\))
= cos \(\frac{5\pi}{6}\) or cos \(\frac{7\pi}{6}\).
∴ Considering only angles in (0, 2π), the only values of θ satisfying cos θ = –\(\frac{\sqrt{3}}{2}\) are \(\frac{5 \pi}{6}\) or \(\frac{7 \pi}{6}\).
Thus \(\frac{7 \pi}{6}\) is the only angle which satisfies both the equations.
Hence general solution for θ is
θ = 2nπ + \(\frac{7 \pi}{6}\), n ∈ Z.
Question 14.
If θ1, θ2 are solutions of the equation a cos 2θ + b sin 2θ = c, tan θ1 ≠ tan θ2 and a + c ≠ 0. find the values of
(i) tan θ1 + tan θ2
(ii) tan θ1 . tanθ2 (T.S.) [Mar 16, 15]
Solution:
a cos 2θ + b sin 2θ = c
⇔ a(\(\frac{1-\tan ^{2} \theta}{1+\tan ^{2} \theta}\)) + b(\(\frac{2 \tan \theta}{1+\tan ^{2} \theta}\)) = c
⇔ a(1 – tan2θ) + 2b tan θ) = c(1 + tan2θ)
(c + a) tan2θ – 2b tan θ + (c – a) = 0
This is a quadratic equation in tan θ.
It has two roots tan θ1 and tan θ2. since θ1 and θ2 are solutions for the given equation
Sum of the roots = tan θ1 + tan θ2 = \(\frac{2 b}{a+c}\)
Product of the roots = tan θ1 tan θ2 = \(\frac{c-a}{c+a}\)
Now tan(θ1 + θ2) = \(\frac{\tan \theta_{1}+\tan \theta_{2}}{1-\tan \theta_{1} \tan \theta_{2}}\)
= \(\frac{\left(\frac{2 b}{a+c}\right)}{1-\left(\frac{c-a}{c+a}\right)}\) = \(\frac{2 \mathrm{~b}}{2 \mathrm{a}}\) = \(\frac{b}{a}\)
Question 15.
Solve 4 sin x sin 2x sin 4x = sin 3x
Solution:
sin 3x = 4 sin x sin 2x sin 4x
= 2 sin x (2 sin 4x sin 2x)
= 2 sin x [cos (2x) – cos 6x]
⇔ sin 3x = 2 cos 2xsinx – 2 cos6x sin x
⇔ sin 3x = sin (3x) – sin x – 2 cos 6x sin x
⇒ 2 cos 6x sin x + sin x = 0
⇒ sin x(2 cos 6x + 1) = 0
⇒ sin x = 0 (or) cos 6x = \(\frac{-1}{2}\)
Case (i) : sin x = 0
⇒ x = 0 is the principal solution and x = nπ, n ∈ Z is the general solution.
Case (ii) : cos 6x = \(\frac{-1}{2}\) = cos (\(\frac{2 \pi}{3}\))
⇒ 6x = \(\frac{2 \pi}{3}\) ⇒ x = \(\frac{\pi}{9}\) is the principal solution
The general solution is given by
6x = 2nπ ± \(\frac{2 \pi}{3}\), n ∈ Z
⇒ x = \(\frac{n \pi}{3}\) ± \(\frac{\pi}{9}\), n ∈ Z
Question 16.
If 0 < θ < π, solve cos θ.cos 2θ cos 3θ = \(\frac{1}{4}\).
Solution:
4 cos θ cos 2θ cos 3θ = 1
⇒ 2 cos 2θ (2 cos 3θ. cos θ) = 1
⇒ 2 cos 2θ (cos 4θ + cos 2θ) = 1
⇒ 2 cos 4θ cos 2θ + (2 cos2 2θ – 1) = 0
⇒ 2 cos 4θ cos 2θ + cos 4θ = 0
⇒ cos 4θ (2 cos 2θ + 1) = 0
⇒ cos 4θ = 0 (or) cos 2θ = \(\frac{-1}{2}\)
Case(i): cos 4θ = 0 = cos (\(\frac{\pi}{2}\))
⇒ 4θ = \(\frac{\pi}{2}\) is the principal solution (or)
θ = \(\frac{\pi}{8}\).
The general solution is given by
4θ = (2n + 1) \(\frac{\pi}{2}\), n ∈ Z
⇒ θ = (2n + 1) \(\frac{\pi}{8}\), n ∈ Z
Put n = 0, 1, 2, ……… we get
\(\left\{\frac{\pi}{8}, \frac{3 \pi}{8}, \frac{5 \pi}{8}, \frac{7 \pi}{8}\right\}\) are the solutions that lie in (0, π)
Case (ii) : cos 2θ = \(\frac{-1}{2}\) = cos (\(\frac{2\pi}{3}\))
⇒ 2θ = \(\frac{2\pi}{3}\) is the principal solution
(i.e.,) θ = \(\frac{\pi}{3}\)
The general solution is given by
2θ = 2nπ ± \(\frac{2\pi}{3}\), n ∈ Z
⇒ θ = nπ ± latex]\frac{\pi}{3}[/latex], n ∈ Z
Put n = 0, 1, we get \(\left\{\frac{\pi}{3}, \frac{2 \pi}{3}\right\}\) are the solutions that lie in the interval (0, π).
Hence the solutions of the given equation in (0, π) are \(\left\{\frac{\pi}{8}, \frac{\pi}{3}, \frac{3 \pi}{8}, \frac{5 \pi}{8}, \frac{2 \pi}{3}, \frac{7 \pi}{8}\right\}\).
Question 17.
Given p ≠ ± q, show that the solutions of cos pθ + cos qθ = 0 form two series each of which is in A.R Find also the common difference of each A.P.
Solution:
cos pθ + cos qθ = 0
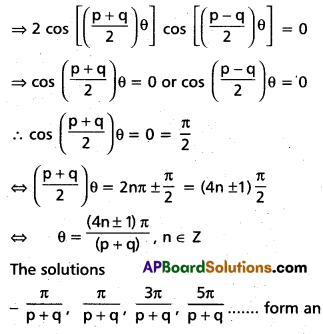
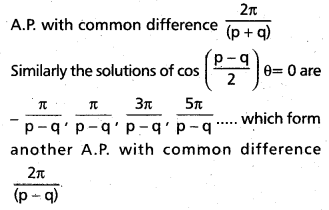
Question 18.
Solve sin 2x – cos 2x = sin x – cos x.
Solution:
sin 2x – cos 2x = sin x – cos x.
⇒ sin 2x – sin x = cos 2x – cos x
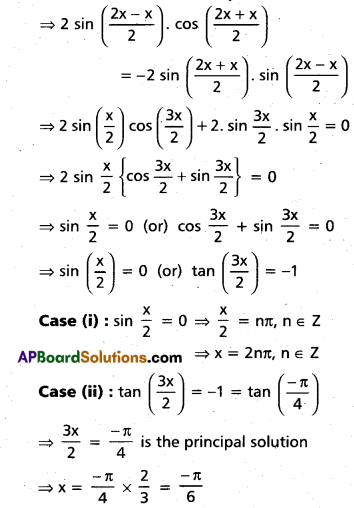
The general solution is given by
\(\frac{3 x}{2}\) = nπ + (\(\frac{-\pi}{4}\)), n ∈ Z
⇒ x = \(\frac{2 n \pi}{3}\) – \(\frac{\pi}{6}\), n ∈ Z
∴ Solution set for the given equation is {2nπ / n ∈ Z} ∪ {\(\frac{2 n \pi}{3}\) – \(\frac{\pi}{6}\) / n ∈ Z}