Students get through AP Inter 2nd Year Physics Important Questions 12th Lesson Dual Nature of Radiation and Matter which are most likely to be asked in the exam.
AP Inter 2nd Year Physics Important Questions 12th Lesson Dual Nature of Radiation and Matter
Very Short Answer Questions
Question 1.
What are “cathode rays”? [A.P. Mar. 17]
Answer:
Cathode rays are streams of fast-moving electrons or negatively charged particles.
Question 2.
What important fact did Millikan’s experiment establish?
Answer:
Millikan’s experiment established that electric charge is quantized. That means the charge on anybody (oil drop) is always an integral multiple of the charge of an electron, i.e., Q = ne.
![]()
Question 3.
What is “work function”? [T.S. Mar. 17, 15]
Answer:
The minimum energy required to liberate an electron from a photo metal surface is called the work function, Φ0.
Question 4.
What is “photoelectric effect” ?
Answer:
When light of sufficierit energy is incident on the photometal surface, electrons are emitted. This phenomenon is called photoelectric effect.
Question 5.
Give examples of “photosensitive substances”. Why are they called so ?
Answer:
Examples of photosensitive substances are Li, Na, K, Rb and Cs etc.
The work function of alkali metals is very low. Even the ordinary visible light, alkali metals can produce photoelectric emission. Hence they are called photosensitive substances.
Question 6.
Write down Einstein’s photoelectric equation. [A.P. Mar. 15]
Answer:
Einstein’s photoelectric equation is given by Kmax = \(\frac{1}{2}\) mv2max = hυ – Φ0
![]()
Question 7.
Write down de-Broglie’s relation and explain the terms therein. [T.S. & A.P. Mar. 16]
Answer:
The de-Broglie wave length (λ) associated with a moving particle is related to its momentum (p) is λ = \(\frac{h}{p}=\frac{h}{m v}\), where h is planck’s constant.
Question 8.
State Heisenberg’s Uncertainity Principle. [Mar. 14]
Answer:
Uncertainity principle states that “it is impossible to measure both position (∆x) and momentum of an electron (∆p) [or any other particle] at the same time exactly”, i.e., ∆x . ∆p ≈ h where ∆x is uncertainty in the specification of position and ∆p is uncertainty in the specification of momentum.
Question 9.
The photoelectric cut off voltage in a certain experiment is 1.5 V. What is the maximum kinetic energy of photoelectrons emitted ? [Mar. 11]
Answer:
Cut off voltage, V0 = 1.5 V; Maximum kinetic energy, (KE)max = eV0 = e × 1.5 = 1.5eV
Question 10.
An electron, an α-particle and a proton have the same kinetic energy. Which of these particles has the shortest de Broglie wavelength ? [T.S. Mar. 15]
Answer:
For a particle,
de Broglie wavelength, λ = h/p
Kinetic energy, K = p2/2m
Then, λ = h/\(\sqrt{2 \mathrm{mK}}\)
For the same kinetic energy K, the de Broglie wavelength associated with the particle is inversely proportional to the square root of their masses.A proton ![]() is 1836 times massive than an electron and an a-particle \(\left({ }_2^4 \mathrm{He}\right)\) four times that of a proton.
is 1836 times massive than an electron and an a-particle \(\left({ }_2^4 \mathrm{He}\right)\) four times that of a proton.
Hence, α-particle has the shortest de Broglie wavelength.
![]()
Qeustion 11.
What is-the de Broglie wavelength associated with an electron, accelerated through a potential difference of 100 volts ? [A.P. Mar. 15]
Solution:
Accelerating potential V = 100 V The de Broglie wavelngth λ is
λ = h/p = \(\frac{1.227}{\sqrt{\mathrm{V}}}\) nm
λ = \(\frac{1.227}{\sqrt{100}}\) nm = 0.123 nm
The de Broglie wavelength associated with an electron in this case is of the order of X-ray wavelengths.
Question 12.
If the wave length of electro magnetic radiation is doubled, what happens to energy of photon ? [IPE 2015 (TS)]
Answer:
E = \(\frac{\mathrm{hc}}{\lambda}\) ⇒ E ∝ \(\frac{1}{\lambda}\), since wave length of photon is doubled, its energy becomes halved.
Question 13.
In an experiment on photoelectric effect, the slope of the cut-off voltage versus frequency of incident light is found to be 4.12 × 10-15 V s. Calculate the value of Planck’s constant.
Solution:
Given, slope of graph tan θ = 4.12 × 10-15 V – s;
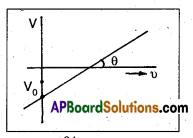
For slope of graph, tan θ = \(\frac{\mathrm{V}}{\mathrm{v}}\)
We know that hv = eV
\(\frac{\mathrm{V}}{\mathrm{v}}=\frac{\mathrm{h}}{\mathrm{e}} \Rightarrow \frac{\mathrm{h}}{\mathrm{e}}\) = 4.12 × 10-15; h = 4.12 × 10-15 × 1.6 × 10-19 = 6.592 × 10-34 J – s.
Question 14.
The energy flux of sunlight reaching the surface of the earth is 1.388 × 103 W/m2. How many photons (nearly) per square metre are incident on the Earth per second ? Assume that the photons in the sunlight have an average wavelength of 550 nm.
Solution:
Given, P = 1.388 × 103 W/m2; λ = 550 nm = 550 × 10-9 m ,
h = 6.63 × 10-34 J-s; c = 3 × 108 m/s
Energy of each photon E = \(\frac{\text { hc }}{\lambda}=\frac{6.63 \times 10^{-34} \times 3 \times 10^8}{550 \times 10^{-9}}\) = 3.616 × 10-19 J
No. of photons incident on the earth’s surface, N = \(\frac{\mathrm{P}}{\mathrm{E}}=\frac{1.388 \times 10^3}{3.66 \times 10^{-19}}\)
∴ N = 3.838 × 1021 photons/m2 – s.
![]()
Question 15.
Show that the wavelength of electromagnetic radiation is equal to the de-Brogile wavelength of its quantum (photon). [Mar. 14]
Answer:
Wave length of electromagnetic wave of frequency v and velocity C is given by,
λ = \(\frac{\mathrm{C}}{\mathrm{v}} \Rightarrow \lambda=\frac{\mathrm{C}}{\mathrm{v}} \times \frac{\mathrm{h}}{\mathrm{h}}=\frac{\mathrm{h}}{\left(\frac{\mathrm{hv}}{\mathrm{C}}\right)}=\frac{\mathrm{h}}{\mathrm{p}}\) (∵ \(\frac{\mathrm{hv}}{\mathrm{C}}\) = p)
Hence, we can say wavelength of electromagnetic radiation is equal to the de-Brogile wavelength.
Sample Problem :
Question 1.
Calculate the (a) momentum and (b) dE-Brogile wavelength of the electrons accelerated through a potential difference of 56 V. [Mar. 14]
Answer:
a) Mass of the electron, m = 9 × 10-31 kg;
Potential difference, V = 56V
Momentum of electron, mv = \(\sqrt{2 \mathrm{eVm}}=\sqrt{2 \times\left(1.6 \times 10^{-19}\right) \times 56 \times 9 \times 10^{-31}}\) = 4.02 × 10-24kg ms-1
b) de-Broglie wavelength, λ = \(\frac{\mathrm{h}}{\mathrm{p}}=\frac{\mathrm{h}}{\mathrm{mv}}=\frac{6.62 \times 10^{-34}}{4.04 \times 10^{-24}}\) = 1.64 × 10-10m
Short Answer Questions
Question 1.
Describe an experiment to study the effect of frequency of incident radiation on ‘stopping potential’.
Answer:
Experimental study of the effect of frequency of incident radiation on stopping potential:
- The experimental set up is shown in fig.
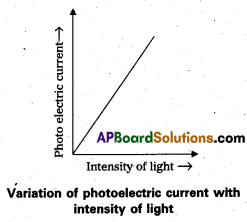
- Monochromatic light of sufficient energy (E = hv) from source ‘s’ is incident on photosensitive plate ‘C’ (emitter), electrons are emitted by it.
- The electrons are collected by the plate A (collector), by the electric field created by the battery.
- The polarity of the plates C and A can be reversed by a commutator.
- For a particular frequency of incident radiation, the minimum negative (retarding) potential V0 given to the plate A for which the photo current stops or becomes zero is called stopping potential.
- The experiment is repeated with different frequencies, and their different stopping potential are measured with voltmeter.
- From graph, we note that
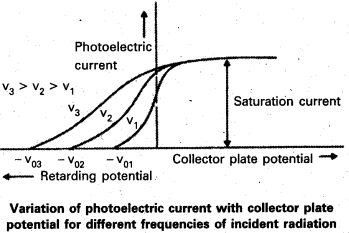
- The values of stopping potentials are different for different frequencies.
- The value of stopping potential is more negative for radiation of highef incident frequency.
- The value of saturation current depends on the intensity of incident radiation but it is independent of the frequency of the incident radiation.
Question 2.
What is the deBroglie wavelength of a ball of mass 0.12 Kg moving with a speed of 20 ms-1? What can we infer from this result ?
Answer:
Given, m = 0.12 kg; υ = 20 m/s; h = 6.63 × 10-34 J-s;
λ = \(\frac{\mathrm{h}}{\mathrm{mv}}=\frac{6.63 \times 10^{-34}}{0.12 \times 20}=\frac{6.63 \times 10^{-34}}{2.4}\)
∴ λ = 2.762 × 10-34 m = 2762 × 10-21 Å.
The wave length of ball is very very small. Hence, its motion can be observed.
![]()
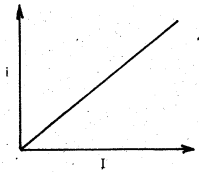
Question 3.
What is the effect of (i) intensity of light (ii) potential on photoelectric current ?
Answer:
(i) Effect of intensity of light on photoelectric current:
1) When the intensity (I) of incident light, with frequency greater than the threshold frequency (υ > υ0) is increased then the number of photoelectrons emitted increases i.e., the value of photoelectric current (i) increases, ie., i ∝ I.
ii) The effect of potential on photoelectric current:
- On increasing the positive potential on collecting electrode, the photoelectric current increases. At a particular positive potential, the photocurrent becomes maximum which is known as saturated current.
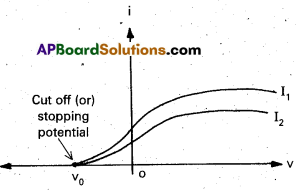
- On increasing the value of negative potential on collecting electrode, the photoelectric current gradually goes on decreasing. At a particular negative potential the value of photoelectric current becomes zero. This is known as stopping potential.
- Stopping potential does not depend on the intensity of incident light. On increasing intensity, the value of saturated current increases, whereas the stopping potential remains unchanged.
Sample Problem :
Question 1.
The work function of caesium metal is 2.14 eV When light of frequency 6 × 1014 Hz is incident on the metal surface, photoemission of electrons occurs. What is the (a) maximum kinetic energy of the emitted electrons, (b) stopping potential and (c) maximum speed of the emitted photoelectrons ? [A.P. Mar. 16]
Solution:
Given, Φ0 = 2.14 eV; v = 6 × 1014 Hz
a) KEmax = hv – Φ0 = \(\frac{6.63 \times 10^{-34} \times 6 \times 10^{14}}{1.6 \times 10^{-19}}\) – 2.14
∴ KEmax = 0.35 eV
b) KEmax = eV0 ⇒ 0.35 eV = eV0
∴ V0 = 0.35 V
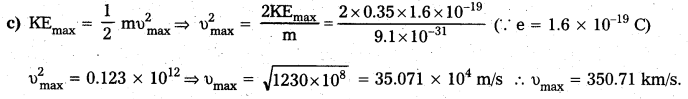
Long Answer Questions
Question 1.
How did Einstein’s photoelectric equation explain the effect of intensity and potential on photoelectric current ? How did this equation account for the effect of frequency of ‘ incident light on stopping potential ?
Answer:
- Einstein postulated that a beam of light consists of small energy packets called photons or quanta.
- The energy of photon is E = hv. Where ‘h1 is Planck’s constant; v is frequency of incident light (or radiation).
- If the absorbed energy of photon is greater than the work function (Φ0 = hυ0), the electron is emitted with maximum kinetic energy i.e., kmax = \(\frac{1}{2} \mathrm{mv}_{\max }^2\) = eV0 = hv – Φ0. This equation is known as Einstein’s photoelectric equation.
- Effect of intensity of light on photoelectric current:
When the intensity (I) of incident light, with frequency greater than the threshold frequency (υ > υ0) is increased then the number of photoelectrons emitted decreases i.e., the value of photoelectric current (i) increases, ie., i ∝ I.

- The effect of potential on photoelectric current:
- On increasing the positive potential on collecting electrode, the photoelectric current increases. At a particular positive potential, the photocurrent becomes maximum which is known as saturated current.

- On increasing the value of negative potential on collecting electrode, the photoelectric current gradually goes on decreasing. At a particular negative potential the value of photoelectric current becomes zero. This is known as stopping potential (v0).
- Stopping potential does not depend on the intensity of incident light. On increasing intensity, the value of saturated current increases, whereas the stopping potential remains unchanged.
- On increasing the positive potential on collecting electrode, the photoelectric current increases. At a particular positive potential, the photocurrent becomes maximum which is known as saturated current.
- The effect of frequency of incident radiation on stopping potential:
On increasing the frequency of incident light, the value of stopping potential goes on increasing gradually as shown in fig. That means kmax increases eV0 also increases.
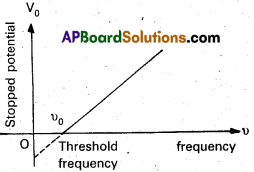
- From the graph, we note that
- For a given photosensitive metal, the cut off potential (v0) varies linearly with the frequency of the incident radiation.
- For a given photosensitive metal, there is a certain minimum cut off frequency υ0 (called threshold frequency) for which the stopping potential is zero.
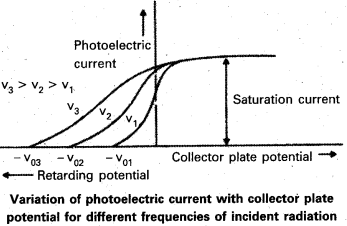
- From the graph we note that
- The value of cut-off potential is different for radiation of different frequency.
- The value of stopping potential is more negative for radiation of higher incident frequency.
- From above experiments, it is found that, if the incident radiation is of higher frequency than that of threshold frequency, the photoelectric emission is. possible.
Textual Examples
Question 1.
Monochromatic light of frequency 6.0 × 1014 Hz is produced by a laser. The power emitted is 2.0 × 10-3 W. (a) What is the energy of a photon in the light beam ? (b) How many photons per second, on an average, are emitted by the source ?
Solution:
a) Each photon has an energy
E = hv = (6.63 × 10-34 J s) (6.0 × 1014 Hz)
= 3.98 × 10-19 J
b) If N is the number of photons emitted by the source per second, the power P transmitted in the beam equals N times the energy per photon E, so that P = NE.
Then N = \(\frac{\mathrm{P}}{\mathrm{E}}=\frac{2.0 \times 10^{-3} \mathrm{~W}}{3.98 \times 10^{-19} \mathrm{~J}}\)
= 5.0 × 1015 photons per second.
![]()
Question 2.
The work function of caesium is 2.14 eV. Find (a) the threshold frequency for caesium, and (b) the wavelength of the incident light if the photocurrent is brought to zero by a stopping potential of 0.60 V. [A.P. Mar. 16]
Solution:
a) For the cut-off or threshold frequency, the energy hv0 of the incident radiation must be equal to work function Φ0, so that
v0 = \(\frac{\phi_0}{\mathrm{~h}}=\frac{2.14 \mathrm{eV}}{6.63 \times 10^{-34} \mathrm{Js}}\)
= \(\frac{2.14 \times 1.6 \times 10^{-19} \mathrm{~J}}{6.63 \times 10^{-34} \mathrm{~J} \mathrm{~s}}\) = 5.16 × 1014 Hz
Thus, for frequencies less than this threshold frequency, no photoelectrons are ejected.
b) Photocurrent reduces to zero, when maximum kinetic energy of the emitted photoelectrons equals the potential energy e V0 by the retarding potential V0. Einstein’s Photoelectric equation is
eV0 = hv – Φ0 = \(\frac{\mathrm{hc}}{\lambda}\) – Φ0
or λ = hc/(eV0 + Φ0)
= \(\frac{\left(6.63 \times 10^{-34} \mathrm{~J} \mathrm{~s}\right) \times\left(3 \times 10^8 \mathrm{~m} / \mathrm{s}\right)}{(0.60 \mathrm{eV}+2.14 \mathrm{eV})}\)
= \(\frac{19.89 \times 10^{-26} \mathrm{~J} \mathrm{~m}}{(2.74 \mathrm{eV})}\)
λ = \(\frac{19.89 \times 10^{-26} \mathrm{~J} \mathrm{~m}}{2.74 \times 1.6 \times 10^{-19} \mathrm{~J}}\) = 454 nm
Question 3.
The wavelength of light in the visible region is about 390 nm for violet colour, about 550 nm (average wavelength) for yellow-green colour and about 760 nm for red colour.
(a) What are the energies of photons in (eV) at the (i) violet end, (ii) average wavelength, yellow-green colour, and (iii) red end of the visible spectrum ? (Take h = 6.63 × 10-34 J s and 1 eV = 1.6 × 10-19 J.)
(b) From which of the photosensitive materials with work functions listed in table and using the results of (i), (ii) and (iii) of (a) can you build a photoelectric device that operates with visible light ?
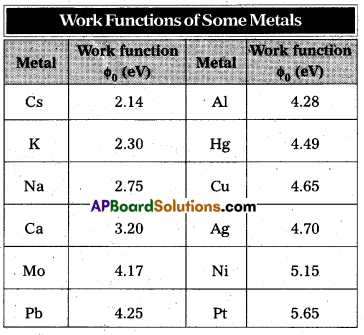
Solution:
a) Energy of the incident photon,
E = hv = hc/λ
E = (6.63 × 10-34 J s) (3 × 108 m/s)/λ.
= \(\frac{1.989 \times 10^{-25} \mathrm{~J} \mathrm{~m}}{\lambda}\)
i) For violet light,
λ1 = 390 nm (lower wavelength end)
Incident photon energy,
E1 = \(\frac{1.989 \times 10^{-25} \mathrm{~J} \mathrm{~m}}{390 \times 10^{-9} \mathrm{~m}}\)
5.10 × 10-19 J = \(\frac{5.10 \times 10^{-19} \mathrm{~J}}{1.6 \times 10^{-19} \mathrm{~J} / \mathrm{eV}}\)
= 3.19 eV
ii) For yellow-green light,
λ2 = 550 nm (average wavelength)
Incident photon energy,
E2 = \(\frac{1.989 \times 10^{-25} \mathrm{~J} \mathrm{~m}}{550 \times 10^{-9} \mathrm{~m}}\)
= 3.62 × 10-19 J = 2.26 eV.
iii) For red light,
λ3 = 760 nm (higher wavelength end)
Incident photon energy,
E3 = \(\frac{1.989 \times 10^{-25} \mathrm{~J} \mathrm{~m}}{760 \times 10^{-9} \mathrm{~m}}\)
= 2.62 × 10-19 J = 1.64 eV
![]()
b) For a photoelectric device to operate, we require incident light energy E to be equal to or greater than the work function Φ0 of the material. Thus, the photoelectric device will operate with violet light (with E = 3.19 eV) photosensitive material Na (with Φ0 = 2.75 eV), K (with Φ0 = 2.30 eV) and Cs (with Φ0 = 2.14 eV). It will also operate with yellow-green light (with E = 2.26 eV) for Cs (with Φ0 = 2.14 eV) only. However, it will not operate with red light (with E = 1.64 eV) for any of these photosensitive materials.
Question 4.
What is the de Broglie wavelength associated with (a) an electron moving with a speed of 5.4 × 106 m/s, and (b) a ball of mass 150 g travelling at 30.0 m/s ?
Solution:
a) For the electron :
Mass m= 9.11 × 10-31 kg, speed υ = 5.4 × 106 m/s. Then, momentum
P = mυ = 9.11 × 10-31 (kg) × 5.4 × 106 (m/s)
P = 4.92 × 10-24 kg m/s
de Broglie wavelength, λ = h/p
= \(\frac{6.63 \times 10^{-34} \mathrm{~J} \mathrm{~s}}{4.92 \times 10^{-24} \mathrm{~kg} \mathrm{~m} / \mathrm{s}}\)
λ = 0.135 nm
b) For the ball:
Mass m’ = 0.150 kg, speed υ’ = 30.0 m/s.
Then momentum
p’ = m’υ’ = 0.150 (kg) × 30.0 (m/s)
p’ = 4.50 kg m/s
de Broglie wavelength λ’ = h/p’.
= \(\frac{6.63 \times 10^{\pm 34} \mathrm{Js}}{4.50 \times \mathrm{kg} \mathrm{m} / \mathrm{s}}\)
λ’ = 1.47 × 10-34 m
The de Broglie wavelength of electron is comparable with X-ray wavelengths. However, for the ball it is about 10-19 times the size of the proton, quite beyond experimental measurement.
Question 5.
An electron, an a-particle and a proton have the same kinetic energy. Which of these particles has the shortest de Broglie wavelength ? [T.S. Mar. 15]
Solution:
For a particle,
de Broglie wavelength, λ = h/p
Kinetic energy, K = p2/2m
Then, λ = h /\(\sqrt{2 \mathrm{mK}}\)
For the same kinetic energy K, the de Broglie wavelength associated with the particle is inversely proportional to the square root of their masses. A proton \(\left({ }_1^1 \mathrm{He}\right)\) is 1836 times massive than an electron and an a-particle \(\left({ }_2^4 \mathrm{He}\right)\) four times that of a proton
Hence, α-particle has the shortest de Broglie wavelength.
![]()
Question 6.
A particle is moving three times as fast as an electron. The ratio of the de Broglie wavelength of the particle to that of the electron is 1.813 × 10-4. Calculate the particle’s mass and identify the particle.
Solution:
de Broglie wavelength of a moving particle, having mass m and velocity υ :
λ = \(\frac{\mathrm{h}}{\mathrm{p}}=\frac{\mathrm{h}}{\mathrm{mv}}\)
Mass, m = h/A.
For an electron, mass me = h/λe υe
Now, we have υ/υe = 3 and
λ/λe = 1.813 × 10-4
Then, mass of the particle,
m = me \(\left(\frac{\lambda_{\mathrm{e}}}{\lambda}\right)\left(\frac{v_{\mathrm{e}}}{v}\right)\)
m = (9.11 × 10-31 kg) × (1/3) × (1/1.813 × 10-4)
m = 1.675 × 10-27 kg.
Thus, the particle, with this mass could be a proton or a neutron.
Question 7.
What is the de Broglie wavelength associated with an electron, accelerated through a potential difference of 100 volts? [A.P. Mar. 15]
Solution:
Accelerating potential V = 100 V The de Broglie wavelength λ is
λ = h/p = \(\frac{1.227}{\sqrt{\mathrm{V}}}\) nm
λ = \(\frac{1.227}{\sqrt{100}}\) nm = 0.123 nm
The de Broglie wavelength associated with an electron in this case is of the order of X-ray wavelengths.