Inter 1st Year Maths 1B Differentiation Solutions Ex 9(c)

Practicing the Intermediate 1st Year Maths 1B Textbook Solutions Inter 1st Year Maths 1B Differentiation Solutions Exercise 9(c) will help students to clear their doubts quickly.

Intermediate 1st Year Maths 1B Differentiation Solutions Exercise 9(c)

I.

Question 1.
Find the derivatives of the following functions.
i) sin^-1 (3x – 4x³)
Solution:
Put x = sin θ
y = sin^-1 (3 sin θ – 4 sin³ θ)
= sin^-1 (sin 3 θ) = 3 θ = 3 sin^-1 x.
\(\frac{dy}{dx}\) = \(\frac{3}{\sqrt{1-x^{2}}}\)

ii) cos^-1 (4X3 – 3x)
Solution:
Put x = cos θ
y = cos^-1 (4 cos³ θ – 3 cos θ)
= cos^-1 (cos 3θ) = 3θ = 3 cos^-1 x
\(\frac{dy}{dx}\) = \(\frac{3}{\sqrt{1-x^{2}}}\)

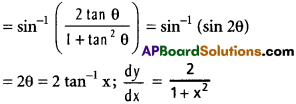
iii) sin^-1 \(\frac{3}{{1-x^{2}}}\)
Solution:
Put x tan θ ⇒ y

iv) tan^-1 \(\frac{a-x}{1+ax}\)
Solution:
Put a tan α, x = tan θ
y = tan^-1 \(\left(\frac{\tan \alpha-\tan \theta}{1+\tan \alpha \tan \theta}\right)\)
= tan^-1 (tan (α – θ)) = α – θ
= tan^-1 a – tan^-1 x;
\(\frac{dy}{dx}\) = 0 – \(\frac{1}{1+x^{2}}\) = – \(\frac{1}{1+x^{2}}\)

v) tan^-1 \(\sqrt{\frac{1-\cos x}{1+\cos x}}\)
Solution:

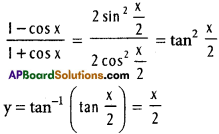
Differentiating w.r.to x; \(\frac{dy}{dx}\) = \(\frac{1}{2}\)

vi) sin [cos (x²)]
Solution:
\(\frac{dy}{dx}\) = cos [cos (x²)]\(\frac{d}{dx}\) [cos (x²)]
= cos [cos (x²)]. [-sin (x²)] \(\frac{d}{dx}\) (x²)
= cos [cos (x²)] [- sin (x²)]. 2x
= -2x . sin (x²).cos [cos (x²)]

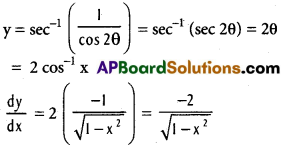
vii) sec^-1 (\(\frac{1}{2x^{2}-1}\)) (0 < x < \(\frac{1}{\sqrt{2}}\))
Solution:
x = cos θ
2x² – 1 = 2 cos² θ – 1 = cos 2θ

viii) sin^-1 [tan^-1 (e^-x)]
Solution:
\(\frac{dy}{dx}\) = cos [tan^-1 (e^-x)]. [tan^-1 (e^-x)]¹
= cos (tan^-1 (e^-x)]x – \(\frac{1}{1+\left(e^{-x}\right)^{2}}\) (e^-x)¹
= \(\frac{-e^{-x}}{1+e^{-2 x}}\) . cos [tan^-1 (e^-x)]

Question 2.
Differentiate f(x) with respect to g(x) for the following.
i) f(x) = e^x, g(x) = √x
Solution:
Let y = e^x and z = √x

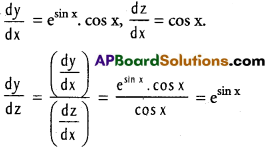
ii) f(x) = e^{sin x}, g(x) = sin x.
Solution:
Let y = e^{sin x} and z = sin x.

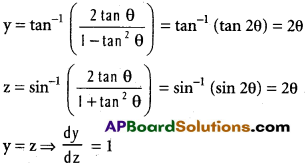
iii) f(x) = tan^-1 \(\frac{2x}{1-x^{2}}\), g(x) sin^-1 = \(\frac{2x}{1+x^{2}}\)
Solution:
Lety = tan^-1 \(\frac{2x}{1-x^{2}}\), and z = sin^-1 = \(\frac{2x}{1+x^{2}}\)
Put x = tan θ

Question 3.
If y = e^a sin^-1x the prove that \(\frac{dy}{dx}\) = \(\frac{a y}{\sqrt{1-x^{2}}}\)
Solution:
y = e^{a sin-1x}
\(\frac{dy}{dx}\) = e^{a sin-1x} (a sin^-1 x)¹
= e^{a sin-1x} . a \(\frac{1}{\sqrt{1-x^{2}}}\) = \(\frac{a y}{\sqrt{1-x^{2}}}\)

II.

Question 1.
Find the derivatives of the following function.
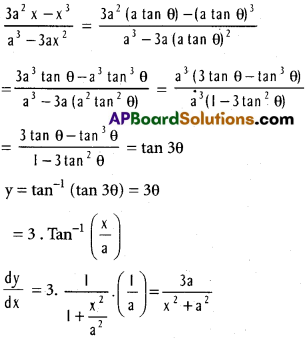
i) tan^-1 \(\left(\frac{3 a^{2} x-x^{3}}{a\left(a^{2}-3 x^{2}\right)}\right)\)
Solution:
Put x = a tan θ

ii) tan^-1 (sec x + tan x)
Solution:

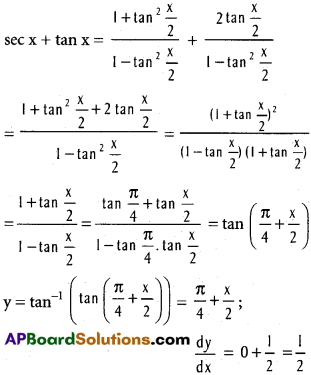
iii) tan^-1 \(\left(\frac{\sqrt{1+x^{2}}-1}{x}\right)\)
Solution:
Put x = tan θ

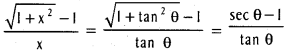
iv) (logx)^{tan x}
Solution:
log y = log (log x)^{tan x}
= (tan x). log (log x)
Differentiating w.r.to x
\(\frac{1}{y}\) . \(\frac{dy}{dx}\) = tan x . \(\frac{d}{dx}\) (log(log x)) + log(logx) \(\frac{d}{dx}\) (tan x)
= tan x. \(\frac{1}{log x}\) . \(\frac{1}{x}\) + log(log x). sec² x

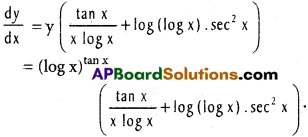
v) (x^x)^x = x^x²
Solution:
log y = log x^x² = x². log x
\(\frac{1}{y}\) . \(\frac{dy}{dx}\) = x² . \(\frac{d(\log x)}{d x}\) + (log x ) \(\frac{d}{dx}\) (x²)
= x². \(\frac{1}{x}\) + 2x. log x
= x + 2x log x = x (1 + 2 log x).
= x (log e + log x²)
= x. log (ex²)
\(\frac{dy}{dx}\) = y. x. log (ex²)
= x^x² . x. log (ex²)
= x^{x² +1} log (ex²)

vi) 20^{log (tan x)}
Solution:
log y = log (20)^{log (tan x)}
= log (tan x) log 20
Differentiating w. r. to x

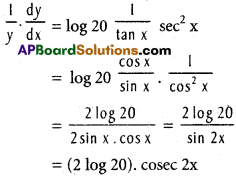
\(\frac{dy}{dx}\) = y. (2 log 20). cosec 2x
= 20^{log (tan x)} (2 log 20). cosec 2x

vii) x^x + e^ex
Solution:
Let y₁ = x^x and y₂ = e^ex so that y = y₁ + y₂.
y₁ = x^x ⇒ log y₁ = log x^x = x log x

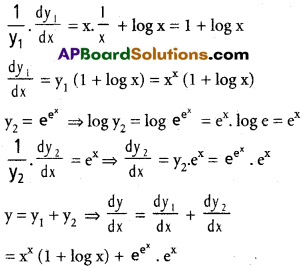
viii) x. log x. log (log x)
Solution:
\(\frac{dy}{dx}\) = x. log x \(\frac{d}{dx}\) (log. (log x)) + log (log x) logx. 1 + x. log (log x) \(\frac{1}{x}\).
= x log x. \(\frac{1}{log x}\) . \(\frac{1}{x}\) + log x. log (log x) + log (log x)
= 1 + log (logx) (1 + logx) = 1 + log (logx) + log x log (log x)
= log e + log (log x) + log x. log (log x)
= log (e log x) + log x. log (log x)

ix) e^-ax² sin (x log x)
Solution:
\(\frac{dy}{dx}\) = e^-ax² . \(\frac{d}{dx}\) (sin (x log x)) dx + sin (x log x) \(\frac{d}{dx}\) (e^-ax²)
= e^-ax² cos (x log x). (x . \(\frac{1}{x}\) + log x) + sin (x log x) e^-ax² (-2ax)
= e^-ax² [(cos (x log x) (1 + log x) – 2 ax. sin (x log x)]
= e^-ax² (cos (x log x) (log ex) -2 ax. sin (x log x))

x) sin^-1\(\left(\frac{2^{x+1}}{1+4^{x}}\right)\) (Put 2ⁿ = tan θ)
Solution:
sin^-1\(\left(\frac{2^{x+1}}{1+4^{x}}\right)\)
Put 2^x = tan θ.

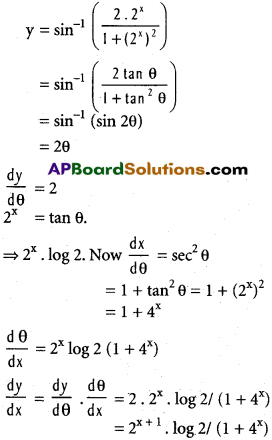
Question 2.
Find \(\frac{dy}{dx}\) for the following functions.
i) x = 3 cos t – 2 cos³ t,
y = 3 sin t – 2 sin³ t
Solution:
\(\frac{dx}{dt}\) = – 3 sin t – 2(3 cos² t) (- sin t)
= – 3 sin t + 6 cos² t (sin t)
= 3 sin t (2 cos² t – 1)
= 3 sin t. cos 2t
y = 3 sin t – 2 sin³ t
\(\frac{dy}{dt}\) = 3 cos t – 2 (3 sin² t) (- cos t)
= 3 cos t (1 – 2 sin² t)
= 3 cos t. cos 2t
\(\frac{dy}{dx}\) = \(\frac{\left(\frac{\mathrm{dy}}{\mathrm{dt}}\right)}{\left(\frac{\mathrm{dx}}{\mathrm{dt}}\right)}=\frac{3 \cos t \cos 2 t}{3 \sin t \cos 2 t}\) = cot t

ii) x = \(\frac{3 a t}{1+t^{3}}\), y = \(\frac{3 a t^{2}}{1+t^{3}}\)
Solution:

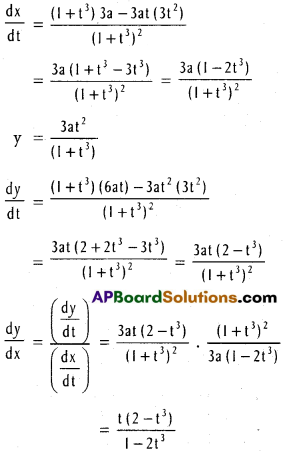
iii) x = a (cos t + t sin t), y = a (sin t – t cos t)
Solution:
\(\frac{dx}{dt}\) = a (- sin t + t cos t + sin t) = at cos t
∴ y = a (sin t – t cos t)
\(\frac{dy}{dt}\) = a (cos t – cos t + t sin t) = at sin t
\(\frac{dy}{dx}\) = \(\frac{\left(\frac{\mathrm{dy}}{\mathrm{dt}}\right)}{\left(\frac{\mathrm{dx}}{\mathrm{dt}}\right)}=\frac{at\cos t}{at\cos t}\) = tan t

iv) x = a\(\left[\frac{\left(1-t^{2}\right)}{1+t^{2}}\right], \mathbf{y}=\frac{2 b t}{1+t^{2}}\)
Solution:

Question 3.
Differentiate f(x) with respect to g(x) for the following.
i) f(x) = log_a^x, g(x) = a^x.
Solution:
y = log_a^x = \(\frac{\log x}{\log _{c}^{a}}\)

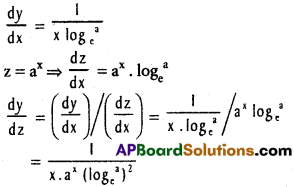
ii) f(x) = sec^-1 (\(\frac{1}{2x^{2}-1}\)) g(x) = \(\sqrt{1-x^{2}}\)
Solution:
Let y = sec^-1 (\(\frac{1}{2x^{2}-1}\)) and z = \(\sqrt{1-x^{2}}\)
Put x = cos θ

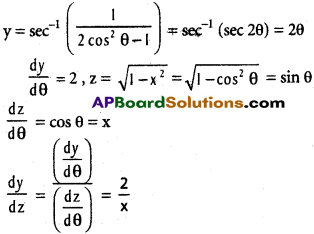
iii) f(x) = tan^-1\(\left(\frac{\sqrt{1+x^{2}}-1}{x}\right)\), g(x) = tan^-1 x.
Solution:
Let y = tan^-1\(\left(\frac{\sqrt{1+x^{2}}-1}{x}\right)\) and z = tan^-1 x
x = tan z

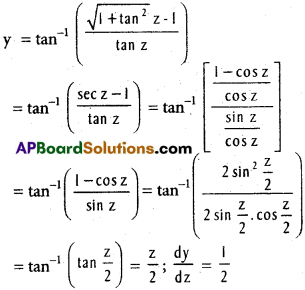
Question 4.
Find the derivative of the function y defined implicitly by each of the following equations.
i) x⁴ + y⁴ – a² xy = 0
Solution:
Differentiate w.r.to x
4x³ + 4y³ . \(\frac{dy}{dx}\) – a²(x. \(\frac{dy}{dx}\) + y . 1 = 0)
4x³ + 4y³ . \(\frac{dy}{dx}\) – a²x\(\frac{dy}{dx}\) – a²y = 0
4y³ – a²x) \(\frac{dy}{dx}\) = a²y – 4x³ ; \(\frac{dy}{dx}\) = \(\frac{a^{2} y-4 x^{3}}{4 y^{3}-a^{2} x}\)

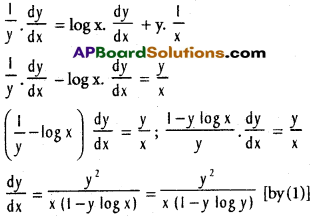
ii) y = x^y
Solution:
log y = log x^y = y log x
Differentiate w.r.to x

iii) y^x = x^{sin y}
Solution:
Take log on both sides
log y^x = log x^{sin y} ⇒ x. log y = (sin y) log x.
Differentiating w.r.to x

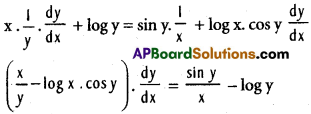
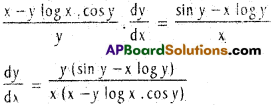
Question 5.
Establish the following.
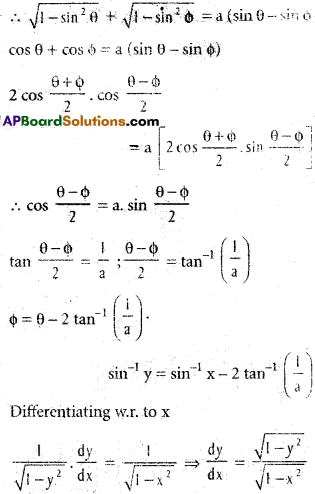
i) If \(\sqrt{1-x^{2}}+\sqrt{1-y^{2}}\) = a(x – y), than \(\frac{d y}{d x}=\frac{\sqrt{1-y^{2}}}{\sqrt{1-x^{2}}}\)
Solution:
Given \(\sqrt{1-x^{2}}+\sqrt{1-y^{2}}\) = a(x – y)
Put x = sin θ, y = sin Φ
Differentiating w.r. to x

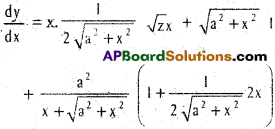
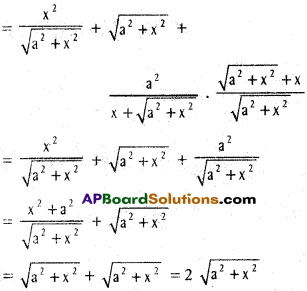
ii) If y = x \(\sqrt{a^{2}+x^{2}}\) + a² log (x + \(\sqrt{a^{2}+x^{2}}\)), then \(\frac{dy}{dx}\) = 2 \(\sqrt{a^{2}+x^{2}}\)
Solution:
y ⇒ x\(\sqrt{a^{2}+x^{2}}\) + a² log (x + \(\sqrt{a^{2}+x^{2}}\))

iii) If x^{log y} = log x, then
Solution:
Given x^{log y} = log x, log x^{log y} = log log x
(log y) (log x) = log(logx).
Differentiating w.r.to x

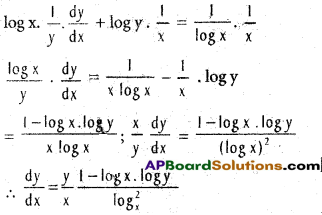
iv) If y = Tan^-1 \(\frac{2x}{1-x^{2}}\) + Tan^-1 \(\frac{3x-x^{3}}{1-3x^{2}}\) – tan^-1 \(\frac{4x-4x^{3}}{1-6x^{2}+x^{4}}\) than \(\frac{dy}{dx}\) = \(\frac{1}{1+x^{2}}\)
Solution:
Put x = tan θ

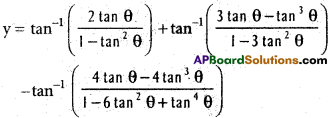
= tan^-1 (tan 2θ) + tan^-1 (tan 3θ) – tan^-1 (tan 4θ)
= 2θ + 3θ – 4θ = θ = tan^-1 x
∴ \(\frac{dy}{dx}\) = \(\frac{1}{1+x^{2}}\)

v) If x^y = y^x, then \(\frac{dy}{dx}\) = \(\frac{y(x log y – y)}{x(y log x – x)}\)
Solution:
Given x^y = y^x ; log x^y = log y^x
y log x = x log y
Differentiating w.r.to x

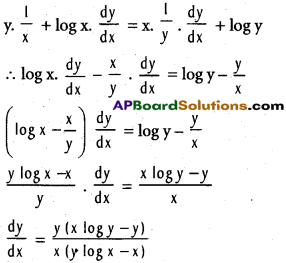
vi) If x^2/3 + y^2/3 = a^2/3 then \(\frac{dy}{dx}\) = -3 √y/x
Solution:
Given x^2/3 + y^2/3 = a^2/3
Differentiating w.r.to x

Question 6.
Find the derivative \(\frac{dy}{dx}\) of each of the following functions.
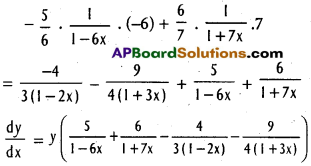
i) y = \(\frac{(1-2 x)^{2 / 3}(1+3 x)^{-3 / 4}}{(1-6 x)^{5 / 6}(1+7 x)^{-6 / 7}}\)
Solution:
log y = log \(\left\{\frac{(1-2 x)^{2 / 3}(1+3 x)^{-3 / 4}}{(1-6 x)^{5 / 6}(1+7 x)^{-6 / 7}}\right\}\)
= log (1 – 2x)^2/3 + log (1 + 3x)^-3/4 – log (1 – 6x)^5/6 – log (1 + 7x)^-6/7
= \(\frac{2}{3}\) log (1 – 2x) – \(\frac{3}{4}\) log (1 + 3x) – \(\frac{5}{6}\) log (1 – 6x) + \(\frac{6}{7}\) log (1 + 7x)
Differentiating w.r.to x
\(\frac{1}{y}\).\(\frac{dy}{dx}\) = \(\frac{2}{3}\) . \(\frac{1(-2)}{1-2x}\) – \(\frac{3}{4}\) . \(\frac{1}{1+3x}\) . 3

ii)

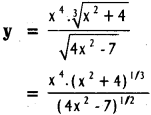
Solution:
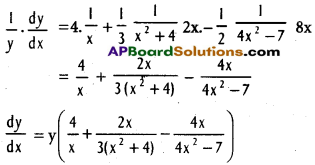
log y = log x⁴ + log (x² + 4)^1/3 – log (4x² – 7)^1/2
= 4 log x + \(\frac{1}{3}\) log (x² + 4) – \(\frac{1}{2}\) log (4x² – 7)
Differentiating w.r.to x

iii) y = \(\frac{(a-x)^{2}(b-x)^{3}}{(c-2 x)^{3}}\)
Solution:
log y = log \(\frac{(a-x)^{2}(b-x)^{3}}{(c-2 x)^{3}}\)
= log (a – x)² + log (b – x)³ – log (c – 2x)³
= 2 log (a – x) + 3 log (b – x) – 3 log (c – 2x)
Differentiating w.r.to x

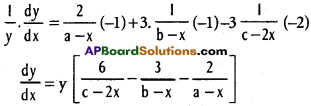
iv)

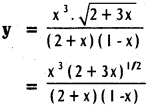
Solution:
log y = log \(\frac{x^{3}(2+3 x)^{1 / 2}}{(2+x)(1-x)}\)
= log x³ + log (2 + 3x)^1/2 – log (2 + x) – log (1 – x)
= 3 log x + \(\frac{1}{2}\) log (2 + 3x) – log (2 + x) – log (1 – x)
Differentiating w.r. to x

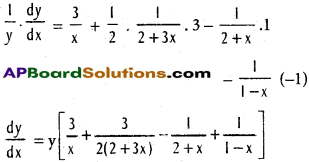
v) y = \(\sqrt{\frac{(x-3)\left(x^{2}+4\right)}{3 x^{2}+4 x+5}}\)
Solution:
log y = log(\(\frac{(x-3)\left(x^{2}+4\right)}{3 x^{2}+4 x+5}\))^1/2
= \(\frac{1}{2}\) log \(\frac{(x-3)\left(x^{2}+4\right)}{3 x^{2}+4 x+5}\)
= \(\frac{1}{2}\) (log (x – 3) + log (x² + 4) – log (3x² + 4x + 5))

III.

Question 1.
Find the derivatives of the following functions.
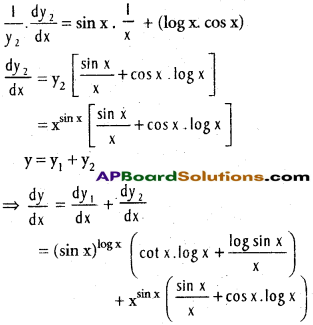
i) y = (sin x)^logx + x^{sin x}
Solution:
Let y₁ =(sinx)^logx, y₂ = x^{sin x} so that y = y₁ + y₂
y₁ = (sin x)^logx
log y₁= log{ (Sin x)^logx} = log x. log (sin x)
Differentiating w.r. to x

y₂ = x^{sin x}
log y₂ = (log x)^{sin x} = sin x. logx

ii) x^xx
Solution:
log y = log x(x^xx) = x^x. log X
\(\frac{1}{y}\) \(\frac{dy}{dx}\) = x^x. \(\frac{1}{x}\) + (log x). x^x (1 + log x)
[\(\frac{d}{dx}\)(x^x) = x^x (1 + log x)]
= x^x-1 [1+ x log x (log e + log x)]
= x^x-1 (1 + x. log x. log ex)
\(\frac{dy}{dx}\) = y.x^x-1 (1 + x log x. log ex)
= x^(xx) . x^x-1 (1 + x log x. log ex)
= x^xx+x-1 (1 + x log x. log ex)

iii) (sin x)^x + x^{sin x}
Solution:
Let y₁ = (sin x)^x and y₂ = x^{sin x}
so that y = y₁ + y₂
log y₁ = log (sin x)^x = x. log sin x

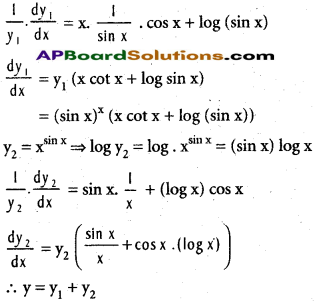
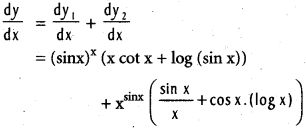
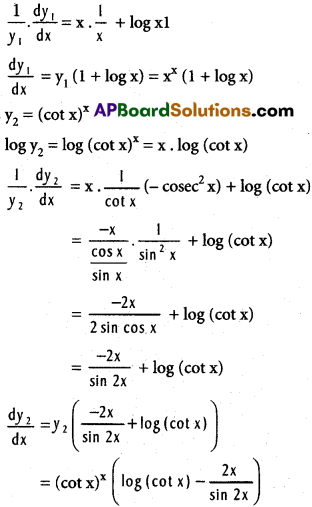
iv) x^x + (cot x)^x
Solution:
Let y₁ = x^x and y₂ = (cot x)^x
log y₁ = log x^x = x log x

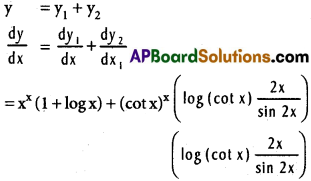
Question 2.
Establish the following
i) If x^y + y^x = a^b then
\(\frac{dy}{dx}\) = \(-\left(\frac{y \cdot x^{y-1}+y^{x} \cdot \log y}{x^{y} \cdot \log x+x \cdot y^{x-1}}\right)\)
Solution:
Let y₁ = x^y and y₂ = y^x. so that y₁ + y₂ = a^b
logy₁ = log x^y = y logx

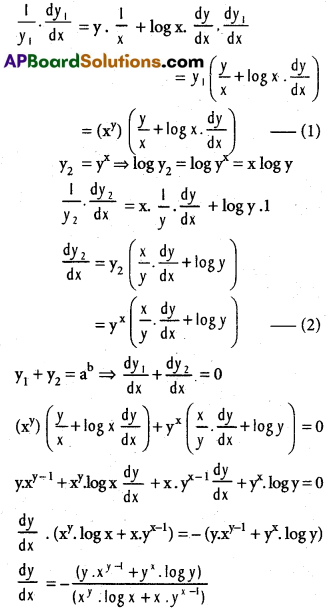
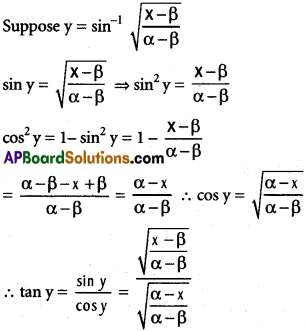
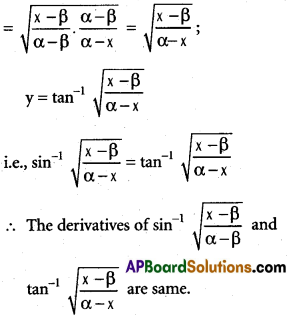
ii) If f(x) = sin^-1\(\sqrt{\frac{x-\beta}{\alpha-\beta}}\) and
g(x) = tan \(\sqrt{\frac{x-\beta}{\alpha-x}}\) than
f'(x) = g'(x) (β < x < α)
Solution:

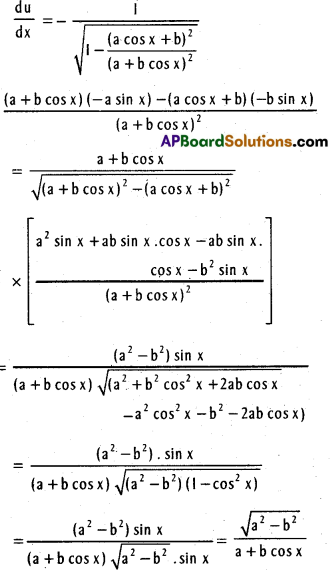
iii) If a > b > 0 and 0 < x < π
f(x) = (a – b)^-1/2 . cos^-1\(\left(\frac{a \cos x+b}{a+b \cos x}\right)\), than f'(x) = (a + b cos x)^-1
Solution:
Let u = cos^-1\(\left(\frac{a \cos x+b}{a+b \cos x}\right)\)

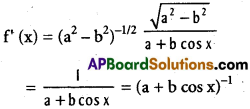
Question 3.
Differentiate (x² – 5x + 8) (x³ + 7x + 9) by
i) Using product
ii) Obtaining a single polynomial expanding the product
iii) Logarithmic differentiation do they all give the same answer?
Solution:
Do Product rule:
y = (x² – 5x + 8) (x³ + 7x + 9)
\(\frac{dy}{dx}\) = (x² – 5x + 8) \(\frac{d}{dx}\)(x³ + 7x + 9) + (x³ + 7x + 9) \(\frac{d}{dx}\)(x² – 5x + 8)
= (x² – 5x + 8)(3x² + 7) + (x³ + 7x + 9)(2x – 5)
= 3x⁴ – 15x³ + 24x² + 7x² – 35x + 56 + 2x⁴ + 14x² + 18x – 15x³ – 35x – 45
= 5x⁴ – 20x³ + 45x² – 52x + 11 ……….. (1)

ii) Expanding the product :
Solution:
y = (x² – 5x + 8) (x³ + 7x + 9)
= 5x⁵ + 7x³ + 9x² – 5x⁴ -35x² – 45x + 8x³ + 56x + 72
= x⁵ – 5x⁴ + 15x³ – 26x² + 11x +72
\(\frac{dy}{dx}\) = 5x⁴ – 20x³ + 45x² – 52x + 11 ……….. (2)

iii) y = (x² – 5x + 8) (x³ + 7x + 9)
Solution:
log y = log (x² – 5x + 8) (x³ + 7x + 9)
= log (x² – 5x + 8) + log (x³ + 7x + 9)

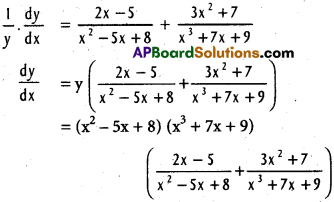
= (2x – 5)(x³ + 7x + 9) + (x² – 5x + 8)(3x² + 7)
= 2x⁴ + 14x² + 18x – 5x³ – 35x – 45 + 3x⁴ -15x³ + 24x² + 7x² – 35x + 56
= 5x⁴ – 20x³ +45x² – 52x + 11 ……….. (3)
From (1), (2) and (3) we observe that all the three give same answer.