Practicing the Intermediate 2nd Year Maths 2A Textbook Solutions Inter 2nd Year Maths 2A Permutations and Combinations Solutions Exercise 5(d) will help students to clear their doubts quickly.
Intermediate 2nd Year Maths 2A Permutations and Combinations Solutions Exercise 5(d)
I.
Question 1.
Find the number of ways of arranging the letters of the word.
(i) INDEPENDENCE
(ii) MATHEMATICS
(iii) SINGING
(iv) PERMUTATION
(v) COMBINATION
(vi) INTERMEDIATE
Solution:
(i) The word INDEPENDENCE contains 12 letters in which there are 3 N’s are alike, 2 D’s are alike, 4 E’s are alike and rest are different.
∴ The number of required arrangements = \(\frac{(12) !}{4 ! 3 ! 2 !}\)
(ii) The word MATHEMATICS contains 11 letters in which there are 2 M’s are alike, 2 A’s are alike, 2 T’s are alike and rest are different.
∴ The number of required arrangements = \(\frac{(11) !}{2 ! 2 ! 2 !}\)
(iii) The word SINGING contains 7 letters in which there are 2 I’s are alike, 2 N’s are alike, 2 G’s are alike and rest is different.
∴ The number of required arrangements = \(\frac{7 !}{2 ! 2 ! 2 !}\)
(iv) The word PERMUTATION contains 11 letters in which there are 2 T’s are alike and rest are different.
∴ The number of required arrangements = \(\frac{(11) !}{2 !}\)
(v) The word COMBINATION contains 11 letters in which there are 2 O’s are alike, 2 I’s are alike, 2 N’s are alike and rest are different.
∴ The number of required arrangements = \(\frac{(11) !}{2 ! 2 ! 2 !}\)
(vi) The word INTERMEDIATE contains 12 letters in which there are 2 I’s are alike, 2 Ts are alike, 3 E’s are alike and rest are different.
∴ The number of required arrangements = \(\frac{(12) !}{2 ! 2 ! 3 !}\)
![]()
Question 2.
Find the number of 7-digit numbers that can be formed using 2, 2, 2, 3, 3, 4, 4.
Solution:
In the given 7 digits, there are three 2 ‘s, two 3’s and two 4’s.
∴ The number of 7 digited numbers that can be formed using the given digits = \(\frac{7 !}{3 ! 2 ! 2 !}\)
II.
Question 1.
Find the number of 4-letter words that can be formed using the letters of the word RAMANA.
Solution:
The given word RAMANA has 6 letters in which there are 3 A’s alike and the rest are different.
Using these 6 letters, 3 cases arise to form 4 letter words.
Case I: All different letters R, A, M, N
Number of 4 letter words formed = 4! = 24
Case II: Two like letters A, A and two out of R, M, N
The two different letters can be choosen from 3 letters in 3C2 = 3 ways.
∴ Number of 4 letters word formed = 3 × \(\frac{4 !}{2 !}\)
= 3 × 12
= 36
Case III: Three like letters A, A, A and one out of R, M, N.
One letter can be choosen from 3 different letters in 3C1 = 3 ways.
∴ Number of 4 letter words formed = 3 × \(\frac{4 !}{3 !}\)
= 3 × 4
= 12
∴ Total number of 4 letter words formed from the word RAMANA = 24 + 36 + 12 = 72
Question 2.
How many numbers can be formed using all the digits 1, 2, 3, 4, 3, 2, 1 such that even digits always occupy even places?
Solution:
In the given 7 digits, there are two 1’s, two 2’s, two 3’s and one 4.
The 3 even places can be occupied by the even digits 2, 4, 2 in \(\frac{3 !}{2 !}\). (Even place is shown by E)
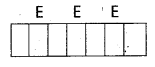
The remained odd places can be occupied by the odd digits 1, 3, 3, 1 in \(\frac{4 !}{2 ! 2 !}\) ways.
∴ The number of required arrangements = \(\frac{3 !}{2 !} \times \frac{4 !}{2 ! \times 2 !}\)
= 3 × 6
= 18
![]()
Question 3.
In a library, there are 6 copies of one book, 4 copies each of two different books, 5 copies each of three different books and 3 copies each of two different books. Find the number of ways of arranging all these books in a shelf in a single row.
Solution:
Total number of books in a library = 6 + (4 × 2) + (5 × 3) + (3 × 2) = 35
∴ The number of required arrangements = \(\frac{(35) !}{6 !(4 !)^2(5 !)^3(3 !)^2}\)
Question 4.
A book store has ‘m’ copies each of, ‘n’ different books. Find the number of ways of arranging the books in a shelf in a single row.
Solution:
Total number of books in a book store are = m × n = mn
∴ The number of required arrangements = \(\frac{(m n) !}{(m !)^n}\)
Question 5.
Find the number of 5-digit numbers that can be formed using the digits 0, 1, 1, 2, 3.
Solution:
‘O’ can also be taken as one digit,
the number of 5 digited number formed = \(\frac{5 !}{2 !}\) = 60
Among them, the numer that starts with zero is only 4 digit number.
The number of numbers start with zero = \(\frac{4 !}{2 !}\) = 12
Hence the number of 5 digit numbers that can be formed by using all the given digits = 60 – 12 = 48
Question 6.
In how many ways can the letters of the word CHEESE be arranged so that no two E’s come together?
Solution:
The given word contains 6 letters in which one C, one H, 3 E’s and one S.
Since no two E’s come together, first arrange the remaining 3 letters in 3! ways. Then we can find 4 gaps between them.
The 3 E’s can be arranged in these 4 gaps in \(\frac{{ }^4 P_3}{3 !}\) = 4 ways.
∴ The number of required arrangements = 3! × 4 = 24
III.
Question 1.
Find the number of ways of arranging the letters of the word ASSOCIATIONS. In how many of them
(i) all the three S’s come together
(ii) The two A’s do not come together
Solution:
Hint: The number of linear permutations of ‘n’ things in which ‘p’ alike things of one kind, ‘q’ alike things of 2nd kind, ‘r’ alike things of 3rd kind and the rest are different is \(\frac{n !}{p ! q ! r !}\)
The given word ASSOCIATIONS has 12 letters in which there are 2 A’s are alike, 3 S’s are alike, 2 O’s are alike 2 I’s are alike and rest are different.
∴ They can be arranged = \(\frac{(12) !}{2 ! 3 ! 2 ! 2 !}\)
(i) Treat the 3 S’s as one unit. Then we have 9 + 1 = 10 entities in which there are 2A’s are alike, 20’s are alike, 2 I’s are alike and rest are different.
They can be arranged in \(\frac{(10) !}{2 ! 2 ! 2 !}\) ways.
The 3 S’s among themselves can be arranged in \(\frac{3 !}{3 !}\) = 1 way
∴ The number of required arrangements = \(\frac{(10) !}{2 ! 2 ! 2 !}\)
(ii) Since 2 A’s do not come together, first arrange the remaining 10 letters in which there are 3 S’s are alike, 2 O’s are alike 2 I’s are alike and rest are different in \(\frac{(10) !}{3 ! 2 ! 2 !}\) ways.
Then we can find 11 gaps between them. The 2 A’s can be arranged in these 11 gaps in \(\frac{{ }^{11} P_2}{2 !}\) ways.
∴ The number of required arrangements = \(\frac{(10) !}{3 ! 2 ! 2 !} \times \frac{{ }^{11} P_2}{2 !}\)
![]()
Question 2.
Find the number of ways of arranging the letters of the word MISSING so that the two S’s are together and the two I’s are together.
Solution:
In the given word MISSING contains 7 letters in which there are 2 I’s are alike, 2 S’s are alike and the rest are different.
Treat the 2 S’s as one unit and 2 I’s as one unit. Then we have 3 + 1 + 1 = 5 entities. These can be arranged in 5! ways.
The 2 S’s can be arranged among themselves in \(\frac{2 !}{2 !}\) = 1 ways and the 2 I’s can be arranged among themselves in \(\frac{2 !}{2 !}\) = 1 way.
∴ The number of required arrangements = 5! × 1 × 1 = 120
Question 3.
If the letters of the word AJANTA are permuted in all possible ways and the words thus formed are arranged in dictionary order, find the ranks of the words
(i) AJANTA
(ii) JANATA
Solution:
The dictionary order of the letters of the word AJANTA is A A A J N T
(i) In the dictionary order first comes that words begin with the letter A.
If we fill the first place with A, we may set the word AJANTA.
Second place can be filled with A, and the remaining 4 places can be filled in 4! = 24 ways.
On proceeding like this, we get
A A – – – – – = 4! = 24
A J A A – – – = 2! = 2
A J A N A – = 1 = 1
AJANTA = 1 = 1
∴ Rank of the word AJANTA = 24 + 2 + 1 + 1 = 28
(ii) In the dictionary order first comes that words begin with the letter A.
If we fill the first place with A, the remaining 5 letters can be arranged in \(\frac{5 !}{2 !}\) ways (since there 2 A’s remain)
On proceeding like this, we get
A – – – – – – = \(\frac{5 !}{2 !}\) = 60
J A A – – – – = 3! = 6
J A N A A – – = 1 = 1
J A N A T A = 1 = 1
∴ Rank of the word JANATA is = 60 + 6 + 1 + 1 = 68.