Inter 2nd Year Maths 2B Circle Important Questions

Students get through Maths 2B Important Questions Inter 2nd Year Maths 2B Circle Important Questions which are most likely to be asked in the exam.

Intermediate 2nd Year Maths 2B Circle Important Questions

Question 1.
If x² + y² + 2gx + 2fy – 12 = 0 represents a circle with centre (2, 3), find g, f and its radius. [Mar. 11]
Solution:
Circle is x² + y² + 2gx + 2fy – 12 = 0
C = (-g, -f) C = (2, 3)
∴ g = – 2, f = – 3, c = – 12
Radius = \(\sqrt{g^{2}+f^{2}-c}\)
= \(\sqrt{4+9+12}\) = 5 units

Question 2.
Obtain the parametric equation of x² + y² = 4 [Mar. 14]
Solution:
Equation of the circle is x² + y² = 4
C (0, 0), r = 2
Parametric equations are
x = – g + r cos θ = 2 cos θ
y = – b + r sin θ = 2 sin θ, 0 < θ < 2π

Question 3.
Obtain the parametric equation of (x – 3)² + (y – 4)² = 8² [A.P. Mar. 16; Mar. 11]
Solution:
Equation of the circle is (x – 3)² + (y – 4)² =8²
Centre (3, 4), r = 8
Parametric equations are
x = 3 + 8 cos θ, y = 4 + 8 sin θ, 0 < θ < 2π

Question 4.
Find the power of the point P with respect to the circle S = 0 when
ii) P = (-1,1) and S ≡ x² + y² – 6x + 4y – 12
Solution:
Power of the point = S₁₁
= 1 + 1 + 6 + 4 – 12 = 0

iii) P = (2, 3) and S ≡ x² + y² – 2x + 8y – 23
Power of the point = S₁₁
= 4 + 9 – 4 + 24 – 23 = 10

iv) P = (2, 4) and S ≡ x² + y² – 4x – 6y – 12
Power of the point = 4 + 16 – 8 – 24 – 12
= -24.

Question 5.
If the length of the tangent from (5, 4) to the circle x² + y² + 2ky = 0 is 1 then find k. [Mar. 15; Mar. 01]
Solution:
= \(\sqrt{S_{11}}=\sqrt{(5)^{2}+(4)^{2}+8 k}\)
But length of tangent = 1
∴ 1 = \(\sqrt{25+16+8k}\)
Squaring both sides we get 1 = 41 + 8k
k = – 5 units.

Question 6.
Find the polar of (1, -2) with respect to x² + y² – 10x – 10y + 25 = 0 [T.S. Mar. 15]
Solution:
Equation of the circle is x² + y² – 10x – 10y + 25 = 0
Equation of the polar is S₁ = 0
Polar of P(1,-2) is
x . 1 + y(-2) – 5(x + 1) – 5(y – 2) + 25 = 0
⇒ x – 2y – 5x – 5 – 5y + 10 + 25 = 0
⇒ -4x – 7y + 30 = 0
∴ 4x + 7y – 30 = 0

Question 7.
Find the value of k if the points (1, 3) and (2, k) are conjugate with respect to the circle x² + y² = 35. [T.S. Mar. 16]
Solution:
Equation of the circle is
x² + y² = 35
Polar of P(1, 3) is x. 1 + y. 3 = 35
x + 3y = 35
P(1, 3) and Q(2, k) are conjugate points
The polar of P passes through Q
2 + 3k = 35
3k = 33
k = 11

Question 8.
If the circle x² + y² – 4x + 6y + a = 0 has radius 4 then find a. [A.P. Mar. 16]
Solution:
Equation of the circle is
x² + y² – 4x + 6y + a = 0
2g = – 4, 2f = 6, c = a
g = -2, f = 3, c = a
radius = 4 ⇒ \(\sqrt{g^{2}+f^{2}-c}\) = 4
\(\sqrt{4+9-a}\) = 4
13 – a = 16
a = 13 – 16 = -3

Question 9.
Find the value of ‘a’ if 2x² + ay² – 3x + 2y – 1 =0 represents a circle and also find its radius.
Solution:
General equation of second degree
ax² + 2hxy + by² + 2gx + 2fy + c = 0
Represents a circle, when
a = b, h = 0, g² + f² – c ≥ 0
In 2x² + ay² – 3x + 2y – 1 = 0
a = 2, above equation represents circle.
x² + y² – \(\frac{3}{2}\) x + y – \(\frac{1}{2}\) = 0
2g = – \(\frac{3}{2}\); 2f = 1; c = – \(\frac{1}{2}\)
c = (-g, -f) = (\(\frac{+3}{4}\), \(\frac{-1}{2}\))
Radius = \(\sqrt{g^{2}+f^{2}-c}\) = \(\sqrt{\frac{9}{16}+\frac{1}{4}+\frac{1}{2}}\)
= \(\frac{\sqrt{21}}{4}\) units

Question 10.
If the abscissae of points A, B are the roots of the equation, x² + 2ax – b² = 0 and ordinates of A, B are root of y² + 2py – q² = 0, then find the equation of a circle for which \(\overline{\mathrm{AB}}\) is a diameter. [Mar. 14]
Solution:
Equation of the circle is
(x – x₁) (x – x₂) + (y – y₁) (y – y₂) = 0
x² – x(x₁ + x₂) + x₁x₂ + y² – y (y₁ + y₂) + y₁y₂ = 0
x₁, x₂ are roots of x² + 2ax – b² = 0
y₁, y₂ are roots of y² + 2py – q² = 0
x₁ + x₂ = – 2a
x₁x₂ = -b²

y₁ + y₂ = -2p
y₁y₂ = -q²
Equation of circle be
x² – x (- 2a) – b² + y²– y (- 2p) – q² = 0
x² + 2xa + y² + 2py – b² – q² = 0

Question 11.
Find the equation of a circle which passes through (4, 1) (6, 5) and having the centre on 4x + 3y – 24 = 0 [A.P. Mar. 16; Mar. 14]
Solution:
Equation of circle be x² + y² + 2gx + 2fy + c = 0 passes through (4, 1) and (6, 5) then
4² + 1² + 2g(4) + 2f(1) + c = 0 ………….. (i)
6² + 5² + 2g(6) + 2f(5) + c = 0 ……………… (ii)
Centre lie on 4x + 3y – 24 = 0
∴ 4(-g) + 3 (-f) – 24 = 0
(ii) – (i) we get
44 + 4g + 8f = 0
Solving (iii) and (iv) we get
f = -4, g = -3, c = 15
∴ Required equation of circle is
x² + y² – 6x – 8y + 15 = 0

Question 12.
Find the equation of a circle which is concentric with x² + y² – 6x – 4y – 12 = 0 and passing through (- 2, 14). [Mar. 14]
Solution:
x² + y² – 6x – 4y – 12 = 0 …………… (i)
C = (- g, – f)
= (3, 2)
Equation of circle concentric with (i) be
(x – 3)² + (y – 2)² = r²
Passes through (-2, 14)
∴ (- 2 – 3)² + (14 – 2)² = r²
169 = r²
Required equation of circle be
(x – 3)² + (y – 2)² = 169
x² + y² – 6x – 4y – 156 = 0

Question 13.
Find the equation of the circle whose centre lies on the X-axis and passing through (- 2, 3) and (4, 5). [A.P. & T.S. Mar. 15]
Solution:
x² + y² + 2gx + 2fy + c = 0 ……………… (i)
(- 2, 3) and (4, 5) passes through (i)
4 + 9 – 4g + 6f + c = 0 ………………………. (ii)
16 + 25 + 8g + 10f + c = 0 …………………. (iii)
(iii) – (ii) we get
28 + 12g + 4f = 0
f + 3g = – 7
Centre lies on X – axis then f = 0
g = -, \(\frac{7}{3}\), f = 0, c = \(\frac{67}{3}\) -, we get by substituting g; f in equation (ii)
Required equation will be 3(x² + y²) – 14x – 67 = 0

Question 14.
Find the equation of circle passing through (1, 2); (3, – 4); (5, – 6) three points.
Solution:
Equation of circle is
x² + y² + 2gx + 2fy + c = 0
1 + 4 + 2g + 4f + c = 0 …………………… (i)
9 + 16 + 6g – 8f + c = 0 …………………… (ii)
25 + 36 + 10g – 12f + c = 0 ………………… (iii)
Subtracting (ii) – (i) we get
20 + 4g – 12f = 0
(or) 5 + g – 3f = 0 ……………….. (iv)
Similarly (iii) – (ii) we get
36 + 4g – 4f = 0
(or) 9 + g – f = 0 ………………… (v)
Solving (v) and (iv) we get
f = -2, g = – 11, c = 25
Required equation of circle be x² + y² – 22x – 4y + 25 = 0

Question 15.
Find the length of the chord intercepted by the circle x² + y² – 8x – 2y – 8 = 0 on the line x + y + 1 = 0 [T.S. Mar. 16]
Solution:
Equation of the circle is x² + y² – 8x – 2y – 8 = 0
Centre is C(4, 1), r = \(\sqrt{16+1+8}\) = 5
Equation of the line is x + y + 1 = 0
P = distance from the centre = \(\frac{|4+1+1|}{\sqrt{1+1}}\)
= \(\frac{6}{\sqrt{2}}\) = 3\(\sqrt{2}\)
Length of the chord = 2\(\sqrt{r^{2}-p^{2}}\)
= 2\(\sqrt{25-18}\)
= 2\(\sqrt{7}\) units.

Question 16.
Find the pair of tangents drawn from (1, 3) to the circle x² + y² – 2x + 4y – 11 =0 and also find the angle between them. [T.S. Mar. 16]
Solution:
SS₁₁ = S₁²
(x² + y² – 2x + 4y – 11) (1 + 9 – 2 + 12 – 11) = [x + 3y – 1 (x + 1) + 2 (y + 3) – 11]²
(x² + y² – 2x + 4y – 11) 9 = [5y – 6]²
9x² + 9y² – 18x + 36y – 99
= 25y² + 36 – 60y
9x² – 16y² – 18x + 96y – 135 = 0
cos θ = \(\frac{|a+b|}{\sqrt{(a-b)^{2}+4 h^{2}}}\) = \(\frac{|9-16|}{\sqrt{(25)^{2}}}\)
= \(\frac{|-7|}{25}\) = \(\frac{7}{25}\) ⇒ θ = cos^-1 (\(\frac{7}{25}\))

Question 17.
Find the value of k if the points (4, 2) and (k, -3) are conjugate points with respect to the circle x² + y² – 5x + 8y + 6 = 0. [T.S. Mar. 17]
Solution:
Equation of the circle is x² + y² – 5x + 8y + 6 = 0
Polar of P(4, 2) is
x . 4 + y . 2 – \(\frac{5}{2}\) (x + 4) + 4 (y + 2) + 6 = 0
8x + 4y – 5x – 20 + 8y + 16 + 12 = 0
3x + 12y + 8 = 0
P(4, 2), Q(k, -3) are conjugate points
Polar of P passes through Q
∴ 3k – 36 + 8 = 0
3k = 28 ⇒ k = \(\frac{28}{3}\)

Question 18.
If (2, 0), (0,1), (4, 5) and (0, c) are concyclic, and then find c. [A.P. & T.S. Mar. 15]
Solution:
x² + y² + 2gx + 2fy + c₁ = 0
Satisfies (2, 0), (0, 1) (4, 5) we get
4 + 0 + 4g + c₁ = 0 …………………. (i)
0 + 1 + 2g. 0 + 2f + c₁ = 0 – (ii)
16 + 25 + 8g + 10f + c₁ = -0 ……………. (iii)
(ii) – (i) we get
– 3 – 4g + 2f = 0
4g – 2f = – 3 ……………… (iv)
(ii) – (iii) we get
– 40 – 8g – 8f = 0 (or)
g + f = – 5 …………………… (v)
Solving(iv) and (v) we get
g = –\(\frac{13}{6}\), f = –\(\frac{17}{6}\)
Substituting g and f values in equation (i) we get
4 + 4 (-\(\frac{13}{6}\)) + c₁ = 0
c₁ = \(\frac{14}{3}\)
Now equation x² + y² – \(\frac{13}{3}\) x – \(\frac{17}{3}\) y + \(\frac{14}{3}\) = 0
Now circle passes through (0, c) then
c² – \(\frac{17}{3}\) c + \(\frac{14}{3}\) = 0
3c² – 17c + 14 = 0
⇒ (3c – 14) (c – 1) = 0
(or)
c = 1 or \(\frac{14}{3}\)

Question 19.
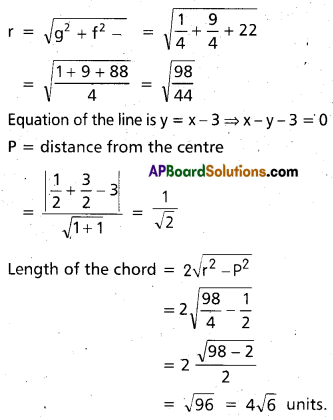
Find the length of the chord intercepted by the circle x² + y² – x + 3y – 22 = 0 on the line y = x – 3. [May 11; Mar. 13]
Solution:
Equation of the circle is
S ≡x² + y² – x + 3y – 22 = 0
Centre C(\(\frac{1}{2}\), –\(\frac{3}{2}\))

Question 20.
Find the equation of the circle with centre (-2, 3) cutting a chord length 2 units on 3x + 4y + 4 = 0 [Mar. 11]
Solution:
Equation of the line is 3x + 4y + 4 = 0
P = Length of the perpendicular .

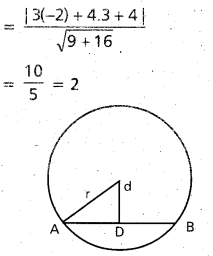
Length of the chord = 2λ = 2 ⇒ λ = 1
If r is the radius of the circle then
r² = 2² + 1² – 4 + 1 = 5
Centre of the circle is (-2, 3)
Equation of the circle is (x + 2)² + (y – 3)² = 5
x² + 4x + 4 + y² – 6y + 9 – 5 = 0
i.e., x² + y² + 4x – 6y + 8 = 0

Question 21.
Find the pole of 3x + 4y – 45 = 0 with respect to x² + y² – 6x – 8y + 5 = 0. [A.P. Mar. 16]
Solution:
Equation of polar is
xx₁ + yy₁ – 3(x + x₁) – 4(y + y₁) + 5 = 0
x(x₁ – 3) + y(y₁ – 4) – 3x₁ – 4y₁ + 5 = 0 …………………. (i)
Polar equation is same 3x + 4y – 45 = 0 ……………….. (ii)
Comparing (i) and (ii) we get

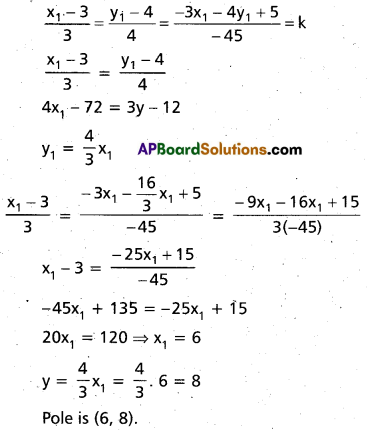
Question 22.
i) Show that the circles x² + y² – 6x – 2y + 1 = 0 ; x² + y² + 2x – 8y + 13 = 0 touch each other. Find the point of contact and the equation of common tangent at their point of contact. [A.P. Mar. 16; Mar. 11]
Solution:
Equations of the circles are
S₁ ≡ x² + y² – 6x – 2y + 1 = 0
S₂ ≡x² + y² + 2x – 8y + 13 = 0
Centres are A (3, 1), B(-1, 4)
r₁ = \(\sqrt{9+1+1}\) = 3, r₁ = \(\sqrt{1+16-13}\) = 2
AB = \(\sqrt{(3+1)^{2}+(1-4)^{2}}\) = \(\sqrt{16+9}\) = \(\sqrt{25}\) = 5
AB = 5 = 3 + 2 = r₁ + r₁
∴ The circles touch each other externally.
The point of contact P divides AB internally in the ratio r₁ : r₂ = 3 : 2
Co- ordinates of P are
\(\left(\frac{3(-1)+2.3}{5}, \frac{3.4+2.1}{5}\right)\) i.e., P\(\left(\frac{3}{5}, \frac{14}{5}\right)\)
Equation of the common tangent is S₁ – S₂ = 0
-8x + 6y – 12 = 0 (or) 4x – 3y + 6 = 0

ii) Show that x² + y² – 6x – 9y + 13 = 0, x² + y² – 2x – 16y = 0 touch each other. Find the point of contact and the equation of common tangent at their point of contact.
Solution:
Equations of the circles are
S₁ ≡ x² + y² – 6x – 9y + 13 = 0
S₂ ≡ x² + y² – 2x – 16y = 0
centres are A(3, \(\frac{9}{2}\)), B(1, 8)
r₁ = \(\sqrt{9+\frac{81}{4}-13}\) = \(\frac{\sqrt{65}}{2}\), r₂ = \(\sqrt{1+64}\)
= \(\sqrt{65}\)
AB = \(\sqrt{(3-1)^{2}+\left(\frac{9}{2}-8\right)^{2}}\) = \(\sqrt{4+\frac{49}{4}}\)
= \(\frac{\sqrt{65}}{2}\)
AB = |r₁ – r₂|
∴ The circles touch each other internally. The point of contact ‘P’ divides AB
externally in the ratio r₁ : r₂ = \(\frac{\sqrt{65}}{2}\) : \(\sqrt{65}\)
= 1 : 2 Co-ordinates of P are
\(\left(\frac{1(1)-2(3)}{1-2}, \frac{1(8)-2\left(\frac{9}{2}\right)}{1-2}=\left(\frac{-5}{-1}, \frac{-1}{-1}\right)\right.\) = (5, 1)
p = (5, 1)
∴ Equation of the common tangent is
S₁ – S₂ = 0
-4x + 7y + 13 = 0
4x – 7y – 13 = 0

Question 23.
Find the direct common tangents of the circles. [T.S. Mar. 15]
x² + y² + 22x – 4y – 100 = 0 and x² + y² – 22x + 4y + 100 = 0.
Solution:
C₁ = (-11, 2)
C₂ = (11, -2)
r₁ = \(\sqrt{121+4+100}\) = 15
r₂ = \(\sqrt{121+4-100}\) = 5
Let y = mx + c be tangent
mx – y + c = 0
⊥ from (-11, 2) to tangent = 15
⊥ from (11 ,-2) to tangent = 5

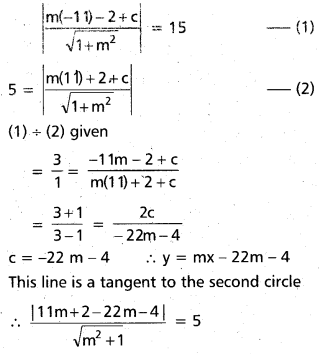
Squaring and cross multiplying
25 (1 + m²) = (11 m + 2 – 22m – 4)²
96m² + 44m – 21 = 0
⇒ 96m² + 72m – 28m – 21 = 0
m = \(\frac{7}{24}\), \(\frac{-3}{4}\)
c = \(\frac{25}{2}\)
y = – \(\frac{3}{4}\)x + \(\frac{25}{2}\)
4y + 3x = 50
c = -22m – 4
= -22(\(\frac{7}{24}\)) – 4
= \(\frac{-77-48}{12}\) =\(\frac{-125}{12}\)
y = \(\frac{7}{24}\) x – \(\frac{125}{12}\)
⇒ 24y = 7x – 250
⇒ 7x – 24y – 250 = 0

Question 24.
Find the transverse common tangents of the circles x² + y² – 4x – 10y + 28 = 0 and x² + y² + 4x-6y + 4 = 0 [A.P. Mar. 15; Mar. 14]
Solution:
C₁ =(2, 5), C₂ = (-2, 3)
r₁ = \(\sqrt{4+25-28}\) = 1,
r₂ = \(\sqrt{4+9-4}\) = 3
r₁ + r₂= 4
C₁C₂ = \(\sqrt{(2+2)^{2}+(5-3)^{2}}\)
= \(\sqrt{16+4}\) = \(\sqrt{20}\)
‘C’ divides C₁C₂ in the ratio 5 : 3

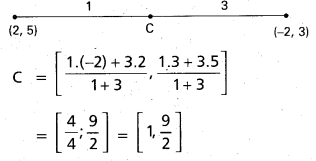
Equation of the pair transverse of the common tangents is
S₁² = SS₁₁
(x . 1 + \(\frac{9}{2}\)y – 2(x + 1) – 5(y + \(\frac{9}{2}\)) + 28)² = [1 + \(\frac{81}{4}\) – 4 – 10 × \(\frac{9}{2}\) + 28]
= – (x² + y² – 4x – 10y + 28)
⇒ (-x – \(\frac{1}{2}\)y + \(\frac{7}{2}\))²
= \(\frac{1}{4}\) (x² + y² – 4x – 10y + 28)
(-2x – y + 7)² = (x² + y² – 4x – 10y + 28)
4x² + y² + 4xy – 28x – 14y + 49 = x² + y² – 4x – 10y + 28
3x² + 4xy – 24x – 4y + 21 = 0
(3x + 4y – 21); (x – 1) = 0
3x + 4y – 21 = 0; x – 1 = 0

Question 25.
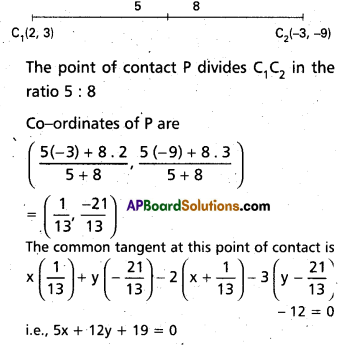
Show that the circles x² + y² – 4x – 6y – 12 = 0 and x² + y² + 6x + 18y + 26 = 0 touch each other. Also find the point of contact and common tangent at this point of contact. [Mar. 13]
Solution:
Equations of the circles are
x² + y² – 4x – 6y – 12 = 0 and x² + y² + 6x + 18y + 26 = 0
Centres are C₁(2, 3), C₂ = (-3, -9)
r₁ = \(\sqrt{4+9+12}\) = 5
r₂ = \(\sqrt{9+81-26}\) = 8
C₁C₂ = \(\sqrt{(2+3)^{2}+(3+9)^{2}}\)
= \(\sqrt{25+144}\) = 13 = r₁ + r₂
∴ Circle touch externally .
Equation of common tangent is S₁ – S₂ = 0
-10x -,24y – .38 = 0
5x + 12y + 19 = 0

Question 26.
Find the equation of circle with centre (1, 4) and radius ‘5’.
Solution:
Here (h, k) = (1, 4) and r = 5.
∴ By the equation of the circle with centre at C (h, k) and radius r is
(x – h)² + (y – k)² = r²
(x – 1)² + (y – 4)² = 5²
i.e., x² + y²– 2x – 8y – 8 = 0

Question 27.
Find the centre and radius of the circle x² + y² + 2x – 4y – 4 = 0.
Solution:
2g = 2, 2f = -4, c = -4
g = 1, f = -2, c = -4
Centre (-g, -f) = (-1, 2)
radius = \(\sqrt{g^{2}+f^{2}-c}\) = \(\sqrt{1+4-(-4)}\) = 3

Question 28.
Find the centre and radius of the circle 3x² + 3y² – 6x + 4y – 4 = 0.
Solution:
Given equation is
3x² + 3y² – 6x + 4y – 4 = 0
Dividing with 3, we have
x² + y² – 2x + \(\frac{4}{3}\) y – \(\frac{4}{3}\) = 0
2g = -2, 2f = \(\frac{4}{3}\), c = –\(\frac{4}{3}\)
g = -1, f = \(\frac{2}{3}\), c = –\(\frac{4}{3}\)
Centre (-g, -f) = (1, \(\frac{-2}{3}\))
radius = \(\sqrt{g^{2}+f^{2}-c}\) = \(\sqrt{1+\frac{4}{9}+\frac{4}{3}}\)
= \(\sqrt{\frac{9+4+12}{9}}\) = \(\sqrt{\frac{25}{9}}\) = \(\frac{5}{3}\)

Question 29.
Find the equation of the circle whose centre is (-1, 2) and which passes through (5, 6).
Solution:
Let C(-1, 2) be the centre of the circle

Since P(5,6) is a point on the circle CP = r
CP² = r² ⇒ r² = (-1 – 5)² + (2 – 6)²
= 36 + 16 = 52
Equation of the circle is (x + 1)² + (y – 2)²
= 52
x² + 2x + 1 + y² – 4y + 4 – 52 = 0
x² + y² + 2x – 4y – 47 = 0

Question 30.
Find the equation of the circle passing through (2, 3) and concentric with the circle x² + y² + 8x + 12y + 15 = 0.
Solution:
The required circle is concentric with the circle x² + y² + 8x + 12y + 15 = 0
∴ The equation of the required circle can be taken as
x² + y² + 8x + 12y + c = 0

This circle passes through P(2, 3)
∴ 4 + 9 + 16 + 36 + c = 0
c = – 65
Equation of the required circle is x² + y² + 8x + 12y – 65 = 0

Question 31.
From the point A(0, 3) on the circle x² + 4x + (y – 3)² = 0 a chord AB is drawn and extended to a point M such that AM = 2 AB. Find the equation of the locus of M.
Solution:
Let M = (x’, y’)
Given that AM = 2AB

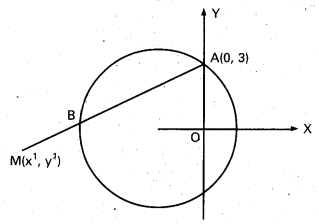
AB + BM = AB + AB
⇒ BM = AB
B is the mid point of AM
Co- ordinates of B are \(\left(\frac{x^{\prime}}{2}-\frac{y^{\prime}+3}{2}\right)\)
B is a point on the circle
(\(\frac{x^{\prime}}{2}\))² + 4(\(\frac{x^{\prime}}{2}\)) + (\(\frac{y^{\prime}+3}{2}\) – 3)² = 0
\(\frac{x^{\prime 2}}{4}\) + 2x’ + \(\frac{y^{\prime 2}-6 y^{\prime}+9}{4}\) = 0
x’² + 8x’ + y’² – 6y’ + 9 = 0
Lotus of M(x’, y’) is x² + y² + 8x – 6y + 9 = 0, which is a circle.

Question 32.
If the circle x² + y² + ax + by – 12 = 0 has the centre at (2, 3) then find a, b and the radius of the circle.
Solution:
Equation of the circle is
x² + y² + ax + by – 12 = 0
Centre = (\(-\frac{a}{2}\), \(-\frac{b}{2}\)) = (2, 3)
\(-\frac{a}{2}\) = 2, \(-\frac{b}{2}\) = 3
a = – 4, b – -6
g = -2, f = -3, c = -12
radius = \(\sqrt{g^{2}+f^{2}-c}\) = \(\sqrt{4+9+12}\) = 5

Question 33.
If the circle x² + y² – 4x + 6y + a = 0 has radius 4 then find a. [A.P. Mar. 16]
Solution:
Equation of the circle is x² + y² – 4x + 6y + a = 0
2g = – 4, 2f = 6, c = a
g =-2, f = 3, c = a
radius = 4 ⇒ \(\sqrt{g^{2}+f^{2}-c}\) = 4
\(\sqrt{4+9-a}\) = 4
13 – a = 16
a = 13 – 16 = -3

Question 34.
Find the equation of the circle passing through (4, 1), (6, 5) and having the centre on the line 4x + y – 16 = 0.
Solution:
Let the equation of the required circle be x² + y² + 2gx + 2fy + c = 0 .
This circle passes through A(4, 1)
16 + 1+ 8g + 2f + c = 0
8g + 2f + c = -17 ………………. (1)
The circle passes through B(6, 5)
36 + 25 + 12g + 10f + c = 0
12g + 10f + c = -61 ……………… (2)
The centre (-g, -f) lies on 4x + y – 16 = 0
– 4g – f – 16 = 0
4g + f + 16 = 0 ……………….. (3)
(2) – (1) gives 4g + 8f = -44 ……………….. (4)
4g + f = -16 …………….. (3)
7f = -28
f = \(\frac{-28}{7}\) = -4
From(3) 4g – 4 = -16
4g = -12 ⇒ g = -3
From(1) 8(-3) + 2(-4) + c = -17
c = -17 + 24 + 8 = 15
Equation of the required circle is
x² + y² – 6x – 8y + 15 = 0

Question 35.
Suppose a point (x₁, y₁) satisfies x² + y²+ 2gx + 2fy + c = 0 then show that it represents a circle whenever g, f and c are real.
Solution:
Comparing with the general equation of second degree co-efficient of x² = coefficient of y² and coefficient of xy = 0
The given equation represents a circle if g² + f² – c ≥ 0
(x₁, y₁) is a point on the given equation
x² + y² + 2gx + 2fy + c = 0, we have
x₁² + y₁² + 2gx₁ + 2fy₁ + c = 0
g² + f² – c = g² + f² + x₁² + y₁² + 2gx₁ + 2fy₁ = 0
= (x₁ + g)² + (y₁ + f)² ≥ 0
g, f and c are real
∴ The given equation represents a circle.

Question 36.
Find the equation of the circle whose extremities of a diameter are (1, 2) and (4, 5).
Solution:
Here (x₁, y₁) = (1, 2) and (x₂, y₂) = (4, 5)
Equation of the required circle is
(x – 1) (x – 4) + (y – 2) ( y – 5) = 0
x² – 5x + 4 + y² – 7y + 10 = 0
x² + y² – 5x – 7y + 14 = 0

Question 37.
Find the other end of the diameter of the circle x² + y² – 8x – 8y + 27 = 0 if one end of it is (2, 3).
Solution:
A = (2, 3) and AB is the diameter of the circle x² + y² – 8x – 8y + 27 = 0

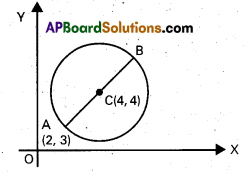
Centre of the circle is C = (4, 4)
Suppose B(x, y) is the other end
C = mid point of AB = \(\left(\frac{2+x}{2}, \frac{3+y}{2}\right)\) = (4, 4)
\(\frac{2+x}{2}\) = 4
2 + x = 8
x = 6
\(\frac{3+y}{2}\) = 4
3 + y = 8
y = 5
The other end of the diameter is B(6, 5)

Question 38.
Find the equation of the circum – circle of the traingle formed by the line ax + by + c = 0 (abc ≠ 0) and the co-ordinate axes.
Solution:
Let the line ax + by + c = 0 cut X, Y axes at A and B respectively co-ordinates of O are (0, 0) A are

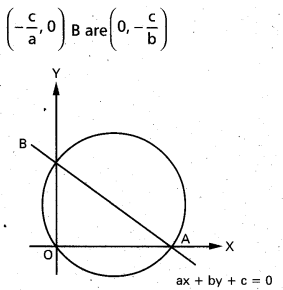
Suppose the equation of the required circle is x² + y² + 2gx + 2fy + c = 0
This circle passes through 0(0, 0)
∴ c = 0
This circle passes through A(-\(\frac{c}{a}\), 0)
\(\frac{c^{2}}{a^{2}}\) + 0 – 2\(\frac{\mathrm{gc}}{\mathrm{a}}\) = 0
2g . \(\frac{c}{a}\) = \(\frac{c^{2}}{a^{2}}\) ⇒ 2g = \(\frac{c}{a}\) ⇒ g = \(\frac{c}{2a}\)
The circle passes through B (0, –\(\frac{c}{b}\))
0 + \(\frac{c^{2}}{b^{2}}\) + 0 – 2g \(\frac{c}{b}\) = 0
2f\(\frac{c}{b}\) = \(\frac{c^{2}}{b^{2}}\) ⇒ 2g = \(\frac{c}{b}\) ⇒ f = \(\frac{c}{2b}\)
Equation of the circle, through O, A, B is
x² + y² + \(\frac{c}{a}\) x + \(\frac{c}{b}\) y = 0
ab(x² + y²) + (bx + ay) = 0
This is the equation of the circum circle of ∆OAB

Question 39.
Find the equation of the circle which passes through the vertices of the triangle formed by L₁ = x + y + 1 = 0; L₂ = 3x + y- 5 = 0 and L₃ = 2x + y – 5 = 0.
Solution:
Suppose L₁, L₂,: L₂, L₃ and L₃, L₁ intersect in A, B and C respectively.
Consider a curve whose equation is
k (x + y + 1) (3x + y – 5) + l(3x + y – 5) (2x + y – 5) + m(2x + y – 5) (x + y + 1) = 0 ………………. (1)
This equation represents a circle
i) Co-efficient of x² = Co – efficient of y²
3k + 6l + 2m = k + l + m
2k + 5l + m = 0 ……………….. (2)
ii) Co-efficient of xy = 0
4k + 5l + 3m = 0 ……………….. (3).
Applying cross multiplication rule for (2) and (3) we get

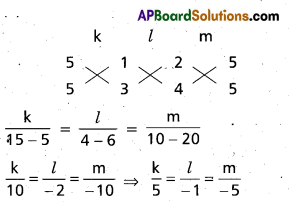
Substituting in (1), equation of the required circle is
5(x + y + 1) (3x + y – 5) – 1 (3x + y – 5)
(2x + y – 5) – 5(2x + y – 5) (x + y + 1) = 0
i.e., x² + y² – 30x – 10y + 25 = 0

Question 40.
Find the centre of the circle passing through the points (0, 0), (2, 0)and (0, 2).
Solution:
Here (x₁, y₁) = (0, 0); (x₂, y₁) = (2, 0);
(x₃, y₃) = (0, 2)
c₁ = -(x₁² + y₁²) = 0
c₂ = – (x₂² + y₂²) = -(2² + 0²) = -4
c₃ = -(x₃² + y₃²) = -(0² + 2²) – 4
The centre of the circle passing through three non-collinear points P(x₁, y₁), Q(x₂, y₂) and R(x₃, y₃)

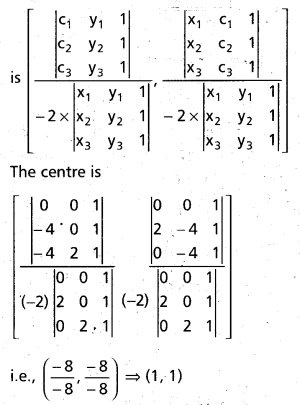
Thus the centre of the required circle is (1, 1)

Question 41.
Obtain the parametric equations of the circle x² + y² = 1.
Solution:
Equation of the circle is x² + y² = 1
Centre is (0, 0) radius = r = T

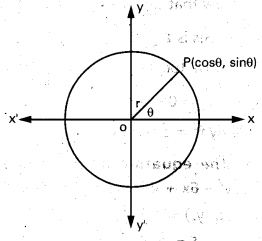
The circle having radius r is x = r cos θ,
y = sin θ where 0 < θ < 2π
The parametric equation of the circle
x² + y² = 1 and
x = 1 . cos θ = cos θ
y = 1 . sin θ = sin θ, θ < θ < 2π
Note: Every point on the circle can be expressed as (cos θ, sin θ)

Question 42.
Obtain the parametric equation of the circle represented by
x² + y² + 6x + 8y – 96 = 0.
Solution:
Centre (h, k) of the circle is (-3, -4)
radius = r = \(\sqrt{9+16+96}\) = \(\sqrt{121}\) = 11
Parametric equations are
x = h + r cos θ = -3 + 11 cos θ
y = k + r sin θ = -4 + 11 sin θ
where 0 < θ < 2π

Question 43.
Locate the position of the point (2, 4) with respect to the circle. x² + y² – 4x – 6y + 11 = 0.
Solution:
Here (x₁, y₁) = (2, 4) and
S ≡ x² + y² – 4x – 6y + 11
S₁₁ = 4 + 16 – 8 – 24 + 11
= 31 – 32 = – 1 < 0
∴ The point (2, 4) lies inside the circle S = 0

Question 44.
Find the length of the tangent from (1, 3) to the circle x² + y² – 2x + 4y – 11 = 0.
Solution:
Here (x₁, y₁) = (1, 3) and
S = x² + y² – 2x + 4y – 11 = 0
P(x₁, y₁) to S = 0 is \(\sqrt{S_{11}}\)
Length of the tangent = \(\sqrt{S_{11}}\)
= \(\sqrt{1+9-2+12-11}\) = \(\sqrt{9}\) = 3

Question 45.
If a point P is moving such that the length of tangents drawn from P to
x² + y² – 2x + 4y – 20 = 0 ……………… (1)
and x² + y² – 2x – 8y + 1 = 0 ……………….. (2)
are in the ratio 2 : 1.
Then show that the equation of the locus of P is x² + y² – 2x – 12y + 8 = 0.
Solution:
Let P(x₁, y₁) be any point on the locus and \(\overline{\mathrm{PT}_{1}}\), \(\overline{\mathrm{PT}_{2}}\) be the lengths of tangents from P to the circles (1) and (2) respectively.
x² + y² – 2x + 4y – 20 = 0 and
x² + y² – 2x – 8y + 1 = 0
\(\frac{\overline{\mathrm{PT}_{1}}}{\overline{\mathrm{PT}_{2}}}=\frac{2}{1}\)
i.e., \(\sqrt{x_{1}^{2}+y_{1}^{2}-2 x_{1}+4 y_{1}-20}\)
= \(2 \sqrt{x_{1}^{2}+y_{1}^{2}-2 x_{1}-8 y_{1}+1}\)
3 (x₁² + y₁²) – 6x₁ – 36y₁ + 24 = 0
Locus of P (x₁, y₁) is
x² + y² – 2x – 12y + 8 = 0

Question 46.
If S ≡ x² + y² + 2gx + 2fy + c = 0. represents a circle then show that the straight line lx + my + n = 0
i) touches the circle S = 0 if
(g² + f² – c) = \(\frac{(g l+m f-n)^{2}}{\left(l^{2}+m^{2}\right)}\)

ii) meets the circle S = 0 in two points if
g² + f² – c > \(\frac{(g l+m f-n)^{2}}{\left(l^{2}+m^{2}\right)}\)

iii) will not meet the circle if
g² + f² – c < \(\frac{(g l+m f-n)^{2}}{\left(l^{2}+m^{2}\right)}\)
Solution:
Let ‘c’ be the centre and ‘r’ be the radius of the circle S = 0
Then C = (-g, -f) and r = \(\sqrt{g^{2}+f^{2}-c}\)
i) The given straight line touches the circle
if r = \(\frac{|l(-\mathrm{g})+\mathrm{m}(-\mathrm{f})-\mathrm{n}|}{\sqrt{l^{2}+\mathrm{m}^{2}}}\)
\(\sqrt{g^{2}+f^{2}-c}\) = \(\frac{|-(l g+m f-n)|}{\sqrt{l^{2}+m^{2}}}\)
squaring on both sides, we get
g² + f² – c = \(\frac{(g l+m f-n)^{2}}{\left(l^{2}+m^{2}\right)}\)

ii) The given line lx + my + n = 0 meets the circle s = 0 in two points if
g² + f² – c > \(\frac{(g l+m f-n)^{2}}{\left(l^{2}+m^{2}\right)}\)

iii) The given line lx + my + n = 0 will not meet the circle s = 0 if
g² + f² – c < \(\frac{(g l+m f-n)^{2}}{\left(l^{2}+m^{2}\right)}\)

Question 47.
Find the length of the chord intercepted by the circle x² + y² + 8x – 4y – 16 = 0 on the line 3x – y + 4 = 0.
Solution:
The centre of the given circle c = (-4, 2) and radius r = \(\sqrt{16+4+16}\) = 6. Let the perpendicular distance from the centre to the line 3x-y + 4 = 0 be ‘d’ then
d = \(\frac{|3(-4)-2+4|}{\sqrt{3^{2}+(-1)^{2}}}\) = \(\frac{10}{\sqrt{10}}\) = \(\sqrt{10}\)
Length of the chord + \(\sqrt{r^{2}-d^{2}}\)
= 2\(\sqrt{6^{2}-(\sqrt{10})^{2}}\) = 2\(\sqrt{26}\)

Question 48.
Find the equation of tangents to x² + y² – 4x + 6y – 12 = 0 which are parallel to x + 2y- 8 = 0.
Solution:
Here g = -2, f = 3, r = \(\sqrt{4+9+12}\) = 5
and the slope of the required tangent is \(\frac{-1}{2}\)
The equations of tangents are
y + 3 = \(\frac{-1}{2}\) (x – 2) ± 5 \(\sqrt{1+\frac{1}{4}}\)
2(y + 3) = – x + 2 ± 5\(\sqrt{5}\)
x + 2y + (4 ± 5\(\sqrt{5}\)) = 0

Question 49.
Show that the circle S ≡ x² + y² + 2gx + 2fy + c = 0 touches the
i) X – axis if g² = c
ii) Y – axis if f² = c.
Solution:
i) We know that the intercept made by S = 0 on X – axis is 2\(\sqrt{g^{2}-c}\)
If the circle touches the X – axis then
22\(\sqrt{g^{2}-c}\) 0 ⇒ g² = c

Question 50.
Find the equation of the tangent to x² + y² – 6x + 4y – 12 = 0 at (- 1, 1).
Solution:
Here (x₁, y₁) = (-1, 1) and
S ≡ x² + y² – 6x + 4y – 12 = 0
∴ The equation of the tangent is
x(-1) + y (1) – 3(x – 1) + 2(y + 1)- 12 = 0
The equation of the tangent at the point P(₁, y₁) to the circle
S ≡ x² + y² + 2gx + 2fy + c = 0 is S₁ = 0
⇒ – x + y – 3x + 3 + 2y + 2 – 12 = 0
⇒ – 4x + 3y – 7 = 0
(or) 4x – 3y + 7 = 0

Question 51.
Find the equation of the tangent to x² + y² – 2x + 4y = 0 at (3, -1). Also find the equation of tangent parallel to it.
Solution:
Here (x₁, y₁) = (3, -1) and
S ≡ x² + y² – 2x + 4y = 0
The equation of the tangent at (3, -1) is
x (3) + y (-1) – (x + 3) + 2(y – 1) = 0
3x – y – x – 3 + 2y – 2 = 0
2x + y – 5 = 0
Slope of the tangent is m = -2, for the circle
g = -1, f = 2, c = 0
r = \(\sqrt{1+4-0}\) = \(\sqrt{5}\)
Equations of the tangents are
y = mx ± r \(\sqrt{1+m^{2}}\) is a tangent to the circle x² + y² = r² and the slope of the tangent is m.
y + 2 = -2(x – 1) ± \(\sqrt{5} \sqrt{1+4}\)
y + 2 = -2x + 2 ± 5
2x 4- y = ± 5
The tangents are
2x + y + 5 = 0 and 2x + y – 5 = 0
The tangent parallel to the given tangent is 2x + y ± 5 = 0

Question 52.
If 4x – 3y + 7 = 0 is a tangent to the circle represented by x² + y² – 6x + 4y – 12 = 0, then find its point of contact.
Solution:
Let (x₁, y₁) be the point of contact
Equation of the tangent is
(x₁ + g) x + (y₁ + f) y + (gx₁ + fy₁ + c) = 0
We have \(\frac{x_{1}-3}{4}\) = \(\frac{y_{1}+2}{-3}\)
= \(\left(\frac{-3 x_{1}+2 y_{1}-12}{7}\right)\) ……………… (1)
From first and second equalities of (1), we get
3x₁ + 4y₁ = 1 ………………. (2)
Now by taking first and third equalities of (1), we get
19x₁ – 8y₁ = -27 ………………. (3)
Solving (2) and (3) we obtain
x₁ = -1;, y₁ = 1
Hence the point of contact is (-1, 1).

Question 53.
Find the equations of circles which touch 2x – 3y + 1 = 0 at (1, 1) and having radius \(\sqrt{13}\).
Solution:
The centres of the required circle lies on a line perpendicular to 2x – 3y + 1 =0 and passing through (1, 1)

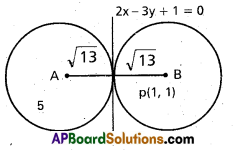
The equation of the line of centre can be taken as
3x + 2y + k = 0
This line passes through (1, 1)
3 + 2 + k = 0 ⇒ k = -5
Equation of AB is 3x + 2y – 5 = 0
The centres A and B are situated on
3x + 2y – 5 = 0 at a distance \(\sqrt{13}\) from (1, 1).
The centres are given by
(x₁ ± r cos θ, y₁ ± r sin θ)
\(\left(1+\sqrt{13}\left(-\frac{2}{\sqrt{13}}\right) 1+\sqrt{13} \cdot \frac{3}{\sqrt{13}}\right)\) and
\(\left(1-\sqrt{13} \frac{(-2)}{\sqrt{13}}, 1-\sqrt{13} \cdot \frac{3}{\sqrt{13}}\right)\)
i.e., (1 -2, 1 +3) and (1 + 2, 1 – 3)
(-1, 4) and (3, -2)
Centre (3, -2), r = \(\sqrt{13}\)
Equation of the required circles are
(x + 1 )² + (y – 4)² = 13 and
(x – 3)² + (y + 2)² = 13
i.e., x² + y² + 2x – 8y + 4 = 0
and x² + y² – 6x + 4y = 0

Question 54.
Show that the line 5x + 12y – 4 = 0 touches the circle x² + y² – 6x + 4y + 12 = 0.
Solution:
Equation of the circle is
x² + y² – 6x + 4y + 12 = 0
Centre (3, -2), r = \(\sqrt{9+4-12}\) = 1 ……………….. (1)
The given line touches the circle if the perpendicular distance from the centre on the given line is equal to radius of the circle, d = perpendicular distance from (3, -2)
= \(\frac{|5(3)+12(-2)-4|}{\sqrt{25+144}}\)
= \(\frac{13}{13}\) = 1 = radius of the circle ………………… (2)
∴ The given line 5x + 12y – 4 = 0 touches the circle.

Question 55.
If the parametric values of two points A and B lying on the circle x² + y² – 6x + 4y – 12 = 0 are 30° and 60° respectively, then find the equation of the chord joining A and B.
Solution:
Here g = -3, f = -2; r = \(\sqrt{9+4-12}\) = 5
∴ The equation of the chord joining the points θ₁ = 30°, θ₂ = 60°
Equation of chord joining the point; (-g+ r cos θ₁(-f + r sin θ₁) where r is the radius of the circle; θ₂ and (-g + r cos θ₂, -f + r sin θ₂) is (x + g) cos (\(\frac{\theta_{1}+\theta_{2}}{2}\)) + (y + f)
sin (\(\frac{\theta_{1}+\theta_{2}}{2}\)) = r cos (\(\frac{\theta_{1}+\theta_{2}}{2}\))
(\(\frac{\theta_{1}+\theta_{2}}{2}\)) = r cos \(\frac{\theta_{1}+\theta_{2}}{2}\)
(x – 3) cos [latex]\frac{60^{\circ}+30^{\circ}}{2}[/latex]
(y + 2) sin [latex]\frac{60^{\circ}+30^{\circ}}{2}[/latex]
= 5 cos [latex]\frac{60^{\circ}-30^{\circ}}{2}[/latex]
i.e., (x – 3) cos 45 ° + (y + 2) sin 45°
= 5 cos 15°
= \(\frac{(x-3)+(y+2)}{\sqrt{2}}=5\left[\frac{(\sqrt{3}+1)}{2 \sqrt{2}}\right]\)
i.e., 2x + 2y – (7 + 5\(\sqrt{3}\)) = 0.

Question 56.
Find the equation of the tangent at the point 30° (parametric value of θ) of the circle is x² + y² + 4x + 6y – 39 = 0.
Solution:
Equation of the circle is
x² + y² + 4x + 6y – 39 = 0
g = 2,f = 3, r = \(\sqrt{4+9+39}\) = \(\sqrt{52}\) = 2\(\sqrt{3}\)
θ = 30°
Equation of the tangent is
(x + g) cos 30° + (y + f) sin 30° = r
(x + 2) \(\frac{\sqrt{3}}{2}\) +(y + 3) \(\frac{1}{2}\) = 2713
\(\sqrt{3}\)x + 2\(\sqrt{3}\) + y + 3 = 4\(\sqrt{13}\)
\(\sqrt{3}\) x + y + (3 + 2\(\sqrt{3}\) – 4\(\sqrt{13}\)) = 0

Question 57.
Find the area of the triangle formed by the tangent at P(x₁, y₁) to the circle x² + y² = a² with the co-ordinate axes where x₁y₁ ≠ 0.
Solution:
Equation of the circle is x² + y² = a²
Equation of the tangent at P(x₁, y₁) is xx₁ + yy₁ = a² ……………… (1)

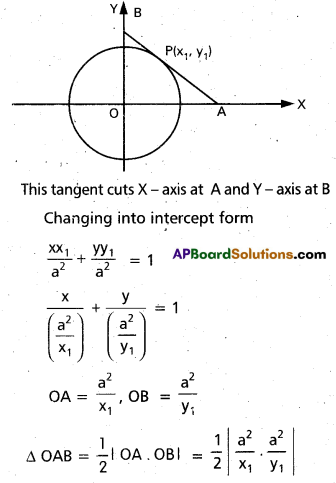
= \(\frac{a^{4}}{2\left|x_{1} y_{1}\right|}\)

Question 58.
Find the equation of the normal to the circle x² + y² – 4x – 6y + 11 = 0 at (3, 2). Also find the other point where the normal meets the circle.
Solution:
Let A(3, 2), C be the centre of given circle and the normal at A meet the circle at B(a, b). From the hypothesis, we have
2g = -4 i.e., g = -2
2f = -6 i.e., f = -3
x₁ = 3 and y₁ = 2
The equation of the normal at P(x₁, y₁) of the circle
S ≡ x² + y² + 2gx + 2fy + c = Q is
(x – x₁) (y₁ + f) – (y – y₁) (x₁ + g) = 0
The equation of normal at A(3, 2) is
(x – 3) (2 – 3) – (y – 2) (3 – 2) = 0
i.e., x + y – 5 = 0
The centre of the circle is the mid point of A and B. (Points of intersection of normal and circle).
\(\frac{a+3}{2}\) = 2 ⇒ a = 1
and \(\frac{b+2}{2}\) = 3 ⇒ b = 4
Hence the normal at (3, 2) meets the circle at (1, 4).

Question 59.
Find the area of the triangle formed by the normal at (3, -4) to the circle x² + y² – 22x – 4y + 25 = 0 with the co-ordinate axes.
Solution:
Here 2g = -22, 2f = – 4, g = -11, f = -2
x₁ = 3, y₁ = – 4
Equation of the normal at (3, -4) is
(x – x₁) (y₁ + f) – (y – y₁) (x₁ + g) = 0
(x – 3) (-4 – 2) – (y + 4) (3 – 11) = 0
3x + 4y – 25 = 0 ……………….. (1)
This line meets X-axis at A(\(\frac{25}{3}\), 0) and Y – axis at B(0, \(\frac{25}{4}\)) , ∆OAB = \(\frac{1}{2}\) |OA . OB|
= \(\frac{1}{2}\) |\(\frac{25}{3} \times-\left[\frac{25}{4}\right]\)| = \(\frac{625}{24}\)

Question 60.
Show that the line lx + my + n =0 is a normal to the circle S = 0 if and only if gl + mf = n.
Solution:
The straight line lx + my + n = 0 is normal to the circle
S = x² + y² + 2gx + 2fy + c = 0
⇒ If the centre (-g, -f) lies on
lx + my + n = 0
l (- g) + m(- f) + n = 0
gl + fm = n

Question 61.
Find the condition that the tangents drawn from the exterior point (g, f) to S ≡ x² + y² + 2gx + 2fy + c = 0 are perpendicular to each other.
Solution:
If the angle between the tangents drawn from P(x₁, y₁) to S = 0 is θ, then
tan (\(\frac{\theta}{2}\)) = \(\frac{\mathrm{r}}{\sqrt{\mathrm{S}_{11}}}\)
Equation of the circle is
x² + y² + 2gx + 2fy + c = 0
r = \(\sqrt{g^{2}+f^{2}-c}\)
S₁₁ = g² + f² + 2g² + 2f² + c
= 3g² + 3f² + c
θ = 90° ⇒ tan \(\frac{\theta}{2}\) = tan 45 = \(\frac{\sqrt{g^{2}+f^{2}-c}}{\sqrt{3 g^{2}+3 f^{2}+c}}\)
1 = \(\frac{g^{2}+f^{2}-c}{3 g^{2}+3 f^{2}+c}\)
⇒ 3g² + 3f² + c = g² + f² – c
⇒ 2g² + 2f² + 2c = 0 ⇒ g² + f² + c = 0
This is the condition for the tangent drawn from (g, f) to the circle S = 0 are perpendicular.
Note : Here c < 0

Question 62.
If θ₁, θ₂ are the angles of inclination of tangents through a point P to the circle x² + y² = a² then find the locus of P when cot θ₁ + cot θ₂ = k.
Solution:
Equation of the circle is x² + y² = a²
If m is the slope of the tangent, then the equation of tangent passing through P(x₁, y₁) can be taken as
y₁ = mx₁ ± a\(\sqrt{1+m^{2}}\)
(y₁ – mx₁)² = a² (1 + m²)
m²x₁² + y₁² – 2mx₁y₁ – a² – a²m² = 0
m² (x₁² – a²) – 2mx₁y₁ + (y₁² – a²) = 0
Suppose m₁ and m₂ are the roots of this equation
m₁ + m₂ = tan θ₁ + tan θ₂ = \(\frac{2 x_{1} y_{1}}{x_{1}^{2}-a^{2}}\)
m₁m₂ – tan θ₁ . tan θ₁ = \(\frac{y_{1}^{2}-a^{2}}{x_{1}^{2}-a^{2}}\)
Given that cot θ₁ + cot θ₂ = k
⇒ \(\frac{1}{\tan \theta_{1}}+\frac{1}{\tan \theta_{2}}\) = k
⇒ \(\frac{\tan \theta_{1}+\tan \theta_{2}}{\tan \theta_{1} \cdot \tan \theta_{2}}\) = k
⇒ \(\frac{2 x_{1} y_{1}}{y_{1}^{2}-a^{2}}\) = k
2x₁y₁ = k (y₁² – a²)
Locus of P(x₁, y₁) is 2xy = k(y² – a²)
Also, conversely if P(x₁, y₁) satisfies the condition 2xy = k(y² – a²) then it can be show that cot θ₁ + cot θ₂ = k
Thus the locus of P is 2xy = k(y² – a²)

Question 63.
Find the chord of contact of (2, 5) with respect to the circle x² + y² – 5x + 4y – 2 = 0.
Solution:
Here (x₁, y₁) = (2, 5). By
S ≡ x² + y² + 2gx + 2fy + c = 0 then the equation of the chord of contact of P with respect to S = 0 is S₁ =0, the required chord of contact is
xx₁ + yy₁ – \(\frac{5}{2}\) (x + x₁) + 2(y + y₁) – 2 = 0
Substituting x₁ and y₁ values, we get
x(2) + y(5) – \(\frac{5}{2}\) (x + 2) + 2(y + 5) – 2 = 0
i.e., x – 14y + 6 = 0.

Question 64.
If the chord of contact of a point P with respect to the circle x² + y² = a² cut the circle at A and B such that, AÔB = 90° then show that P lies on the circle x² + y² = 2a².
Solution:
Given circle x² + y² = a² …………… (1)
Let P(x₁, y₁) be a point and let the chord of contact of it cut the circle in A and B such that AÔB = 90°. The equation of the chord of contact of P(x₁, y₁) with respect to (1) is
xx₁ + yy₁ – a² = 0 ……………………. (2)
The equation of the pair of the lines \(\overleftrightarrow{\mathrm{OA}}\) and \(\overleftrightarrow{\mathrm{OB}}\) is.given by x² + y² – a²
\(\left(\frac{x x_{1}+y y_{1}}{a^{2}}\right)^{2}\) = 0
or a² (x² + y²)- (xx₁ + yy₁)² = 0
or x² (a² – x₁²) – 2x₁y₁xy + y² (a² – y₁²) = 0 – (3)
Since AÔB = 90°, we have the coefficient of x² in (3) + coefficient of y² in (3) = 0
∴ a² – x₁² + a² – y₁² = 0
i.e., x₁² + y₁² = 2a²
Hence the point P(x₁, y₁) lies on the circle x² + y² = 2a².

Question 65.
Find the equation of the polar of (2, 3) with respect to the circle x² + y² + 6x + 8y – 96 = 0.
Solution:
Here (x₁, y₁) = (2, 3) ⇒ x₁ = 2, y₁ = 3
Equation of the circle is
x² + y² + 6x + 8y – 96 = 0
Equation of the polar is S₁ = 0
Polar of (2, 3) is x . 2 + y . 3 + 3(x + 2) + 4(y + 3) – 96 = 0
2x + 3y + 3x + 6 + 4y + 12 – 96 = 0
5x + 7y – 78 = 0

Question 66.
Find the pole of x + y + 2 = 0 with respect to the circle x² + y² – 4x + 6y – 12 = 0.
Solution:
Here lx + my + n = 0 is x + y + 2 = 0
l = 1, m = 1, n = 2
Equation of the circle is
S ≡ x² + y² – 4x + 6y – 12 = 0
2g = -4, 2f = 6, c = -12
g = -2, f = 3, c = -12

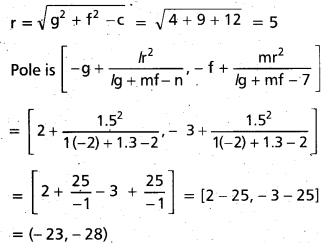
The pole of the given line x + y + 2 = 0 w.r.to the given circle (-23, -28)

Question 67.
Show that the poles of the tangents to the circle x² + y² = a² with respect to the circle (x + a)² + y² = 2a² lie on y² + 4ax = 0.
Solution:
Equations of the given circles are
x² + y² = a² …………………. (1)
and (x + a)² + y² = 2a² ………………. (2)
Let P(x₁, y₁) be the pole of the tangent to the circle (1) with respect to circle (2).
The polar of P w.r.to circle (2) is
xx₁ + yy₁ + a(x + x₁) – a² = 0
x(x₁ + a) + yy₁ + (ax₁ – a₂) = 0
This is a tangent to circle (1)
∴ a = \(\frac{\left|0 .+0+a x_{1}-a^{2}\right|}{\sqrt{\left(\dot{x}_{1}+a\right)^{2}}+y_{1}^{2}}\)
a = \(\frac{a\left|x_{1}-a\right|}{\sqrt{\left(x_{1}+a\right)^{2}}+y_{1}^{2}}\)
Squaring and cross – multiplying
(x₁ + a)² + y₁² = (x₁ – a)²
(or) y₁² + (x₁ + a)² – (x₁ – a)² = 0
i.e., y₁² + 4ax₁ = 0
The poles of the tangents to circle (1) w.r.to circle (2) lie on the curve y² + 4ax = 0

Question 68.
Show that (4, -2) and (3, -6) are conjugate with respect to the circle x² + y² – 24 = 0.
Solution:
Here (x₁, y₁) = (4, -2) and (x₂, y₂) = (3, -6) and
S ≡ x² + y² – 24 = 0
Two points (x₁, y₁) and (x₂, y₂) are conjugate with respect to the circle S = 0 if S₁₂ = 0;
In this case x₁x₂ + y₁y₂ – 24 = 0
S₁₂ = 4.3 + (-2) (-6) – 24 .
= 12 + 12 – 24 = 0
∴ The given points are conjugate with respect to the given circle.

Question 69.
If (4, k) and (2, 3) are conjugate points with respect to the circle x² + y² = 17 then find k.
Solution:
Here (x₁, y₁) = (4, k) and (x₂, y₂) = (2, 3) and
S ≡ x² + y² – 17 = 0
The given points are conjugate ⇒ S₁₂ = 0
x₁x₂ + y₁y₂ – 17 = 0
4.2 + k. 3 – 17 = 0
3k = 17 – 8 = 9
k = \(\frac{9}{3}\) = 3

Question 70.
Show that the lines 2x + 3y + 11 = 0 and 2x – 2y – 1 = 0 are conjugate with respect to the circle x² + y² + 4x + 6y – 12 = 0.
Solution:
Here l₁ = 2, m₁ = 3, n₁ = 11
l₂ = 2, m₂ = -2, n₂ = -1
and g = 2, f = 3, c = 12
r = \(\sqrt{9+4-12}\) = 1
We know that l₁x + m₁y + n₁ = 0
l₂x + m₂y + n₂ = 0 are conjugate with respect to S = 0
r² (l₁l₂ + m₁m₂) = (l₁g + m₁f – n₁) (l₂g + m₂f – n₂)
r² (l₁l₂ + m₁m₂) – 1(2.2 + 3(- 2)) = 4 – 6 = -2
(l₁g + m₁f – n₁) (l₂g + m₂f – n₂)
= (2.2 + 3.3-11) (2.2-2.3 + 11)
= 2(- 1) = -2 L.H.S. = R.H.S.
Condition for conjugate lines is satisfied
∴ Given lines are conjugate lines.

Question 71.
Show that the area of the triangle formed by the two tangents through P(xv to the circle S ≡ x² + y² + 2gx + 2fy + c = 0 and the chord of contact of P with respect to S = 0 is \(\frac{r\left(S_{11}\right)^{3 / 2}}{S_{11}+r^{2}}\) where r is the radius of the circle.
Solution:
PA and PB are two tangents from P to the circle S = 0
AB is the chord of contact

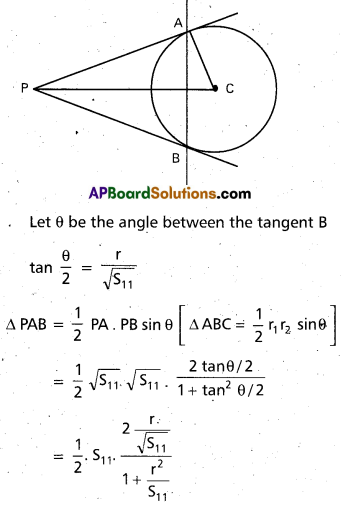
= \(S_{11} \cdot \frac{r}{\sqrt{S_{11}}} \cdot \frac{S_{11}}{S_{11}+r^{2}}\)
= \(\frac{r\left(S_{11}\right)^{3 / 2}}{S_{11}+r^{2}}\)

Question 72.
Find the mid point of the chord intercepted by
x² + y² – 2x – 10y + 1 = 0 ………………. (1)
on the line x – 2y + 7 = 0. ……………. (2)
Solution:
Let P(x₁, y₁) be the mid point of the chord AB
Equation of the chord is S₁ = S₁₁
xx₁ + yy₁ – 1 (x + x₁) – 5(y + y₁) + 1 = x₁² + y₁² – 2x₁ – 10y₁ + 1 .
x(x₁ – 1) + y(y₁ – 5) – (x₁² + y₁² – x₁ – 5y₁) = 0 ………………. (3)
Equation of the given line is x – 2y – 7 = 0
Comparing (1) and (2)
\(\frac{x_{1}-1}{1}=\frac{y_{1}-5}{-2}=\frac{x_{1}^{2}+y_{1}^{2}-x_{1}-5 y_{1}}{-7}\) = k (say)
x₁ – 1 = k ⇒ x₁ = k + 1
y₁ – 5 = -2(k) ⇒ y₁ = 5 – 2k
x₁² + y₁² – x₁ – 5y₁ = -7k
⇒ (k + 1)² + (5 – 2k)² – (k + 1) – 5(5 – 2k) + 7k = 0
⇒ k² + 2k + 1 + 25 + 4k² – 20k – k – 1 – 25 + 10k + 7k = 0
⇒ 5k² – 2k = 0
⇒ k (5k — 2) = 0 ⇒ k = 0, \(\frac{2}{5}\)
k = 0 ⇒ (x₁, y₁) = (1, 5) and x – 2y + 7
= 1 – 10 + 7 ≠ 0
(1, 5) is not a point on the chord.
k = \(\frac{2}{5}\) (x₁, y₁) = \(\left(\frac{7}{5}, \frac{21}{5}\right)\)

Question 73.
Find the locus of mid-points of the chords of contact of x² + y² = a² from the points lying on the line lx + my + n = 0.
Solution:
Let P = (x₁, y₁) be a point on the locus P is the mid point of the chord of the circle
x² + y² = a²
Equation of the chord is lx + my + n = 0 ……………… (1)
Equation of the circle is x² + y² = a²
Equation is the chord having (x₁, y₁) as mid point of S₁ = S₁₁
xx₁ + yy₁ = x₁² + y₁²
xx₁ + yy₁ – (x₁² + y₁²) = 0 ……………….. (2)
Pole of (2) with respect to the circle in

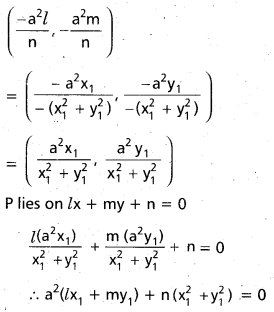
Locus of P(x₁, y₁) is n(x² + y²) + a²(lx + my) = 0

Question 74.
Show that the four common tangents can be drawn for the circles given by
x² + y² – 14x + 6y + 33 = 0 …………… (1)
and x² + y² + 30x – 2y +1 = 0 ……………… (2)
and find the internal and external centres of similitude. [T.S. Mar. 19]
Solution:
Equations of the circles are
x² + y² – 14x + 6y + 33 = 0
and x² + y² + 30x – 2y + 1 = 0
Centres are A(7, -3), B(-15, 1)
r₁ = \(\sqrt{49+9-33}\) = 5
r₂ = \(\sqrt{225+1-1}\) = 15
AB = \(\sqrt{(7+15)^{2}+(-3-1)^{2}}\)
= \(\sqrt{484+16}\) = \(\sqrt{500}\) > r₁ + r₂
∴ Four common tangents can be drawn to the given circle
r₁ : r₂ = 5 : 15 = 1 : 3

Internal centre of similitude S’ divides AB in the ratio 1 : 3 internally
Co-ordinates of S’ are
\(\left(\frac{1 .(-15)+3.7}{1+3}, \frac{1.1+3(-3)}{1+3}\right)\)
= \(\left(\frac{6}{4}, \frac{1-9}{4}\right)\) = \(\left(\frac{3}{2},-2\right)\)
External centre of similitude S divides AB externally in the ratio 1 : 3
Co-ordinates of S are
\(\left(\frac{1(-15)+3(7)}{1-3}, \frac{1.1-3(-3)}{1-3}\right)\)
= \(\left(\frac{-15-21}{-2}, \frac{1+9}{-2}\right)\) = (18, -5)

Question 75.
Prove that the circles x² + y² – 8x – 6y + 21 = 0 and x² + y² – 2y – 15 = 0 have exactly two common tangents. Also find the point of intersection of those tangents.
Solution:
Let C₁, C₂ be the centres and r₁, r₂ be their radii.
Equation of the circles are
x² + y² – 8x – 6y + 21 = 0
and x² + y² – 2y – 15 = 0
C₁(4, 3), C₂(0, 1)
r₁ = \(\sqrt{16+9-21}\) = 2, r₂ = \(\sqrt{1+15}\) = 4
\(\overline{\mathrm{C}_{1} \mathrm{C}_{2}^{2}}\) = (4 – 0)² + (3 – 1)² = 16 + 4 = 20
C₁C₂ = 2\(\sqrt{5}\)
|r₁ – r₂| = |2 – 4| = 2, r₁ + r₂ = 2 + 4 = 6
|r₁ – r₂| < C₁C₂ < r₁ + r₂
Given circles intersect and have exactly two common tangents.
r₁ : r₂ = 2 : 4 = 1 : 2
The tangents intersect in external centre of similitude

Co-ordinates of S are
\(\left(\frac{1.0-2.4}{1-2}, \frac{1.0-2.3}{1-2}\right)\) = \(\left(\frac{-8}{-1}, \frac{-5}{-1}\right)\)
= (8, 5)

Question 76.
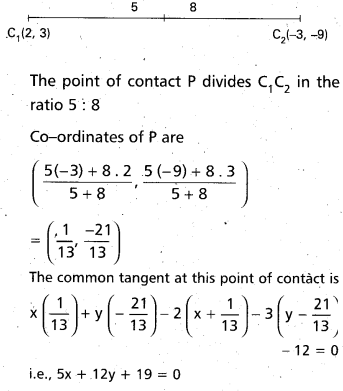
Show that the circles x² + y² – 4x – 6y – 12 = 0 and x² + y² + 6x + 18y + 26 = 0 touch each other. Also find the point of contact and common tangent at this point of contact. [Mar. 13]
Solution:
Equations of the circles are
x² + y² – 4x – 6y – 12 = 0 and x² + y² + 6x + 18y + 26 = 0
Centres are C₁(2, 3), C₂ = (-3, -9)
r₁ = \(\sqrt{4+9+12}\) = 5
r₂ = \(\sqrt{9+81-26}\) = 8
C₁C₂ = \(\sqrt{(2+3)^{2}+(3+9)^{2}}\)
= \(\sqrt{25+144}\) = 13 = r₁ + r₂
∴ Circle touch externally
Equation of common tangent is S₁ – S₂ = 0
-10x – 24y – 38 = 0
5x + 12y + 19 = 0

Question 77.
Show that the circles x² + y² – 4x – 6y – 12 = 0 and 5(x² + y²) – 8x – 14y – 32 = 0 touch each other and find their point of contact.
Solution:
Equations of the circles are
x² + y² – 4x – 6y – 12 = 0
and x² + y² – \(\frac{8}{5}\) x – \(\frac{14}{5}\) y – \(\frac{32}{4}\) = 0
Centres are A(2, 3), B(\(\frac{4}{5}\), \(\frac{7}{5}\))

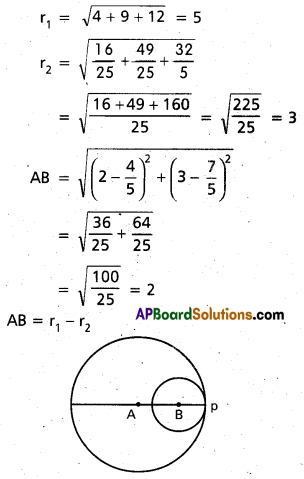
The circles touch internally
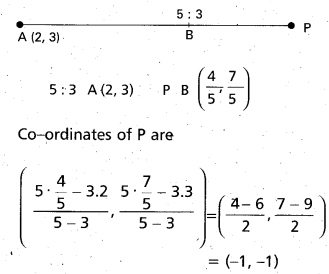
P divides AB externally the ratio 5 : 3

Question 78.
Find the equation of the pair of tangents from (10, 4) to the circle x² + y² = 25.
Solution:
(x₁, y₁) = (1o, 4)
Equation of the circle is x² + y² – 25 = 0
Equation of the pair of tangents is S₁² = S . S₁₁
(10x + 4y – 25)² = (100 + 16 – 25)(x² + y² – 25)
100x² + 16y² + 625 + 80xy – 500x – 200y = 91x² + 91y² – 2275
9x² + 80xy – 75y² – 500x – 200y + 2900 = 0

Question 79.
Find the equation to all possible common tangents of the circles x² + y² – 2x – 6y + 6 = 0 and x² + y² = 1.
Solution:
Equations of the circles are
x² + y² – 2x – 6y + 6 = 0
and x² + y² = 1
Centres are A(1, 3), B(0, 0),
r₁ = \(\sqrt{1+9-6}\) = 2
r₂ = 1
External centre of similitude S divides AB externally in the ratio 2 : 1
Co-ordinates of S are
\(\left(\frac{2.0-1.1}{2-1}, \frac{2.0-1.3}{2-1}\right)\) = (-1, -3)
Equation to the pair of direct common tangents are
(x² + y² – 1) (1 + 9 – 1) = (x + 3y + 1)²
This can be expressed as
(y – 1) (4y + 3x – 5) = 0
Equations of direct common tangents are
y – 1 = 0 and 3x + 4y – 5 = 0
Internal centre of S’ divides AB internally in the ratio 2 : 1
Co-ordinates of S’ are
\(\left(\frac{2.0+1.1}{2+1}=\frac{2.0+1.3}{2+1}\right)\left(\frac{1}{3}, 1\right)\)
Equation to the pair of transverse common tangents are
(\(\frac{x}{3}\) +y – 1)²
= (\(\frac{1}{9}\) + 1 – 1) (x² + y² – 1)
This can be expressed as
(x + 1)(4x – 3y – 5) = 0
Equation of transverse common tangents are x + 1 = 0 and 4x – 3y – 5 = 0