AP Board 7th Class Maths Solutions Chapter 5 Triangle and Its Properties InText Questions

AP State Syllabus AP Board 7th Class Maths Solutions Chapter 5 Triangle and Its Properties InText Questions and Answers.

AP State Syllabus 7th Class Maths Solutions 5th Lesson Triangle and Its Properties InText Questions

Try This

Question 1.
The lengths of two sides of a triangle are 6 cm and 9 cpi. Write all the possible lengths of the third side. (Page No. 92)
Solution:
The possible length of the third side must greater than (9— 6) and less than (9 + 6).
∴ The third side may be 4 cm, 5 cm, 6 cm, 7 cm, 8 cm, 9 cm, 10 cm, 11 cm, 12 cm, 13 cm, 14 cm.

Try This

Question 1.
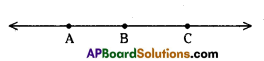
Uma felt that a triangle can be formed with three collinear points. Do you agree ? Why? Draw diagrams to justify your answer. (Page No. 88)
Solution:
Uma ¡s not correct.
A triangle cant be formed with three collinear points.

Do This

Question 1.
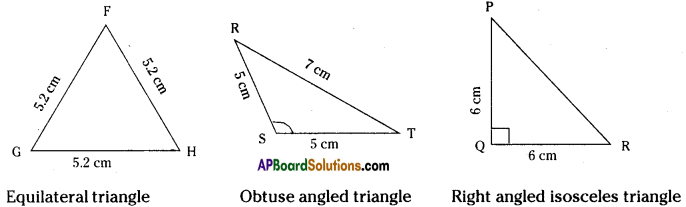
Classify the following triangles according to their (i) sides and (ii) angles. (Page No. 89)
Solution:

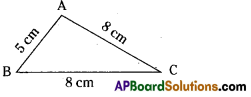
Two sides AC and BC are equal.
∴ ∆ABC is an isosceles triangle

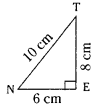
As ∠E = 90°;
∆NET is a right angled triangle.

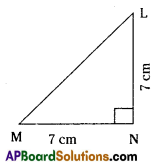
In ∆MNL,
MN = NL and ∠N = 90°
∆ MNL is right angled isosceless triangle.

Question 2.
Write the six elements (i.e., the 3 sides and 3 angles) of ∆ABC.
Solution:
The three sides \(\overline{\mathrm{AB}}, \overline{\mathrm{BC}}, \overline{\mathrm{CA}}\) and the three angles ∠A, ∠B and ∠C are the six elements of ∆ABC.

Question 3.
Write the side opposite to vertex Q in ∆PQR.
Solution:
The side opposite to the vertex Q is \(\text { PR }\) .

Question 4.
Write the angle opposite to side \(\text { LM }\) in ∆LMN.
Solution:
Angle opposite to side \(\text { LM }\) is ∠N.

Question 5.
Write the vertex opposite to side \(\text { RT }\) in ∆RST.
Solution:
Vertex opposite to \(\text { RT }\) is S.

Try This

Question 1.
Rashmi claims that no triangle can have more than one right angle. Do you agree with her. Why ?
Solution:
Yes, Rashmi is correct. A triangle can’t have more than one right angle. As the sum of two right angles is 180° there will be no scope for 3rd angle.

Question 2.
Kamal claims that no triangle can have more than two acute angles. Do you agree with him. Why?
Solution:
No. Kamal is not right. A triangle can have all the three acute angles.
(60°, 60°, 60°), (30°, 70°, 80°), (40°, 60°, 80°) etc ………….

Question 3 i)
Draw altitudes from P to \(\text { QR }\) for the following triangles. Also, draw altitudes from the other vertices.

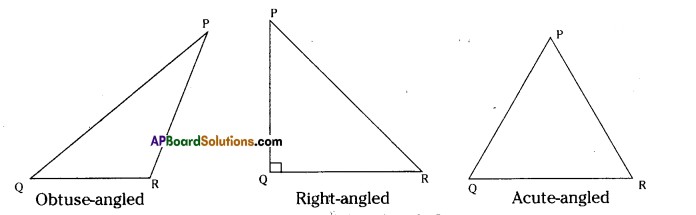
ii) Will an altitude always lie in the interior of a triangle ?
iii) Can you think of a triangle in which the two altitudes of a triangle are two of its sides ?
Solution:
i)

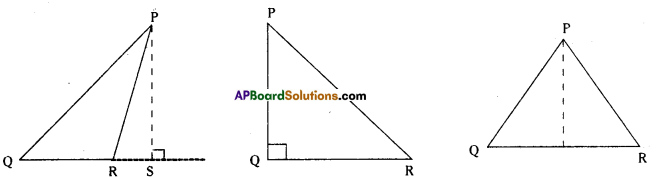
ii) An altitude need not necessarily be in the Interior of a triangle.
iii) Yes. In a right triangle the two sides containing the right angle are the two altitudes of it.

Do This

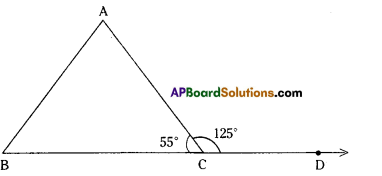
Question 1.
Draw ∆ABC and fofm an exterior ∠ACD. Now take a protractor and measure ∠ACD, ∠A and ∠B. Find the sum ∠A + ∠B and compare it with the measure ∠ACD. Do you observe that ZACD is equal (or nearly equal) to ∠A + ∠B ? (Page No. 102)
Solution:
For instance in the adjacent figure
∠A = 68° ; ∠B = 57°
∠A + ∠B = 68° + 57° = 125 ∠ACD = 125°
∴ ∠ACD = ∠A + ∠B

Question 2.
Copy each of the following triangles. In each case verify that an exterior angle of a triangle^ is equal to the sum of the two interior opposite angles. (Page No. 103)

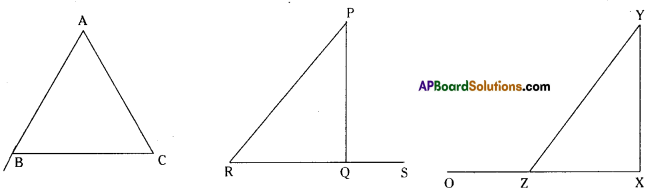
Solution:
From the figures
exterior ∠B = ∠A + ∠C
∠130° = 65° + 65°
∠PQS = ∠P + ∠R also ∠YZO = ∠X
90° = 40° + 50° 120° = 90° + 30°