Students get through AP Inter 2nd Year Physics Important Questions 7th Lesson Moving Charges and Magnetism which are most likely to be asked in the exam.
AP Inter 2nd Year Physics Important Questions 7th Lesson Moving Charges and Magnetism
Very Short Answer Questions
Question 1.
What is the importance of Oersted’s experiment? [T.S. Mar. 17]
Answer:
The importance of Oersted’s experiment is every current-carrying conductor produces a magnetic field around it which is perpendicular to the current-carrying conductor.
Question 2.
State Ampere’s law and Biot-Savart’s law.
Answer:
Ampere’s law : The line integral of the intensity of magnetic induction around a closed path is equal to g0 times the total current enclosed in it.
∴ \(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{d}} l\) = μ0 i.
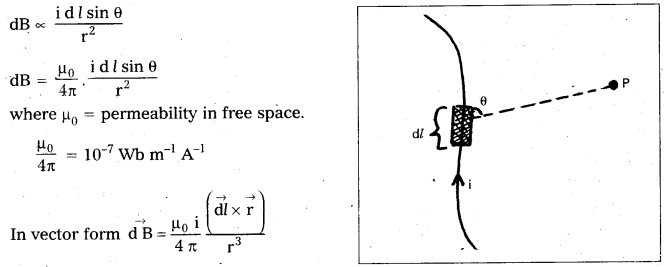
Biot – Savart’s laws : Biot – Savart’s law states that the intensity of magnetic induction (dB) due to a small element is directly proportional to the
i) current (i)
ii) length of the element (dZ)
iii) sine angle between radius vector (r) and dl and inversely proportional to the square of the point from current element.
∴dB ∝ \(\frac{\mathrm{i} \mathrm{dl} \sin \theta}{\mathrm{r}^2}\)
dB = \(\frac{\mu_0}{4 \pi} \cdot \frac{\mathrm{i} \mathrm{d} l \sin \theta}{\mathrm{r}^2}\)
![]()
Question 3.
Write the expression for the magnetic induction at any point on the axis of a circular current-carrying coil. Hence, obtain an expression for the magnetic induction at the centre of the circular coil.
Answer:
- Intensity of magnetic induction field on the axis of the circular coil B = \(\frac{\mu_0 \mathrm{ni} \mathrm{r}^2}{2\left(\mathrm{r}^2+\mathrm{x}^2\right)^{3 / 2}}\)
- At the centre of the coil B = \(\frac{\mu_0 \mathrm{ni}}{2 \mathrm{r}}\)
Question 4.
A circular coil of radius T having N turns carries a current “i”. What is its magnetic moment ?
Answer:
Magnetic moment (M) = N i A
M = N i (πr2) (∵ A = πr2)
∴ M = π N i r2
Question 5.
What is the force on a conductor of length L carrying a current “i” placed in a magnetic field of induction B ? When does it become maximum ?
Answer:
- Force on a conductor (F) = B i L sinθ
- If θ = 90°,FMax = BiL
i.e., the direction of current and magnetic field are perpendicular to,each other, then force is maximum.
Question 6.
What is the force on a charged particle of charge “q” moving with a velocity “v” in a uniform magnetic field of induction B ? When does it become maximum ?
Answer:
- Force on a charged particle (F) = B q v sin θ.
- If θ = 90°, FMax = B q v.
![]()
Question 7.
Distinguish between ammeter and voltmeter. [A.P. Mar. 17; A.P. Mar. 15]
Answer:
Ammeter
- It is used to measure current.
- Resistance of an ideal ammeter is zero.
- It is connected in series in the circuits.
Voltmeter
- It is used to measure RD between two points.
- Resistance of ideal voltmeter is infinity.
- It is connected in parallel in the circuits.
Question 8.
What is the principle of a moving coil galvanometer ?
Answer:
Moving coil galvanometer is based on the fact that when a current carrying coil is placed in a uniform magnetic field, it experiences a torque.
∴ current in the coil (i) ∝ deflecting angle (θ).
Question 9.
What is the smallest value of current that can be measured with a moving coil galvanometer ?
Answer:
Moving coil galvanometer is sensitive galvanometer, it is used to measure very small current upto 10-9 A.
Question 10.
How do you convert a moving coil galvanometer into an ammeter ?
Answer:
A small resistance is connected in parallel to the moving coil galvanometer, then it converts to ammeter.
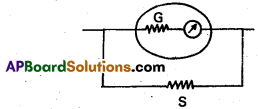
S = \(\frac{\mathrm{G}}{\frac{\mathrm{i}}{\mathrm{i}_{\mathrm{g}}}-1}\)
![]()
Question 11.
How do you convert a moving coil galvanometer into a voltmeter ? [T.S. Mar. 16, 15, 14; A.P. Mar. 16]
Answer:
A high resistance is connected in series to the moving coil galvanometer, then it converts to voltmeter.
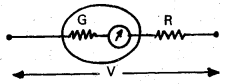
R = \(\frac{\mathrm{v}}{\mathrm{i}_g}\) – G
Question 12.
What is the relation between the permittivity of free space e0, the permeability of free space m0 and the speed of light In vaccum?
Answer:
Speed of light in vaccum (C) = \(\frac{1}{\sqrt{\mu_0 \varepsilon_0}}\)
Here μ0 = m0 = permeability in vaccum
ε0 = permittivity in vaccum.
Question 13.
A current carrying circular loop lies on a smooth horizontal plane. Can a uniform magnetic Held be set up in such a manner that the loop turns about the vertical axis ?
Answer:
Torque (τ) = \(\overrightarrow{\mathrm{M}} \times \overrightarrow{\mathrm{B}}=\mathrm{i} \overrightarrow{\mathrm{A}} \times \overrightarrow{\mathrm{B}}\) (M = n i A)
where i is current, \(\overrightarrow{\mathrm{A}}\) is area vector, \(\overrightarrow{\mathrm{B}}\) is magnetic field. Area vector \(\overrightarrow{\mathrm{A}}\) acts normal to the loop, so torque \(\vec{\tau}\) cannot act along the vertical axis. The magnetic field is not set up to turn the loop around itself.
Question 14.
A current carrying circular loop is placed in a uniform external magnetic field. If the loop is free to turn, what is its orientation when it achieves stable equilibrium?
Answer:
The plane of the loop is perpendicular to the direction of magnetic field because the torque on the loop in this orientation is zero.
![]()
Question 15.
A wire loop of irregular shape carrying current is placed in an external magnetic field. If the wire is flexible, what shape will the loop change to ? Why ?
Answer:
For a given perimeter, a circle has maximum area among all geometrical shapes. So to maximise the magnetic flux through it will assume a circular shape with its plane normal to the field.
Question 16.
Consider a tightly wound 100 turn coil of radius 10 cm, carrying a current of 1 A. What is the magnitude of the magnetic field at the centre of the coil ?
Solution:
Since the coil is tightly wound we may take each circular element to have the same radius R = 10 cm = 0.1 m. The number of turns N = 100. The magnitude of the magnetic field is (From Eq.),
B0 = \(\frac{\mu_0 \mathrm{I}}{2 \mathrm{R}} \hat{\mathrm{i}}\)
B = \(\frac{\mu_0 \mathrm{NI}}{2 \mathrm{R}}=\frac{4 \pi \times 10^{-7} \times 10^2 \times 1}{2 \times 10^{-1}}\) = 2π × 10-4 = 6.28 × 10-4 T
Question 17.
A solenoid of length 0.5 m has a radius of 1 cm and is made up of 500 turns. It carries a current of 5 A. What is the magnitude of the magnetic field inside the solenoid ?
Solution:
The number of turns per unit length is,
n = \(\frac{500}{0.5}\) = 1000 turns / m
The length l = 0.5m and radius r = 0.01 m. Thus, l/a = 50 i.e., l >> a.
Hence, we can use the long solenoid formula, namely, Eq. (B = μ0nI)
B = μ0 n I
= 4π × 10-7 × 103 × 5 = 6.28 × 10-3 T
![]()
Question 18.
A circular coil of wire consisting of 100 turns, each of radius 8.0 cm carries a current of 0.40 A. What is the magnitude of the magnetic field B at the centre of the coil ?
Solution:
Here, n = 100, r = 8cm = 8 × 10-2 m and I = 0.40 A
The magnetic field B at the centre
B = \(\frac{\mu_0}{4 \pi} \cdot \frac{2 \pi \mathrm{In}}{\mathrm{r}}=\frac{10^{-7} \times 2 \times 3.14 \times 0.4 \times 100}{8 \times 10^{-2}}\) = 3.1 × 10-4 T
The direction of magnetic field depends on the direction of current if the direction of current is anticlockwise. According to Maxwell’s right hand rule, the direction of magnetic field at the centre of coil will be perpendicular outwards to the plane of paper.
Question 19.
What is the magnitude of magnetic force per unit length on a wire carrying a current of 8A and making an angle of 30° with the direction of a uniform magnetic field of 0.15 T ?
Solution:
According to the question
I = 8 A, 6 = 30°, B = 0,15 T, l = 1 m
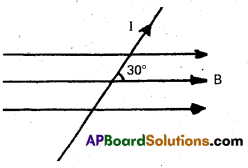
The magnitude of magnetic force
f = I (l × B) = I l B sin θ
= 8 × 1 × 0.15 × sin 30°
= \(\frac{8 \times 0.15}{2}\) = 4 × 0.15 = 0.6 N/m
Short Answer Questions
Question 1.
State and explain Biot-Savart’s law.
Answer:
Consider a very small element of length dl of a conductor carrying current (i). Magnetic induction due to small element at a point P distance r from the element.
Magnetic induction (dB) is directly proportional to i) current (i) ii) Length of the element (di) iii) sine angle between r and dl and inversely proportional to the square of the distance from small element to point P.
Question 2.
State and explain Ampere’s law.
Answer:
Ampere’s law : The line integral of the intensity of magnetic induction field around closed path is equal to μ0 times the net current (i) enclosed by the path.
\(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{d} l}\) = μ0i
Proof: Consider a long straight conductor carrying current i as shown in figure. Magnetic induction at a distance r from the conductor is given by
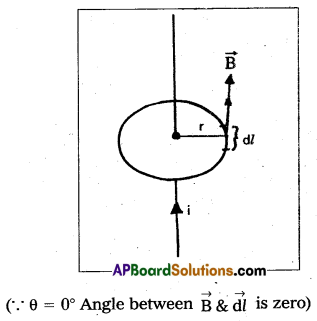
B = \(\frac{\mu_0 \mathrm{i}}{2 \pi \mathrm{r}}\) (From Biot-Savart’s law)
The value of B is same at all points on the circle.
\(\oint \overrightarrow{\mathrm{B}} . \overrightarrow{\mathrm{d} l}=\oint \mathrm{B} \mathrm{d} l \cos \theta\)
= \(\mathrm{B} \oint \mathrm{d} l\) = B × 2π
= \(\frac{\mu_0 \mathrm{i}}{2 \pi \mathrm{r}}\) × 2πr
\(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{d} l}\) = μ0i
This proves Ampere’s circuital laws.
![]()
Question 3.
Find the magnetic induction due to a long current carrying conductor.
Answer:
Consider a long straight conductor carrying a current i. Let P be a point at a distance r from the conductor. Let r be the radius of the circle passing through point p.
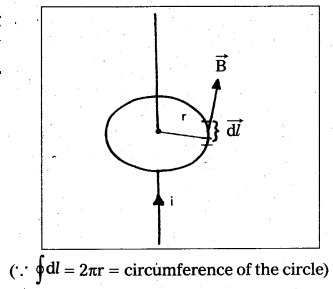
Magnetic induction is same at all points on the circle. Consider a small element of length dl.
\(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{d} l}=\oint \mathrm{Bd} l \cos \theta\)
Angle between B and dl is zero i.e. θ = 0
= \(\mathrm{B} \oint \mathrm{d} l\)
\(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{d} l}\) = B (2πr) ………………. (1)
According to Ampere’s laws
\(\oint \overrightarrow{\mathrm{B}} \cdot \overrightarrow{\mathrm{d} l}\) = μ0i ……………. (2)
From equations (1) and (2), B (2πr) = μ0i
= \(\frac{\mu_0 \mathrm{i}}{2 \pi \mathrm{r}}\)
Question 4.
Derive an expression for the magnetic induction at the centre of a current carrying circular coil using Biot-Savart’s law.
Answer:
Consider a circular coil of radius r and carry a current! Consider a small element ‘dl’. Let O is the centre of the coil. By using Biot – Savart’s law,
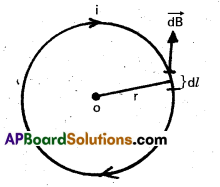
dB = \(\frac{\mu_0}{4 \pi} \cdot \frac{\mathrm{id} l \sin \theta}{\mathrm{r}^2}\)
Here angle \(\overrightarrow{\mathrm{d} l}\) and \(\overrightarrow{\mathrm{r}}\) is 90° (i.e., θ = 90°)
dB = \(\frac{\mu_0}{4 \pi} \frac{\mathrm{idl}}{\mathrm{r}^2}\) …………… (1)
As the field due to all elements of the circular loop have the same direction. The resultant magnetic field can be obtained by integrating equation (1)
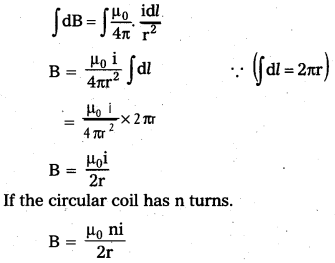
![]()
Question 5.
Derive an expression for the magnetic induction of a point on the axis of a current carrying circular coil using Biot-Savart’s law.
Answer:
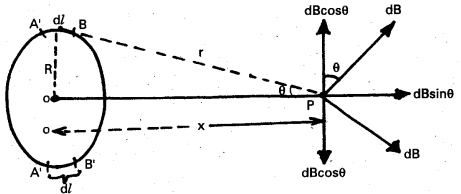
Consider a circular coil of radius R and carrying a current i. Let P is a point on the axis at a distance x from the centre O. Let r be the distance of small element (dl) from P.
From Biot – savart’s law
dB = \(\frac{\mu_0}{4 \pi} \cdot \frac{\mathrm{id} l \sin \theta}{\mathrm{r}^2}=\frac{\mu_0}{4 \pi} \cdot \frac{\mathrm{id} l}{\mathrm{r}^2}\) ……………….. (1)
(∵ θ = 90° Angle between \(\overrightarrow{\mathrm{d} l}\) and \(\overrightarrow{\mathrm{r}}\))
dB can be resolved into two components dB cosθ and dB sinθ. If we consider another This also resolved into dB cosθ and dB sinθ.
The components along the axis will add up and perpendicular to the axis will cancel.
∴ Resultant magnetic induction at P is
Question 6.
Explain how crossed E and B fields serve as a velocity selector.
Answer:
When a charged particle q moving with a velocity v in presence of both electric and magnetic fields.
The force experienced due to electric field FE = q\(\overrightarrow{\mathrm{E}}\)
The force experienced due to magnetic field FB = q \((\vec{v} \times \vec{B})\)
Consider electric and magnetic fields are perpendicular to each other and also perpendicular to the velocity of the particle.
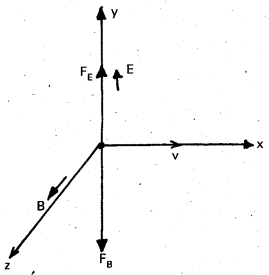
E = E\(\hat{\mathrm{j}}\),B = B\(\hat{\mathrm{k}}\), v = υ\(\hat{\mathrm{i}}\)
FE = qE\(\hat{\mathrm{j}}\), FB =q(v\(\hat{\mathrm{i}}\) × B\(\hat{\mathrm{k}}\)) = – qvB\(\hat{\mathrm{j}}\)
∴ F = FE + FB
F = q (E – υB)\(\hat{\mathrm{j}}\)
Thus electric and magnetic forces are in opposite directions.
We adjust E and B such that, the forces are equal
FE = FB
qE = q υ B
υ = \(\frac{E}{B}\)
This condition can be used to selefct charged particles of a particular velocity. The crossed field E and B serve as a velocity selector.
![]()
Question 7.
What are the basic components of a cyclotron ? Mention its uses ?
Answer:
Cyclotron is a device used to accelerate positively charged particles like protons, α – particles, deutrons etc.
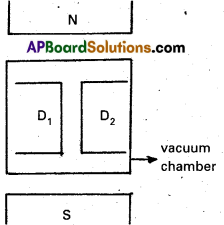
Cyclotron mainly consists of
- Two hollow D-shaped metallic chambers D1 and D2
- High-frequency oscillator
- Strong electro magnet
- Vaccum chamber.
Uses of cyclotron :
- It is used for producing radioactive material for medical purposes i.e. diagnostics and treatment of chronic diseases. “
- It is used to improve the quality of solids by adding ions.
- It is used to synthesise fresh substances.
- It is used to bombard the atoms with highly accelerated particles to study the nuclear reactions.
Question 8.
Derive an expression for the magnetic dipole moment of a revolving electron. [A.P. Mar. 16]
Solution:
Consider an electron revolving in a circular orbit of radius r with speed v and frequency υ. If the electron cross a point P on the circle in every revolution, then distance travelled by electron to complete one revolution = 2πr.
No. of revolutions in one second (υ) = \(\frac{\mathrm{v}}{2 \pi \mathrm{r}}\)
The electric current (i) = \(\frac{\text { Charge }}{\text { Time }}\) = charge × frequency
i = e × \(\frac{\mathrm{v}}{2 \pi \mathrm{r}}\)
∴ Magentic dipole moment (M) = iA (∵ N = 1)
M = \(\frac{\mathrm{ev}}{2 \pi \mathrm{r}}\) × πr2 (∵ A = πr2)
M = \(\frac{\mathrm{evr}}{2}\)
Long Answer Questions
Question 1.
Deduce an expression for the force on a current carrying conductor placed in a magnetic field. Derive an expression for the force per unit length between two parallel current-carrying conductors.
Answer:
Expression for the Force acting on a current carrying conductor :
Consider a straight conductor (wire) of length T, area of cross section ’A1, carrying a current T, which is placed in a uniform magnetic field of induction ’B’ as shown in fig.
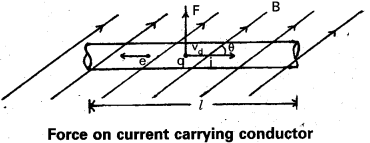
We know the external magnetic field exerts a force on the conductor.
The electrons in effect move with an average velocity called drift velocity ‘Vd‘. The direction of conventional current will be opposite to the direction of drift velocity.
Let us assume that the current flows through the conductor from left ‘B’ in the plane of the paper makes an angle ‘θ’ with the direction of current ‘i’ as shown in fig.
If F’ is the force acting on the charge ‘q’ in B.
∴ F’ = q Vd B sin θ
If ‘n’ represents number of moving electrons per unit volume (∵ n = \(\frac{N}{V}\))
∴ Current i = nq Vd A
If ‘N’ is the number of electrons in the length ‘l’
N = nlA
Total force on conductor F = F’.N (∵N = nV = n × A × l)
= (q Vd B sin θ) (nlA)
(nqVdA) (lB sin θ)
∴ F = ilB sin θ
Case (i) : If θ = 0°, FMin = 0
Case (ii) : If θ = 90°, FMax = Bil
Expression for the force between two Parallel conductors carrying conductors :
Consider two straight parallel conductors AB and ‘CD’ carrying currents ‘ix’ and ‘i2’ and which are separated by a distance ‘r’ as shown in fig.
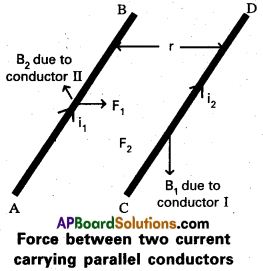
If B1 and B2 are magnetic inductions produced by the current carrying conductors AB and CD. Magnetic induction Bx at a distance ‘r’ from the conductor ‘AB’ can be written as B1 = \(\frac{\mu_0 \mathrm{i}_1}{2 \pi \mathrm{r}}\)
If ‘F’ is forœ acting on ‘CD’ clue to magnetic induction ‘B1‘ then
FCD = i2lB1
Where l = length of the conductor
FCD = i2l \(\left(\frac{\mu_0 i_1}{2 \pi \mathrm{r}}\right)=\frac{\mu_0 i_1 i_2 l}{2 \pi r}\) ……………. (1)
The direction of the force can be determined by using Flemings left hand rule.
Similarly we can find the force acting on the A conductor AB due to magnetic induction B2.
FAB = i1lB2
∴ FAB = i1l \(\left(\frac{\mu_0 i_2}{2 \pi r}\right)\) ………….. (2) [∵B2 = \(\left(\frac{\mu_0 i_2}{2 \pi r}\right)\)]
From the equations (1) and (2) FAB = FCD = \(\frac{\mu_0 \mathrm{i}_1 \mathrm{i}_2 l}{2 \pi \mathrm{r}}\)
∴ Force between two parallel, straight conductors carrying currents,
F = \(\frac{\mu_0 \mathrm{i}_1 \mathrm{i}_2 l}{2 \pi \mathrm{r}}\)
Force per unit length \(\frac{\mathrm{F}}{l}=\frac{\mu_0 \mathrm{i}_1 \mathrm{i}_2}{2 \pi \mathrm{r}}\)
![]()
Question 2.
Obtain an expression for the torque on a current carrying loop placed in a uniform ‘ magnetic field. Describe the construction and working of a moving coil galvanometer.
Answer:
Torque acting on a coil carrying a current kept in a uniform magnetic field : Let a rectangular current loop ABCD of length l = AB = CD and width b = AD – BC carrying a current “i” be suspended in a magnetic field of flux density B.
The normal ON drawn to the plane of the coil makes an angle ‘θ’ with the magnetic field B.
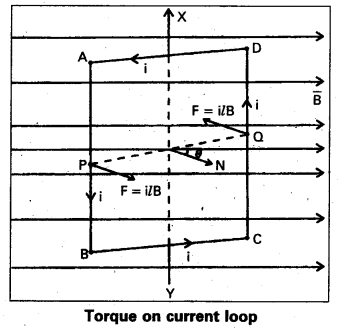
Force on arm AD = \(\mathrm{i} \overline{\mathrm{b}} \times \overline{\mathrm{B}}\) acting upwards along the axis of suspension
Force on arm BC = \(\mathrm{i} \overline{\mathrm{b}} \times \overline{\mathrm{B}}\) acting downwards along the axis of suspension
Hence these two forces cancel.
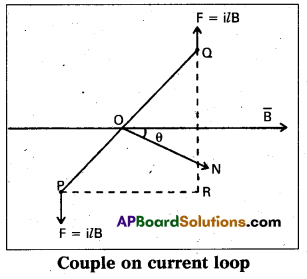
Force on arm AB = ilB acting perpendicular to the plane as shown.
Force on arm CD = ilB acting perpendicular to the plane as shown.
These two forces constitute a couple on the coil.
Moment of the couple = (Force) × (Perpendicular distance between the forces) = ilB (PQ sin θ)
Torque = ilB b sinθ
But l × b = Area of coil
∴ Torque = iAB sin θ
If the loop has ‘n’ turns the torque on the coil
τ = n i AB sin θ
If ‘Φ’ is the deflection of the coil, that is the angle between the plane of the coil and magnetic field B
τ = n i AB cos Φ
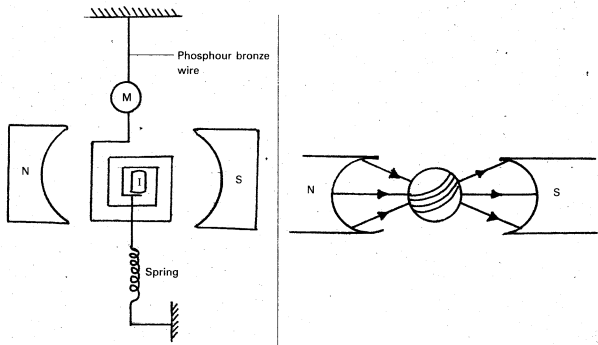
Moving coil galvanometer:
Principle : When a current carrying coil is placed in the uniform magnetic field, it experiences a torque.
Construction :
- It consists of a coil wound on a non metallic frame.
- A rectangular coil is suspended between two concave shaped magnetic poles with the help of phosphour Bronze wire.
- The lower portion of the coil is connected to a spring.
- A small plane mirror M is fixed to the phosphour Bronze wire to measure the deflection of the coil.
- A small soft iron cylinder is placed within the coil without touching the coil. The soft iron cylinder increases the induction field strength.
- The concave shaped magnetic poles render the field radial. So maximum torque acting on it.
- The whole of the apparatus is kept inside a brass case provided with a glass window.
Theory:
Consider a rectangular coil of length l and breadth b and carrying current i suspended in the induction field strength B.
Deflecting torque (τ) = B i A N …………….. (5)
where A = Area of the coil
N = Total number of turns.
The restoring torque developed in the suspension = C θ …………….. (2)
Where C is the couple per unit twist and 9 is the deflection made by the coil.
When the coil is in equilibrium position
Deflecting torque = Restoring torque
B i A N = Cθ
i = \(\left(\frac{\mathrm{C}}{\mathrm{BAN}}\right) \theta\)
Where K = \(\frac{\mathrm{C}}{\mathrm{BAN}}\) = Galvanometer constant.
i = K θ ……………… (3)
i ∝ θ
Thus deflection of the coil is directly proportional to the current flowing through it. The deflection in the coil is measured using lamp and scale arrangement.
![]()
Question 3.
How can a galvanometer be converted to an ammeter ? Why is the parallel resistance smaller that the galvanometer resistance ? A moving coil galvanometer can measure a current of 10-6 A. What is the resistance of the shunt required if it is to measure 1A ?
Answer:
Conversion of Galvanometer into Ammeter :
Galvanometer is converted into an ammeter by connecting a suitable resistance is parallel to it:
This arrangement decreases the effective resistance.
Ammeter is used for measuring the current in an electric circuit and it is connected in series in circuit. The inclusion of the ammeter in the circuit should not alter the current or total resistance of the circuit so it has very low resistance.
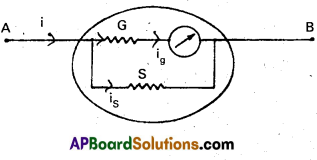
The resistance of An ideal Ammeter is zero.
Let G and S be the Galvanometer and shunt resistances respectively.
Let ‘i’ be the total current, divided at A into ig and is as shown in fig.
From Kirchhoffs Ist law, i = ig + is
As ‘G’ and ‘S’ are parallel P.D. across
Galvanometer = P.D. across shunt
igG = isS
S = \(\frac{\mathrm{i}_{\mathrm{g}}}{\mathrm{i}_{\mathrm{s}}} \mathrm{G}\)
= \(\frac{\mathrm{Gi}_{\mathrm{g}}}{\mathrm{i}-\mathrm{i}_{\mathrm{g}}}\) [∵ is = i – ig]
S = \(\frac{\mathrm{G}}{\frac{\mathrm{i}}{\mathrm{i}_{\mathrm{g}}}-1}\)
If \(\frac{\mathrm{i}}{\mathrm{i}_{\mathrm{g}}}\) = n ⇒ ig = \(\frac{\mathrm{i}}{\mathrm{n}}\)
∴ The current flowing through the galvanometer be \(\left(\frac{1}{n}\right)^{\text {th }}\) of total current.
∴ S = \(\frac{\mathrm{G}}{\mathrm{n}-1}\)
If ‘R’ is the effective resistance between points ‘A’ and ‘B’ then
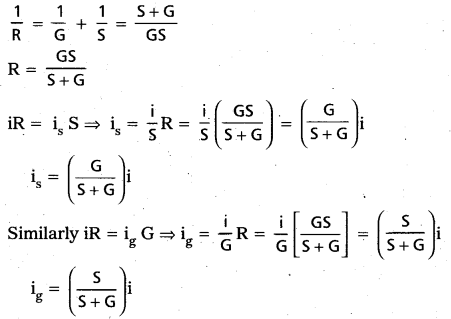
Hence current through galvanometer is proportional to the total current. Since ‘S’ is small major portion of the current flows through it and a small portion of current flows through G. So shunt protects the galvanometer from high currents. Parallel resistance is smaller than Galvanometer resistance because to protect the Galvanometer from high (large) current (or) to pass. Large currents through shunt and small current passes through the galvanometer.
Solution for the problem : Current in the circuit i = 1A;
Current through the galvanometer, ig = 10-6A
Shunt resistance, S = \(\frac{G}{n-1}=\frac{G}{\frac{i}{i_g}-1}=\frac{G}{10^6-1}=\frac{G}{99.999} \Omega\)
![]()
Question 4.
How can a galvanometer be converted to a voltmeter ? Why is the series resistance greater that the galvanometer resistance ? A moving coil galvanometer of resistance 5Ω can measure a current of 15mA. What is the series resistance required if it is to measure 1.5V ?
Answer:
Conversion of Galvanometer into Voltmeter : A galvanometer is converted into voltmeter by connecting a high resistance (R) in series with it. Voltmeter is used to measure the P.D. between any two points in circuit and it is connected in parallel to the component of the circuit.
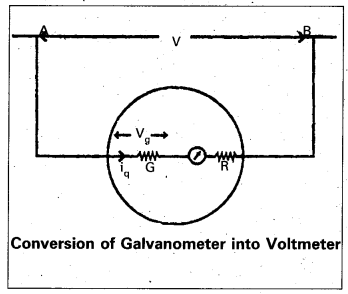
Let ‘V’ be the potential difference to be measured between the points ‘A’ and ‘B’.
∴ V = (R + G) ig [∴ V = iR]
ig = Current passing through the galvanometer
\(\frac{\mathrm{V}}{\mathrm{i}_{\mathrm{g}}}\) = R + G
R = \(\frac{\mathrm{V}}{\mathrm{i}_{\mathrm{g}}}\) – G ……………. (1)
The value of ‘R’ can be calculated by using the above formula. If Vg is the maximum P.D. across the galvanometer then Vg = ig G
∴ ig = \(\frac{V_g}{G}\) …………….. (2)
Substitute ‘ig‘ in Equ (1)
R = \(\frac{V_G}{V_g}\) – G = \(\left(\frac{V}{V_g}-1\right)\)
If \(\frac{V}{V_g}\) = n ⇒ R = G(n – 1)
Note : n = \(\frac{V}{V_g}\) is the ratio of maximum voltage to be measured to the maximum voltage across the galvanometer.
Series resistance is greater than galvanometer resistance because the current in external resistance and potential difference will be decreased and to increase the resistance of the galvanometer.
Solution for the problem:
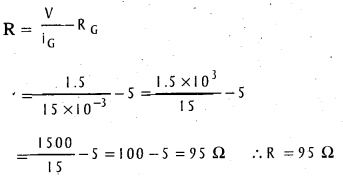
Problems
Question 1.
Two long and parallel straight wires A and B canying currents of 8.0 A and 5.0 A in the same direction are separated by a distance of 4.0 cm. Estimate the force on a 10 cm section of wire A.
Solution:
Given I1 = 8A, I2 = 5A and r = 4 cm = 0.4m
F = \(\frac{\mu_0}{4 \pi} \cdot \frac{2 \mathrm{I}_1 \mathrm{I}_2}{\mathrm{r}}=\frac{10^{-7} \times 2 \times 8 \times 5}{0.04}\) = 2 × 10-4 N
The force on A of length 10 cm is F1 = F × 0.1 (∵ 1 m = 100 cm)
F1 = 2 × 10-4 × 0.1
F1 = 2 × 10-5 N.
Question 2.
A current of 10A passes through two very long wires held parallel to each other and separated by a distance of 1m. What is the force per unit length between them ? [A.P. & T.S. Mar. 15]
Answer:
i1 = i2 = 10A
r = 1m
\(\frac{\mathrm{F}}{l}=\frac{\mu_0 \mathrm{i}_1 \mathrm{i}_2}{2 \pi \mathrm{r}}\)
= \(\frac{4 \pi \times 10^{-7} \times 10 \times 10}{2 \pi \times 1}\)
\(\frac{\mathrm{F}}{l}\) = 2 × 10-5 Nm-1.
![]()
Question 3.
A straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal magnetic field B (Fig.). What is the magnitude of the magnetic field ?
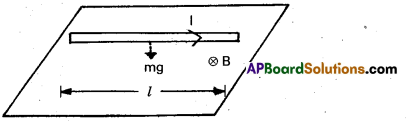
Solution:
From Eq F = Il × B we find that there is an upward force F, of magnitude IlB,. For mid-air suspension, this must be balanced by the force due to gravity.
m g = I lB mg
B = \(\frac{\mathrm{mg}}{\mathrm{I} l}\)
= \(\frac{0.2 \times 9.8}{2 \times 1.5}\) = 0.65 T
Note that it would have been sufficient to specify mll, the mass per unit length of the wire. The earth’s magnetic field is approximately 4 × 10-5 T and we have ignored it.
Question 4.
The horizontal component of the earth’s magnetic field at a certain place is 3.0 × 10-5 T and the direction of the field is from the geographic south to the geographic north. A very long straight conductor is carrying a steady current of 1 A. What is the force per unit length on it when it is placed on a horizontal table and the direction of the current is
(a) east to west;
(b) south to north ?
Solution:
F = Il × B
F = IlB sin θ
The force per unit length is
f = F/l = IB sinθ
a) When the current is flowing from east to west,
θ = 90°
Hence,
f = IB
= 1 × 3 × 10-5 = 3 × 10-5 Nm-1
This is larger than the value 2 × 10-7 Nm-1 quoted in the definition of the ampere. Hence it is important to eliminate the effect of the earth’s magnetic field and other stray fields while standardising the ampere.
The direction of the force is downwards. This direction may be obtained by the directional property of cross product of vectors.
b) When the current is flowing from south to north, .
θ = 0°
f = 0
Hence there is no force on the conductor.
Textual Examples
Question 1.
A straight wire of mass 200 g and length 1.5 m carries a current of 2 A. It is suspended in mid-air by a uniform horizontal niagnetic field B (Fig.). What is the magnitude of the magnetic field ?
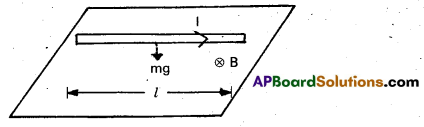
Solution:
From Eq F = Il × B we find that there is an upward force F, of magnitude IlB,. For mid-air suspension, this must be balanced by the force due to gravity, m g = I lB .
B = \(\frac{\mathrm{mg}}{\mathrm{I} l}\)
= \(\frac{0.2 \times 9.8}{2 \times 1.5}\) = 0.65 T
Note that it would have been sufficient to specify m/l, the mass per unit length of the wire. The earth’s magnetic field is approximately 4 × 10-5 T and we have ignored it.
![]()
Question 2.
If the magnetic field is parallel to the positive y-axis and the charged particle is moving along the positive x-axis (Fig.), which way would the Lorentz force be for (a) an electron (negative charge), (b) a proton (positive charge).

Solution:
The velocity v of particle is along the x-axis, while B, the magnetic field is along the y-axis, so v × B is along the z-axis (screw rule or right-hand thumb rule). So, (a) for electron it will be along -z axis, (b) for a positive charge (proton) the force is along +z axis.
Question 3.
What is the radius of the path of ah electron (mass 9 × 10-31 kg and charge 1.6 × 10-19 C) moving at a speed of 3 × 107 m/s in a magnetic field of 6 × 10-4 T perpendicular to it ? What is its frequency ? Calculate its energy in keV. (1 eV = 1.6 × 10-19 J).
Solution:
Using Eq. r = mυ/qb we find
r = mυ/(qB) = 9 × 10-31 kg × 3 × 107 m s-1 / (1.6 × 10-19 C × 6 × 10-4 T)
= 26 × 10-2 m = 26 cm
v = υ / (2 πr) = 2 × 106 s-1 = 2 × 106 Hz = 2MHz.
E = (1/2)mυ2 = (1/2) 9 × 10-31 kg × 9 × 1014 m2/s = 40.5 × 10-17 J
≈ 4 × 10-16 J = 2.5 keV.
![]()
Question 4.
A cyclotron’s oscillator frequency is 10 MHz. What should be the operating magnetic field for accelerating protons ? If the radius of its does is 60 cm, what is the kinetic energy (in MeV) of the proton beam produced by the accelerator.
(e = 1.60 × 10-19 C, mp = 1.67 × 10-27 kg, 1 MeV = 1.6 × 10-13 J).
Solution:
The oscillator frequency should be same as proton’s cyclotron frequency.
Using Eqs. r = mυ/qb and ω = 2πυ = \(\frac{\mathrm{qB}}{\mathrm{M}}\) we have
B = 2π m υ/q = 6.3 × 1.67 × 10-27 × 107 / (1.6 × 10-19) = 0.66 T
Final velocity of protons is
υ = r × 2π v = 0.6 m × 6.3 × 107 = 3.78 × 107 m/s.
E = 1/2 mv2 = 1.67 × 10-27 × 14.3 × 1014/ (2 × 1.6 × 10-13) = 7 MeV
Question 5.
element ∆1 = ∆x\(\hat{\mathrm{i}}\) is placed at the origin and carries a large current I = 10 A (Fig.). Wat is the magnetic field on the y-axis at a distance of 0.5 m. ∆x = 1 cm.
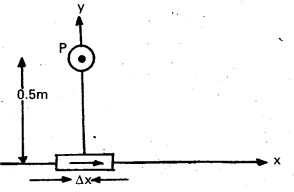
Solution:
|dB| = \(\frac{\mu_0}{4 \pi} \frac{I \mathrm{dl} \sin \theta}{\mathrm{r}^2}\)
dl = ∆x = 10-2 m, I = 10 A, r = 0.5 m = y, μ0/4π = 10-7 \(\frac{\mathrm{Tm}}{\mathrm{A}}\)
θ = 90°; sin θ = 1
|dB| = \(\frac{10^{-7} \times 10 \times 10^{-2}}{25 \times 10^{-2}}\) = 4 × 10-8 T
The direction of the field is in the +z-direction. This is so since,
dl × r = \(\Delta \mathrm{x} \hat{\mathrm{i}} \times \mathrm{y} \hat{\mathrm{j}}=\mathrm{y} \Delta \mathrm{x}(\hat{\mathrm{i}} \times \hat{\mathrm{j}}) \mathrm{y} \Delta \mathrm{x} \hat{\mathrm{k}}\)
We remind you of the following cyclic property of cross-products,
\(\hat{\mathrm{i}} \times \hat{\mathrm{j}}=\mathrm{k} ; \hat{\mathrm{j}} \times \hat{\mathrm{k}}=\hat{\mathrm{i}} ; \hat{\mathrm{k}} \times \hat{\mathrm{i}}=\hat{\mathrm{j}}\)
Note that the field is small in magnitude.
![]()
Question 6.
A straight wire carrying a current of 12 A is bent into a semi-circular arc of radius 2.0 cm as shown in Fig. Consider the magnetic field B at the centre of the arc. (a) What is the magnetic field due to the straight segments ? (b) In what way the contribution to B from the semicircle differs from that of a circular loop and in what way does it resemble ? (c) Would your answer be different if the wire were bent into a semi-circular arc of the same radius but in the opposite way as shown in Fig. (b) ?
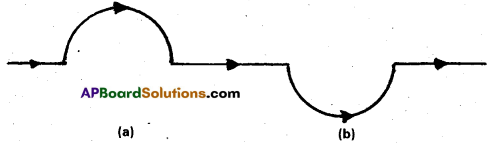
Solution:
a) dl and r for each element of the straight segments are parallel. Therefore, dl × r = 0. Straight segments do not contribute to |B|.
b) For all segments of the semicircular arc, dl × r are all parallel to each other (into the plane of the paper). All such contributions add up in magnitude. Hence direction of B for a semicircular arc is given by the right-hand rule and magnitude is half that of a circular loop. Thus B is 1.9 × 10-4 T normal to the plane of the paper going into it.
c) Same magnitude of B but opposite in direction to that in (b).
Question 7.
Consider a tightly wound 100 turn coil of radius 10 cm, carrying a current of 1 A. What is the magnitude of the magnetic field at the centre of the coil?
Solution:
Since the coil is tightly wound we may take each circular element to have the same radius R = 10 cm = 0.1 m. The number of turns N = 100. The magnitude of the magnetic field is (From Eq.),
B0 = \(\frac{\mu_0 \mathrm{I}}{2 \mathrm{R}} \hat{\mathrm{i}}\)
B = \(\frac{\mu_0 \mathrm{NI}}{2 \mathrm{R}}=\frac{4 \pi \times 10^{-7} \times 10^2 \times 1}{2 \times 10^{-1}}\) = 2π × 10-4 = 6.25 × 10-4 T
Question 8.
Magnetic field due to a long current-carrying wire Oersted’s experiments showed that there is a magnetic field around a current-carrying wire. We determine the magnitude of magnetic field at some distance from a long striaght wire carrying a current I.
Solution:
The direction of the field is given by the right hand rule. The figure shows an element dl of the current-carrying wire. The point P, where the field is to be determined is at a perpendicular distance ‘S’ from the wire. The position vector of P from dl is r.
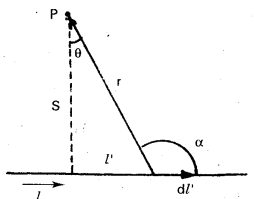
The magnitude dB of the magnetic field due to dl is given by Biot-Savart law to be
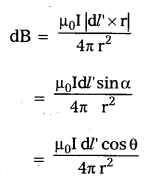
now from the figure S = r cosθ which gives l/r2 = cos2θ/s2
and l’ = S tanθ which gives dl’ = S sec2θ dθ = S dθ/cos2θ
thus dB = \(\frac{\mu_0 I \cos \theta}{4 \pi S}\) dθ
we integrate this to get B at P If the wire is very long then the limits for 0 would be -π/2 to π/2
thus B = \(\frac{\mu_0 l}{2 \pi S}\) (emerging from the paper at P)
![]()
Question 9.
Find \(\oint\) B . dl for the paths shown in (a) and (b)
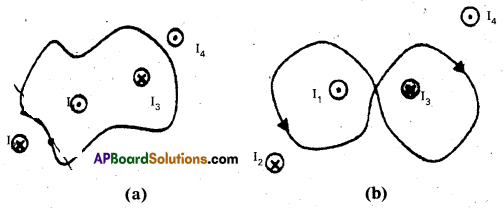
Solution:
a) Going around theKpath in the anticlockwise direction, I1 is taken as positive while I3 is negative. Currents I2 and I4 do not matter as they are NOT enclosed by the path.
\(\oint\) B . dl = μ0(I1 – I3)
Note : Currents I2 and I4 create magnetic fields all around them and B due to them on any element of the path would be non-zero. However, the sum B.dZ due to them would be zero, b) Calculation of B. dl for the entire path can be broken up into two separate calculations, one covering all contributions from an anti-clockwise traversal around I1 and the other covering all contributions from a clockwise traversal around I3. Thus
\(\int_1 \mathrm{~B} . \mathrm{d} l\) = μ0I1 for all elements around I1 traversed in an anti-clockwise direction
\(\int_2 \mathrm{~B} . \mathrm{d} l\) = μ0I3 for all elements around I3 traversed in a clockwise direction; I3 taken as positive because it is flowing into the plane. Thus the total \(\oint\) B.dl = μ0 (I1 – I3)
Question 10.
Figure shows the circular cross-section of a long straight wire of radius a carrying steady current I. The current I is uniformly distributed across this cross-section. Calculate the magnetic field in the region r < a (dashed inner circle) and r > a (dashed outer circle).
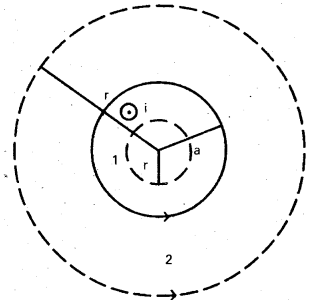
Solution:
a) Consider the case r > a. The Amperian loop, labelled 2, is a circle concentric with the cross-section. For this loop,
L = 2πr
Ie = Current enclosed by the loop = I
The result is the familiar expression for a long straight wire B(2πr) = μ0I
B = \(\frac{\mu_0 I}{2 \pi \mathrm{r}}\) ……………… (1)
b) Consider the case r < a. The Amperian loop is a circle labelled 1 . For this loop, taking the radius of the circle to be r,
L = 2 π r
Now the current enclosed Ie is not I (because r < a), but is less than this value. Since the current distribution is uniform, the current enclosed is,
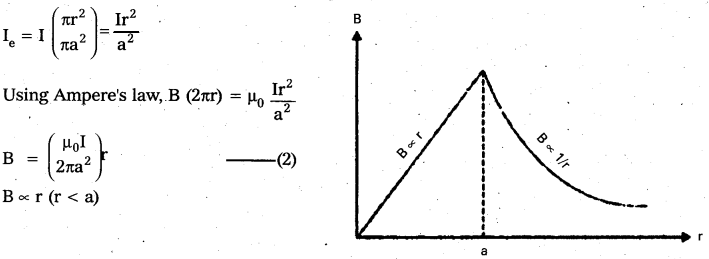
Figure shows a plot of the magnitude of B with distance r from the centre (axis) of the wire. The direction of the field is tangential to the respective circular loop (1 or 2) and given by the right-hand rule described earlier in this section.
This example possesses the required symmetry so that Ampere’s law can be applied readily.
![]()
Question 11.
A solenoid of length 0.5 m has a radius of 1 cm and is made up of 500 turns. It carries a current of 5 A. What is the magnitude of the magnetic field inside the solenoid ?
Solution:
The number of turns per unit length is,
n = \(\frac{500}{0.5}\) = 1000 turns / m
The length l = 0.5m and radius r = 0.01 m. Thus, l/a = 50 i.e., l > >a.
Hence, we can use the long solenoid formula, namely, Eq. (B = μ0nI)
B = μ0 n I
= 4π × 10-7 × 103 × 5 = 6.28 × 10-3 T
Question 12.
The horizontal componet of the earth’s magnetic field at a certain place is 3.0 × 10-5 T and the direction of the field is from the geographic south to the geographic north. A very long straight conductor is carrying a steady current of 1A. What is the force per unit length on it when it is placed on a horizontal table and the direction of the current is
(a) east to west;
(b) south to north ?
Solution:
F = Il × B
F = IlB sin θ
The force per unit length is
f = F/l = IB sinθ
a) When the current is flowing from east to west,
θ = 90°
Hence,
f = IB
= 1 × 3 × 10-5 = 3 × 10-5 Nm-1
This is larger than the value 2 × 10-7 Nm-1 quoted in the definition of the ampere. Hence it is important to eliminate the effect of the earth’s magnetic field and other stray fields while standardising the ampere.
The direction of the force is downwards. This direction may be obtained by the directional property of cross product of vectors.
b) When the current is flowing from south to north,
θ = 0°
f = 0
Hence there is no force on the conductor.
![]()
Question 13.
A 100 turn closely wound circular coil of radius 10 cm carries a current of 3.2A. (a) What is the field at the centre of the coil ? (b) What is the magnetic moment of this coil ?
The coil is placed in a vertical plane and is free to rotate about a horizontal axis which coincides with its diameter. A uniform magnetic field of 2T in the horizontal direction exists such that initially the axis of the coil is in the direction of the field. The coil rotates through an angle of 90° under the influence of the magnetic field, (c) What are the magnitudes of the torques on the coil in the initial and final position ? (d) What is the angular speed acquired by the coil when it has rotated by 90° ? The moment of inertia of the coil is 0.1 kg m2. [A.P. Mar. 19]
Solution:
a) From B = \(\frac{\mu_0 \mathrm{NI}}{2 \mathrm{R}}\)
Here, N = 100; I = 3.2 A, and R = 0.1 m. Hence,
B = \(\frac{4 \pi \times 10^{-7} \times 10^2 \times 3.2}{2 \times 10^{-1}}=\frac{4 \times 10^{-5} \times 10}{2 \times 10^{-1}}\) (using π × 3.2 = 10)
= 2 × 10-3 T
The direction is given by the right-hand thumb rule.
b) The magnetic moment is given by
m = N I A = N I π r2 = 100 × 3.2 × 3.14 × 10-2 = 10 A m2
The direction is once again given by the right hand thumb rule.
c) τ = |m × B|
= mBsin θ
Initially, θ = 0. Thus initial torque τi = 0. Finally, θ = π/2 (or 90°).
Thus, final torque τf = m B = 10 × 2 = 20 N m.
d) From Newton’s second law.
I\(\frac{\mathrm{d} \omega}{\mathrm{dt}}\) = mBsin θ
where I is the moment of inertia of the coil. From chain rule,
\(\frac{\mathrm{d} \omega}{\mathrm{dt}}=\frac{\mathrm{d} \omega}{\mathrm{d} \theta} \frac{\mathrm{d} \theta}{\mathrm{dt}}=\frac{\mathrm{d} \omega}{\mathrm{d} \theta} \omega\)
Using this,
Iω dω = m B sinθ dθ
Integrating from θ = 0 to θ = π/2,
\(\mathrm{I} \int_0^{\omega \mathrm{I}} \omega \mathrm{d} \omega=\mathrm{mB} \int_0^{\pi / 2} \sin \theta d \theta\)
\(\mathrm{I} \frac{\omega_{\mathrm{I}}^2}{2}=-\left.\mathrm{mB}[\cos \theta]\right|_0 ^{\pi / 2}=\mathrm{mB}\)
ωf = \(\left(\frac{2 \mathrm{mB}}{\mathrm{I}}\right)^{1 / 2}=\left(\frac{2 \times 20}{10^{-1}}\right)^{1 / 2}\) = 20 s-1
Question 14.
a) A current-carrying circular loop lies on a smooth horizontal plane. Can a uniform magnetic field be set up in such a manner that the loop turns around it self (i.e. turns about the vertical axis).
Solution:
No, because that would require τ to the in the vertical direction. But τ = I A × B, and since A of the horizontal loop is in the vertical direction, τ would be in the plane of the loop for any B.
b) A current-carrying circular loop is located in a uniform external magnetic field. If the loop is free to turn, what is its. orientation of stable equilibrium ? Show that in this orientation, the flux of the total field (external field + field produced by the loop) is maximum.
Solution:
Orientation of stable equilibrium is one where the area vector A of the loop is in the direction of external magnetic field. In this orientation, the magnetic field produced by the loop is in the same direction as external field, both normal to the plane of the loop, thus giving rise to maximum flux of the total field.
c) A loop of irregular shape carrying current is located in an external magnetic field. If the wire is flexible, why does it change to a circular shape ? What could be the sense of current in the loop and the direction of magnetic field ?
Solution:
It assumes circular shape with its plane normal to the field to maximize flux, since for a given perimeter, a circle encloses greater area than any other shape.
![]()
Question 15.
In the circuit (Fig.) the current is to be measured. What is the value of the current if the ammeter shown (a) is a galvanometer with a resistance RG = 60.00 Ω; (b) is a galvanometer described in (a) but converted to an ammeter by a shunt resistance rs = 0.02 Ω; (c) is an ideal ammeter with zero resistance ?
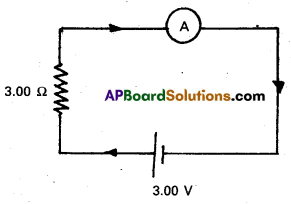
Solution:
a) Total resistance in the circuit is,
RG + 3 = 63 Ω. Hence I = 3/63 = 0.048 A.
b) Resistance of the galvanometer converted to an ammeter is,
\(\frac{\mathrm{R}_{\mathrm{G}} \mathrm{r}_{\mathrm{s}}}{\mathrm{R}_{\mathrm{G}}+\mathrm{r}_{\mathrm{s}}}=\frac{60 \Omega \times 0.02 \Omega}{(60+0.02) \Omega}\) = 0.02 Ω
Total resistance in the circuit is,
0.02 Ω + 3 Ω = 3.02 Ω. Hence I = 3/3.02 = 0.99 A.
c) For the ideal ammeter with zero resistance,
1 = 3/3 = 1.00 A