Andhra Pradesh BIEAP AP Inter 2nd Year Physics Study Material 13th Lesson Atoms Textbook Questions and Answers.
AP Inter 2nd Year Physics Study Material 13th Lesson Atoms
Very Short Answer Questions
Question 1.
What is the angular momentum of electron in the second orbit of Bohr’s model of hydrogen atom ?
Answer:
Angular momentum of electron in second orbit of Hydrogen atom
L = \(\frac{2 \mathrm{~h}}{2 \pi}\) = \(\frac{h}{\pi}\) (∵ L = \(\frac{h h}{2 \pi}\))
Question 2.
What is the expression for fine structure constant and what is its value ?
Answer:
Formula for fine structure constant
α = \(\frac{\mathrm{e}^2}{2 \varepsilon_0 \mathrm{ch}}\); value of α = \(\frac{1}{137}\)
![]()
Question 3.
What is the physical meaning of ‘negative energy of an electron’ ?
Answer:
The ‘negative energy of an electron’ indicates that the electron bound to the nucleus due to force of attraction.
Question 4.
Sharp lines are present in the spectrum of a gas. What does this indicate ?
Answer:
Sharp lines in the spectrum of gas indicates bright lines against dark background.
Question 5.
Name a physical quantity whose dimensions are the same as those of angular momentum.
Answer:
Planck’s constant.
Question 6.
What is the difference between α – particle and helium atom ?
Answer:
Alpha particle
- It is a + 2e charged Helium nucleus.
- It contains 2 protons and 2 neutrons.
Helium atom
- It has no charge.
- It contains 2 protons, 2 electrons and 2 neutrons.
Question 7.
How is impact parameter related to angle of scattering ?
Answer:
The impact parameter related to angle of scattering is given by b = \(\frac{1}{4 \pi \varepsilon_0} \times \frac{\mathrm{Ze}^2}{\left(\frac{1}{2} m v^2\right)} \cot \theta_2\)
Question 8.
Among alpha, beta and gamma radiations, which get affected by the electric field ?
Answer:
Alpha and Beta radiations are get affected by the electric field.
Question 9.
What do you understand by the ‘phrase ground state atom’ ?
Answer:
If the electron is present in the ground state, it is called ground state atom.
Question 10.
Why does the mass of the nucleus not have any significance in scattering in Rutherford’s experiment ?
Answer:
The size of the atom is 10-10 m and size of the nucleus is 10-15 m. Hence atom has large empty space. So the mass of nucleus has no significance in Rutherford’s scattering experiment.
Question 11.
The Lyman series of hydrogen spectrum lies in the ultraviolet region. Why ? (A.P. Mar. ’15)
Answer:
The calculated values of wavelengths lie in the ultraviolet region of the spectrum well agree with the values of wavelengths observed experimentally by Lyman.
Question 12.
Write down a table giving longest and shortest wavelengths of different spectral series.
Answer:
Wavelength limits of some spectral series of hydrogen.
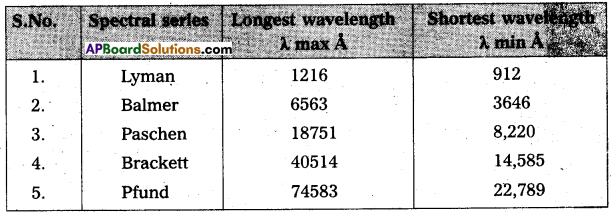
Question 13.
The wavelengths of some of the spectral lines obtained in hydrogen spectrum are 1216 A, 6463 A and 9546A. Which one of these wavelengths belongs to the Paschen series ?
Answer:
The wavelength of spectral line 9546A belongs to the Paschen series.
![]()
Question 14.
Give two drawbacks of Rutherford’s atomic model.
Answer:
Drawbacks of Rutherford’s atom model:
- As the revolving electron loses energy continuously, it must spiral inwards and eventually fall into the nucleus. But matter is stable, we can not expect the atom collapse.
- The atoms should emit continuous spectrum, but what we observe is only a line spectrum.
Short Answer Questions
Question 1.
What is impact parameter and angle of scattering ? How are they related to each other ?
Answer:
- Impact parameter (b) : Impact parameter is defined as the perpendicular distance of the initial velocity vector of the alpha particle from the central line of the nucleus, when the particle is far away from the nucleus of the atom.
- Scattering angle (θ): The scattering angle (θ) is the angle between the asymtotic direction of approach of the α – particle and the asymptotic direction in which it receeds.
- The relation between b and θ is b = \(\frac{1}{4 \pi \varepsilon_0} \frac{Z \mathrm{e}^2}{E} \cot \frac{\theta}{2}\) where E = K.E. of α – particle = \(\frac{1}{2} \mathrm{mv}^2\)
Question 2.
Derive an expression for potential and kinetic energy of an electron in any orbit of a hydrogen atom according to Bohr’s atomic model. How does P.E change with increasing n. (T.S. Mar. ’15)
Answer:
- According fo Bohr electrostatic force of attraction, Fe between the revolving electrons and nucleus provides the necessary centripetal force Fc to keep them in their orbits.
- Thus for dynamically state orbit in a hydrogen atom.
Fc = Fe ⇒ \(\frac{m v^2}{r}\) = \(\frac{1}{4 \pi \varepsilon_0} \frac{e^2}{r_2}\) - The relation between the orbit radius and the electron velocity is r = 2
4e0 (m ) - The kinetic energy (K) and electrostatic potential energy (υ) of the electron in hydrogen atom are
K = \(\frac{1}{2} \mathrm{mv}^2\) = \(\frac{\mathrm{e}^2}{8 \pi \varepsilon_0 r}\) and υ = \(\frac{-\mathrm{e}^2}{4 \pi \varepsilon_0 \mathrm{r}}\) - The total energy E of the electron in a hydrogen atom is
E = K + U = \(\frac{\mathrm{e}^2}{8 \pi \varepsilon_0 \mathrm{r}}\) – \(\frac{\mathrm{e}^2}{4 \pi \varepsilon_0 \mathrm{r}}\)
∴ E = \(\frac{-\mathrm{e}^2}{8 \pi \varepsilon_0 \mathrm{r}}\) - With increase in ‘nr potential energy (U) also increases.
Question 3.
What are the limitations of Bohr’s theory of hydrogen atom ? (Mar. ’14)
Answer:
Limitations of Bohr’s theory of Hydrogen atom :
- This theory is applicable only to simplest atom like hydrogen, with z = 1. The theory fails in case of atoms- of other elements for which z > 1.
- The theory does not explain why orbits of electrons are taken as circular, while elliptlical orbits are also possible.
- Bohr’s theory does not say anything about the relative intensities of spectral lines.
- Bohr’s theory does not take into account the wave properties of electrons.
Question 4.
Explain the distance of closest approach and impact parameter.
Answer:
Distance of closest approach :
- Suppose an α-particle with initial kinetic energy (K.E) is directed towards the centre of the nucleus of an atom.
- On account of Coulomb’s repulsive force between nucleus and alpha particle, kinetic energy of alpha particle goes on decreasing and in turn, electric potential energy of the particle goes on increasing.
- At certain distance ‘d’ from the nucleus, K. E of α-particle reduces to zero. The particle stops and it can not go closer to the nucleus. It is repelled by the nucleus and therefore it retraces its path, turning through 180°.
- Therefore, the distance d is known as the distance of closest of approach.
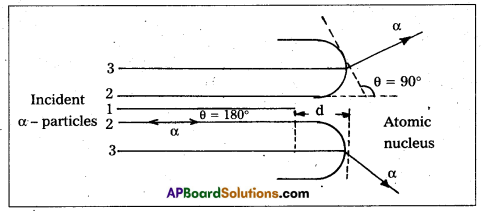
The closest distance of approach,
d = \(\frac{1}{4 \pi \varepsilon_0} \times \frac{Z e^2}{\left(\frac{1}{2} m v^2\right)}\)
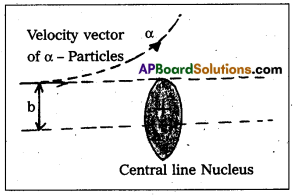
- Impact parameter (b) : Impact parameter is defined as the ⊥r distance of the initial velocity vector of the α – particle from the central line of the nucleus, when the particle is far away from the nucleus of the atom.
Question 5.
Give a brief account of Thomson model of atom. What are its limitations ?
Answer:
Thomson’s model of atom :
- According to Thomson’s model, every atom consists of a positively charged sphere of radius of the order of 10-10 m in which entire mass and positive charge of the atom are uniformly distributed.
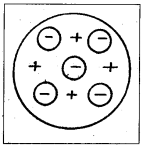
- Inside this sphere, the electrons are embedded like seeds in a watermelon or like plums in a pudding.
- The negative charge of electrons is equal to the positive charge of the atom. Thus atom is electrically neutral.
Limitations :
- It could not explain the origin of spectral series of hydrogen and other atoms, observed experimentally.
- It could not explain large angle scattering of a-particles from thin metal foils, as observed by Rutherford.
Question 6.
Describe Rutherford atom model. What are the draw backs of this model.
Answer:
Rutherford atom model: The essential features of Rutherford’s nuclear model of the atom or planetary model of the atom are as follows :
- Every atom consists of tiny central core, called the atomic nucleus, in which the entire positive charge and almost entire mass of the atom are concentrated.
- The size of nucleus is of the order of 10-15m, which is very small as compared to the size of the atom which is of the order of 10-10m.
- The atomic nucleus is surrounded by certain number of electrons. As atom on the whole is electrically neutral, the total negative charge of electrons surrounding the nucleus is equal to total positive charge on the nucleus.
- These electrons revolve around the nucleus in various circular orbits as do the planets around the sun. The centripetal force required by electron for revolution in provided by the electrostatic force of attraction between the electrons and the nucleus.
Draw backs : According to classical E.M. theory.
- The revolving electron loses energy continuously, it must spiral inwards and eventually fall into the nucleus. As matter is stable, we cannot expect the atoms to collapse.
- Since the frequency of radiation emitted is the same as the frequency of revolution, the atom should radiate a continuous spectrum, but what we observe is only a line spectrum.
Question 7.
Distinguish between excitation potential and ionization potential.
Answer:
Excitation Potential:
1) When the electron jumps from lower orbit to higher orbit by absorbing energy is called excited electron and the process is known as excitation. The minimum accelerating potential which provides an electron energy sufficient to jump from the inner most orbit (ground state) to one of the outer orbits is called excitation potential or resonance potential.
2) a) For example, in case of hydrogen atom,
E1 = -13.6 eV. E2 = -3.4 eV E3 = -1.51eV and soon, E∞ = 0
∴ Energy required to raise an electron from ground state (n = 1) to first excited state
(n = 2) is E = E2 – E1 = -3.4 – (-13.6) = 10.2 eV.
The corresponding excitation potential = 10.2 Volt,
b) Similarly, energy required to raise an electron from ground state (n = 1) to second excited , state (n = 3) is
E = E3 – E1 = -1.51 – (-13.6) = -1.51 + 13.6 = 12.09 eV
The corresponding excitation potential = 12.09 Volt and so on.
3) The excitation potential of an atom is not one. It can have many values, depending on the state to which the atom is excited.
Ionisation potential:
- The energy supplied is so large that it can remove an electron from the outer most orbit of an atom, the process is called Ionisation. Thus ionisation is the phenomenon of removal of an electron from the outer most orbit of an atom.
- The minimum accelerating potential which would provide an electron energy sufficient just to remove it from the atom is called Ionisation potential.
- For example, total energy of electron in ground state of hydrogen atom, + 13.6 eV energy is required.
∴ Ionisation energy of hydrogen atom = 13.6 eV.
Ionisation potential of hydrogen atom = 13.6 Volts. - The general expression for ionisation potential of an atom is V = \(\frac{13.6 \mathrm{Z}^2}{\mathrm{n}^2}\) volt, Where Z is the charge number of the atom and n is number of orbit from which electron is to be removed.
- For a given element, ionisation potential is fixed, but for different elements, ionisation potentials are different.
![]()
Question 8.
Explain the different types of spectral series in hydrogen atom. (A.P. Mar. ’19, ’15; T.S. Mar. ’16)
Answer:
The atomic hydrogen emits a line spectrum consisting of five series.
- Lyman series : v = Rc \(\left(\frac{1}{1^2}-\frac{1}{n^2}\right)\) where n = 2, 3, 4, ……
- Balmer series : v = Rc\(\left(\frac{1}{2^2}-\frac{1}{n^2}\right)\) where n = 3, 4, 5, ………
- Paschen series : v = Rc\(\left(\frac{1}{3^2}-\frac{1}{n^2}\right)\) where n = 4, 5, 6, …….
- Brackett series : v = Rc\(\left(\frac{1}{4^2}-\frac{1}{n^2}\right)\) where n = 5, 6, 7, ……
- Pfund series : v = Rc\(\left(\frac{1}{5^2}-\frac{1}{n^2}\right)\) where n = 6, 7, 8,……..
Question 9.
Write a short note on Debroglie’s explanation of Bohr’s second postulate of quantization.
Answer:
Debroglie’s explanation of Bohr’s second postulate of quantization :
- The second postulate of Bohr atom model says that angular momentum of electron orbiting around the nucleus is quantized i.e., mυr = \(\frac{\text { nh }}{2 \pi}\) where m = 1, 2, 3,….
- According to Debroglie, the electron in its circular orbit, as proposed by Bohr, must be seen as a particle wave.
- When a string fixed at two ends is plucked, a large number of wavelengths are excited and standing wave is formed.
- It means that in a string, standing waves form when total distance travelled by a wave down the string and back is an integral number of wavelengths.
- According to Debroglie, a stationary orbit is that which contains an integral number of Debrogile waves associated with the revolving electron.
- For an electron revolving in nth circular orbit of radius rn, total distance covered = circumference òf the orbit = 2πrn
∴ For permissible orbit, 2πrn = nλ - According to Debrogile, λ = \(\frac{h}{m v_n}\) Where υn is speed of electron revolving in nth orbit
∴ mυnrn = \(\frac{\mathrm{nh}}{2 \pi}\) = \(\mathrm{n}\left(\frac{\mathrm{h}}{2 \pi}\right)\)
i.e., angular momentum of electron revolrmg in nth orbit must be an integral multiple of \(\frac{\mathrm{h}}{2 \pi}\), which is the quantum condition proposed by Bohr in second postulate.
Long Answer Questions
Question 1.
Describe Geiger-Marsden Experiment on scattering of α – particles. How is the size of the nucleus estimated in this experiment ?
Answer:
- The experimental set up used by Rutherford and his colaborators, Geiger and Marsden is shown in fig.
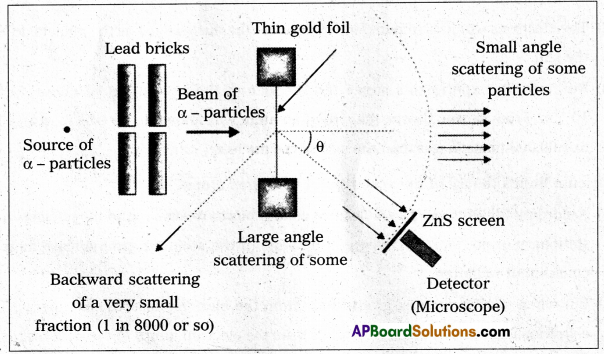
- The α-particles emitted by radio active source contained in a lead cavity are collimated into a narrow beam with the help of a lead slit (collimator).
- The collimated beam is allowed to fall on a thin gold foil of thickness of the order of 2.1 × 10-7m.
- The α-particles scattered in different directions are observed through a rotatable detector consisting of zinc sulphide screen and a microscope.
- The α-particles produce bright flashes or scintillations on the ZnS screen.
- These are observed in the microscope and counted at different angles from the direction of incidence of the beam.
- The angle θ of deviation of an α-particle from its original direction is called its scattering angle θ.
Observations : We find that
- Most of the alpha particles pass straight through the gold foil. It means they do not suffer any collision with gold atoms.
- Only about 0.14% of incident α-particles scatter by more than 1°.
- About one α-particle in every 8000 α-particles deflect by more than 90°.
Estimation of size of the nucleus :
- This led to Rutherford postulate, that the entire positive charge of the atom must be concentration in a tiny central core of the atom. This tiny central core of each atom was called atomic nucleus.
- The electrons would be moving in orbits about the nucleus just as the planets do around the sun.
- Rutherford’s experiments suggested the size of the nucleus to be about 10-15m to 10-14m. From kinetic theory, the size of an atom was known to be 10-10m, about 10,000 to 1,00,000 times larger than the size of the nucleus.
Question 2.
Discuss Bohr’s theory of the spectrum of hydrogen atom.
Answer:
- According to Bohr’s model an electron continuous to revolve round the nucleus in fixed, stationary orbits. This is called groupd state of the atom. In ground state there is no emission of radiation.
- But when some energy is given to an atom the electron absorbs this energy. This is called excited state of the atom. In this state the electron jumps to the next higher orbit. But it can remain 10-8 sec and it immediatly returns back to its ground state and the balance of the energy is emitted out as a spectral line.
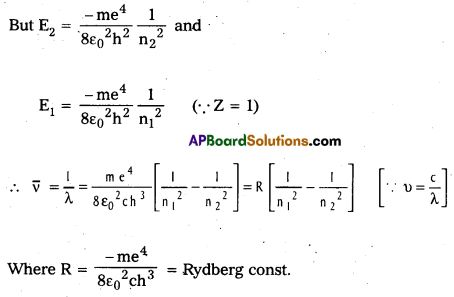
- According to Bohr’s third postulate, the emitted energy is given by E = hv = E2 – E1
Spectral series of Hydrogen atom:
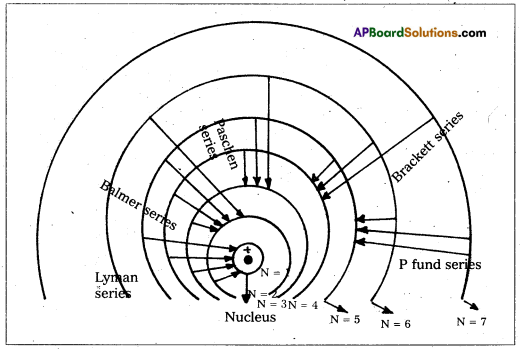
Hydrogen atom has five series of spectral lines: They are
1. Lyman series: When an electron jumps from the outer orbits to the first orbit, the spectral lines are in the ultra – violet region. Here n1 = 1, n2 = 2, 3, 4, 5….
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left[\frac{1}{1^2}-\frac{1}{\mathrm{n}_2^2}\right]\) = \(\mathrm{R}\left[1-\frac{1}{\mathrm{n}_2^2}\right]\)
2) Balmer Series : When an electron jumps from the outer orbits to the second orbit, the
spectral Lines are in the visible region. Here n1 = 2, n2 = 3, 4, 5…
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left[\frac{1}{2^2}-\frac{1}{\mathrm{n}_2^2}\right]\)
3) Paschen series : When an electron jumps from the outer orbits to the third orbit, the spectral lines are in the near infrared region. Here n1 = 3, n2 = 4, 5, 6 ….
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left[\frac{1}{3^2}-\frac{1}{\mathrm{n}_2{ }^2}\right]\)
4) Brackett series : When an electron jumps from outer orbits to the forth orbit, the spectral lines are in the infrared region. Here n1 = 4, n2 = 5, 6, 7…….
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left[\frac{1}{4^2}-\frac{1}{\mathrm{n}_2^2}\right]\)
5) Pfund series : When an electron jumps from outer orbits to the fifth orbit, the spectral lines are in the far infrared region. Here n1 = 5, n2 = 6, 7, 8, ………
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left[\frac{1}{5^2}-\frac{1}{\mathrm{n}_2^2}\right]\)
![]()
Question 3.
State the basic postulates of Bohr’s theory of atomic spectra. Hence obtain an expression for the radius of orbit and the energy of orbital electron in a hydrogen atom.
Answer:
a) Basic postulates of Bohr’s theory are
1) The electron revolves round a nucleus is an atom in various orbits known as stationary orbits. The electrons can not emit radiation when moving in their own stationary levels.
2) The electron can revolve round the nucleus only in allowed, orbits whose angular momentum is the integral multiple of \(momentum is the integral multiple of
i.e., mυnrn = [latex]\frac{\mathrm{nh}}{2 \pi}\) ———> (1)
where n = 1, 2, 3…..
3) If an electron jumps from higher energy (E2) orbit to the lower energy (E1) orbit, the difference of energy is radiated in the form of radiation.
i.e., E = hv = E2 – E1 ⇒ v = \(\frac{E_2-E_1}{h}\) ——> (2)
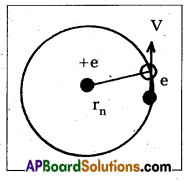
b) Energy of emitted radiation : In hydrogen atom, a single electron of charge — e, revolves around the nucleus of charge e in a ciccular orbit of radius rn.
1) K.E. of electron : For the electron to be in circular orbit, centripetal force = The electrostatic force of attraction between the electron and nucleus,
From Coulomb’s law, \(\frac{\mathrm{m} \dot{v}_n^2}{r_n}\) = \(\frac{\mathrm{Ke}^2}{\mathrm{r}_{\mathrm{n}}^2}\) ——> (3)
where K = \(\frac{1}{4 \pi \varepsilon_0}\) —–> (4)
\(m v_n^2\) = \(\frac{\mathrm{Ke}^2}{\mathrm{r}_{\mathrm{n}}}\) —–> (5)
\(m v^2 r_n\) = Ke2 ——-> (6)
Dividing (5) by (1), υn = Ke2 × \(\frac{2 \pi}{\mathrm{nh}}\)
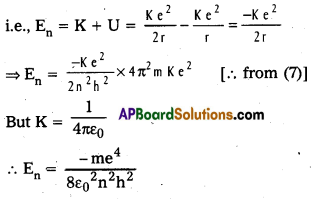
From (3), kinetic energy K = \(\frac{1}{2} m v_n^2\) = \(\frac{\mathrm{Ke}^2}{2 r_{\mathrm{n}}}\)
2) Potential energy of electron:
P.E. of electron, U = \(\frac{\mathrm{Ke}}{\mathrm{r}_{\mathrm{n}}} \times-\mathrm{e}\) [∵ W = \(\frac{I^{\prime}}{4 \pi \varepsilon_0} \frac{Q}{d}\) × -Q]
∴ U = \(\frac{-\mathrm{Ke}^2}{\mathrm{r}_{\mathrm{n}}}\)
3) Radius of the orbit: Substituting the value of (6) in (2),
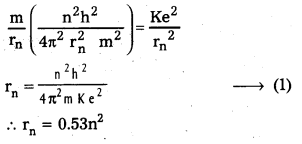
4) Total energy (En) : Revolving electron posses K.E. as well as P.E.
Textual Exercises
Question 1.
The radius of the first electron orbit of a hydrogen atom is 5.3 × 10-11m. What is the radius of the second orbit ?
Solution:
rn ∝ n2
\(\frac{\mathrm{r}_2}{\mathrm{r}_1}\) = \(\frac{2^2}{1^2}\) = \(\frac{4}{1}\) ⇒ r2 = 4r1
Question 2.
Determine the radius of the first orbit of the hydrogen atom. What would be the velocity and frequency of the electron in the first orbit ?
Solution:
Given: h = 6.62 × 10-34 J-s,
m = 9.1 × 10-31kg,
e = 1.6 × 10-19 C,
k = 9 × 109Nm2C-2, n = 1
i)
r1 = \(\frac{n^2 h^2}{4 \pi^2 \mathrm{mke}^2}\)
= \(\frac{(1)^2 \times\left(6.62 \times 10^{-34}\right)^2}{4 \times(3.14)^2 \times 9.1 \times 10^{-31} \times 9 \times 10^9\left(1.6 \times 10^{-19}\right)^2}\)
∴ r1= 0.529 A \(\simeq\) 0.53 A
ii)
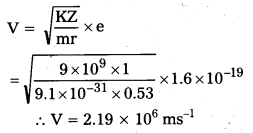
iii)
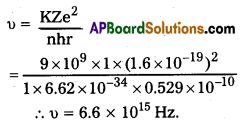
Question 3.
The total energy of an electron in the first excited state of the hydrogen atom is -3.4eV. What is the potential energy of the electron in this state ?
Solution:
In 1st orbit, E = -3.4eV
Total energy E = \(\frac{\mathrm{KZe}^2}{2 \mathrm{r}}\) – \(\frac{\mathrm{KZe}^2}{\mathrm{r}}\)
\(\frac{\mathrm{KZe}^2}{\mathrm{r}}\) = U(say)
E = \(\frac{\mathrm{U}}{2}-\mathrm{U}\) = \(\frac{-\mathrm{U}}{2}\)
U = -2E
∴ U = -2 × -3.4 = 6.8 eV.
Question 4.
The total energy of an electron in the first excited state of hydrogen atom is -3.4eV. What is the kinetic energy of the electron in this state ?
Solution:
In Hydrogen like atom, we know that
K = – Total energy E
Here E = – 3.4eV
∴ K = -(-3.4) = 3.4 eV
![]()
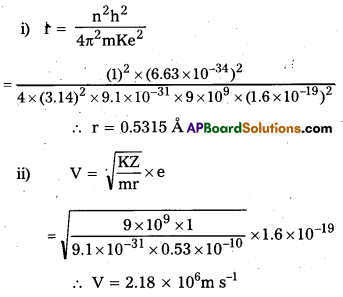
Question 5.
Find the radius of the hydrogen atom in its ground state. Also calculate the velocity of the electron in n = 1 orbit. Given
h = 6.63 × 10-34 J s, m = 9.1 × 10-31 kg, e = 1.6 × 10-19 C, K = 9 × 109N m2C-2
Solution:
n = 1, h = 6.63 × 10-34 J-s,
m = 9.1 × 10-31 kg
e = 1.6 × 10-19C,
K = 9 × 109 Nm2C-2
Question 6.
Prove that the ionisation energy of hydrogen atom is 13.6 eV.
Solution:
n = 1 corresponds to ground state.
E = \(\frac{-13.6}{n^2} e V\)
E = \(\frac{-13.6}{1^2} \mathrm{eV}\)
E = -13.6 eV
∴ The minimum energy required to free the electron from the ground state of hydrogen atom
= 13.6 eV.
∴ Ionisation energy of hydrogen atom = 13.6 eV
Question 7.
Calculate the ionization energy for a lithium atom.
Solution:

∴ Ionization energy of Lithium = 30.6eV.
Question 8.
The wavelength of the first member of Lyman series is 1216 A. Calculate the wavelength of second member of Balmer series.
Solution:
\(\frac{1}{\lambda}\) = \(R\left(\frac{1}{n_1^2}-\frac{1}{n_2^2}\right)\)
For 1st member of Lyman series, λ = 1216; n1 = 1, n2 = 2
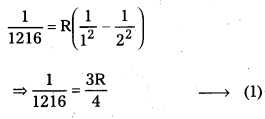
For 2nd member of Balmer senes,

Question 9.
The wavelength of first member of Balmer series is 6563 A. Calculate the wavelength of second member of Lyman series.
Solution:
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left(\frac{1}{\mathrm{n}_1^2}-\frac{1}{\mathrm{n}_2^2}\right)\)
For 1st member of Balmer senes,
\(\frac{1}{6563}\) = \(\mathrm{R}\left(\frac{1}{2^2}-\frac{1}{3^2}\right)\)
\(\frac{1}{6563}\) = \(\frac{5 R}{36}\) —–> (1)
For 2nd member of Lyman senes,
\(\frac{1}{\lambda^1}\) = \(\mathrm{R}\left(\frac{1}{1^2}-\frac{1}{3^2}\right)\)
\(\frac{1}{\lambda^1}\) = \(\frac{8 \mathrm{R}}{9}\) —–> (2)
\(\frac{(1)}{(2)}\) ⇒ \(\frac{\lambda^1}{6563}\) = \(\frac{5 \mathrm{R}}{36} \times \frac{9}{8 \mathrm{R}}\)
λ’ = \(\frac{5}{32} \times 6563\)
∴ λ’ = 1025.5A
Question 10.
The second member of Lyman series in hydrogen spectrum has wavelength 5400 A. Find the wavelength of first member.
Solution:
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left(\frac{1}{\mathrm{n}_1^2}-\frac{1}{\mathrm{n}_2^2}\right)\)
For second member of Lyman senes,
\(\frac{1}{5400}\) = \(R\left(\frac{1}{1^2}-\frac{1}{3^2}\right)\) ⇒ \(\frac{1}{5400}\) = \(\frac{8 \mathrm{R}}{9}\) —-> (1)
For first member of Lyman series,
\(\frac{1}{\lambda^1}\) = \(\mathrm{R}\left(\frac{1}{1^2}-\frac{1}{2^2}\right)\)
\(\frac{1}{\lambda^1}\) = \(\frac{3 R}{4}\) —–> (2)
\(\frac{(1)}{(2)}\) ⇒ \(\frac{\lambda^1}{5400}\) = \(\frac{8 R}{9} \times \frac{4}{3 R}\)
∴ λ’ = \(\frac{32}{27}\) × 5400 = 6400A.
Question 11.
Calculate the shortest wavelength of Balmer series. Or Calculate the wavelength of the Balmer senes limit. Given : R = 10970000m-1.
Solution:
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left(\frac{1}{\mathrm{n}_1^2}-\frac{1}{\mathrm{n}_2^2}\right)\)
R = 10970000 = 1.097 × 107 ms-1
For Balmer senes limit n1 = 2 and n2 = ∞
\(\frac{1}{\lambda}\) = \(\mathrm{R}\left(\frac{1}{2^2}-\frac{1}{\infty}\right)\) ⇒ \(\frac{1}{\lambda}\) = \(\frac{R}{4}\)
λ = \(\frac{4}{\mathrm{R}}\) = \(\frac{4}{1.097 \times 10^7}\) = 3646.3A
![]()
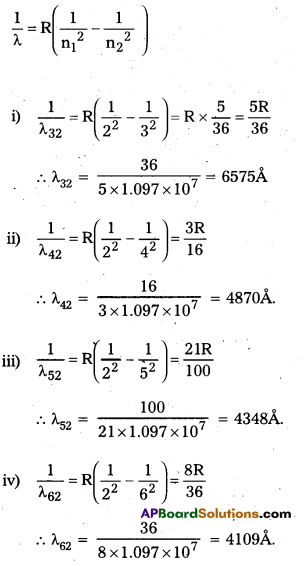
Question 12.
Using the Rydberg formula, calcûlate the wavelength of the first four spectral lines in the Balmer series of the hydrogen spectrum.
Solution:
Additional Exercises
Question 1.
Choose the correct alternative from the clues given at the end of the each statement:
a) The size of the atom in Thomson’s model is ……… the atomic size in Rutherford’s model. (much greater than / no different from / much less than.)
b) In the ground state of ……. electrons are in stable equilibrium, while in …… electrons always experience a net force. (Thomson’s model / Rutherford’s model.)
c) A classical atom based on …… is doomed to collapse. (Thomson’s model / Rutherford’s model).
d) An atom has a nearly continuous mass distribution in a ……. but has a highly non-uniform mass distribution in ………. (Thomson’s model / Rutherford’s model.)
e) The positively charged part of the atom possesses most of the mass in …….. (Rutherford’s model / both the models.)
Answer:
a) No different from
b) Thomson’s model, Rutherford’s model
c) Rutherford’s model
d) Thomson’s model, Rutherford’s model
e) Both the models.
Question 2.
Suppose you are given a chance to repeat the alpha – particle scattering experiment using a thin sheet of solid hydrogen in place of the gold foil. (Hydrogen is a solid at temperatures below 14 K.) What results do you expect ?
Answer:
The basic purpose of scattering experiment is defeated because solid hydrogen will be much lighter target compared to the alpha particle acting as projectile. According to theory of elastic the collisions, the target hydrogen will move much faster compared to alpha after collision. We cannot determine the size of hydrogen nucleus.
Question 3.
What is the shortest wavelength present in the Paschen series of spectral lines ?
Answer:
From Rydberg’s formula
\(\frac{\mathrm{hc}}{\lambda}\) = 13.6 × 1.6 × 10-19\(\left(\frac{1}{\mathrm{n}_1^2}-\frac{1}{\mathrm{n}_2^2}\right)\)
For shortest wavelength in Paschen series n2 = ∞ and n1 = 3
\(\frac{\mathrm{hc}}{\lambda}\) = 21.76 × 10-19\(\left[\frac{1}{3^2}-\frac{1}{\infty^2}\right]\)
= 2.42 × 10-19
λ = \(\frac{\mathrm{hc}}{2.42 \times 10^{-19}}\) = \(\frac{6.6 \times 10^{-34} \times 3 \times 10^8}{2.42 \times 10^{-19}} \mathrm{~m}\)
= 8.1818 × 10-7m = 818.18nm.
Question 4.
A difference of 2.3 eV separates two energy levels in an atom. What is the frequency of radiation emitted when the atom make a transition from the upper level to the lower level ?
Answer:
Here E = 2.3eV = 2.3 × 1.6 × 10-19 J
As E = hv
∴ v = \(\frac{\mathrm{E}}{\mathrm{h}}\) = \(\frac{2.3 \times 1.6 \times 10^{-19}}{6.6 \times 10^{-34}}\) = 5.6 × 104 Hz
Question 5.
The ground state energy of hydrogen atom is -13.6 eV. What are the kinetic and potential energies of the electron in this state ?
Answer:
Total energy E = -13.6 eV
K.E = -E = 13.6 eV
RE. = -2.K.E = -2 × 13.6
= -27.2eV.
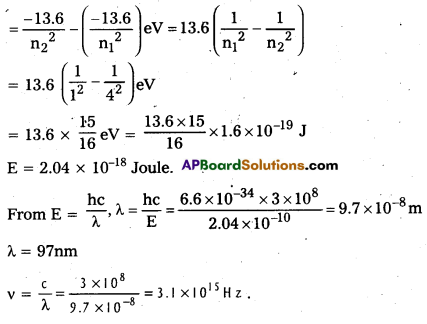
Question 6.
A hydrogen atom initially in the ground level absorbs a photon, which excites it to the n = 4 level. Determine the wavelength and frequency of photon.
Answer:
For ground state n1 = 1 and n2 = 4
Energy of photon absorbed E = E2 – E1
Question 7.
a) Using the Bohr’s model calculate the speed of the electron in a hydrogen atom in the n = 1, 2 and 3 levels.
b) Calculate the orbital period in each of these levels.
Answer:
a) From v = \(\frac{c}{n} \alpha\), where α = \(\frac{2 \pi \mathrm{Ke}^2}{\mathrm{ch}}\) = 0.0073
v1 = \(\frac{3 \times 10^8}{1}\) × 0.0073 = 2.19 × 106 m/s
v2 = \(\frac{3 \times 10^8}{2}\) × 0.0073 = 1.095 × 106 m/s
v3 = \(\frac{3 \times 10^8}{3}\) × 0.0073 = 7.3 × 105 m/s
b) Orbital period, T = \(\frac{2 \pi r}{V}\), As r1 = 0.53 × 10-10m
T1 = \(\frac{2 \pi \times 0.53 \times 10^{-10}}{2.19 \times 10^6}\) = 1.52 × 10-16S
As r2 = 4r1 and V2 = \(\frac{1}{2} V_1\)
T2 = 8T2 = 8 × 1.52 × 10-6 S = 1.216 × 10-15S
As r3 = 9r1 and V3 = \(\frac{1}{3} \mathrm{~V}_1\)
T3 = 27T1= 27 × 1.52 × 10-16 S = 4.1 × 10-15S
Question 8.
The radius of the innermost electron orbit of a hydrogen atom is 5.3 × 10--11m. What are the radii of the n = 2 and n = 3 orbits?
Answer:
As r = n2r
∴ r2 = 4r1 = 4 × 5.3 × 10-11 m = 2.12 × 10-10m ,
and r3 = 9r1 = 9 × 5.3 × 10-11 = 4.77 × 10-10m.
Question 9.
A 12.5 eV electron beam is used to bombard gaseous hydrogen at room temperature. What series of wavelengths will be emitted?
Answer:
In ground state, energy of gaseous hydrogen at room temparature = -13.6eV, when it is bombarded with 12.5 eV electron beam, the energy becomes 13.6 + 12.5 = -1.1eV.
The electron would jump from n = 1 to n = 3 where E3 = \(\frac{-13.6}{32}\) = -1.5eV
On de — excitation the electron may jump from n = 3 to n = 2 giving rise to Balmer series. It may also jump from n = 3 to n = 1 giving rise to Lýman series.
![]()
Question 10.
In accordance with the Bohr’s model, find the quantum number that characterises the earths revolution around the sun in an orbit of radius 1.5 × 1011 m with orbital speed 3 × 104m/s. (Mass of earth = 6.0 × 1024 kg.)
Answer:
Here r = 1.5 × 1011m, V = 3 × 104m/s, m = 6.0 × 1024kg
According to Bohrs model mvr = \(\frac{\mathrm{nh}}{2 \pi}\)
n = \(\frac{2 \pi \mathrm{mvr}}{\mathrm{h}}\) = 2 × \(\frac{22}{7}\) × \(\frac{6.0 \times 10^{24} \times 3 \times 10^4 \times 1.5 \times 10^{11}}{6.6 \times 10^{-34}}\)
= 2.57 × 1074, which is too large.
Question 11.
Answer the following questions, which help you understand the difference between Thomson’s model and Rutherford’s model better.
a) Is the average angle of deflection of α — particles by a thin gold foil predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model?
b) Is the probability of backward scattering (i.e., scattering of α – particles at angles greater than 90°) predicted by Thomson’s model much less, about the same, or much greater than that predicted by Rutherford’s model ?
c) Keeping other factors fixed, it is found experimentally that for small thickness t, the number of α – particles scattered at moderate angles is proportional to t. What clue does this linear dependence on t provide ?
d) In which model is it completely wrong to ignore multiple scattering for the calculation of average angle of scattering of a – particles by a thin foil ?
Answer:
a) About the same this is because we are talking of average angle of deflection.
b) Much less, because in Thomson’s model there is no such massive central core called the nucleus as in Rutherford’s model.
c) This suggests that scattering is predominantly due to a single collision increases with the number of target atoms which increases linearly with the thickness of the foil.
d) In Thomson model, positive charge is uniformly distributed in the spherical atom. Therefore a single collision causes very little deflection. Therefore average scattering angle can be explained only by considering multiple scattering may be ignored as a first approximation.
Question 12.
The gravitational attraction between electron and proton in a hydrogen atom is weaker than the coulomb attraction by a factor of about 10-40. An alternative way of looking at this fact is to estimate the radius of the first Bohr orbit of a hydrogen atom if the electron and proton were bound by gravitational attraction. You will find the answer interesting.
Answer:
The radius of the first Bohr orbit of a hydrogen atom is
r0 = \(\frac{4 \pi \varepsilon_0(h / 2 \pi)^2}{m_e \mathrm{e}^2}\)
If we consider the atom bound by the gravitational force
= \(\left(\frac{\mathrm{Gm}_{\mathrm{p}} \mathrm{m}_{\mathrm{e}}}{\mathrm{r}^2}\right)\). We should replace \(\frac{\mathrm{e}^2}{4 \pi \varepsilon_0}\) by (Gmpme). In that case radius of first Bohr orbit of hydrogen atom would be given by r0 = \(\frac{(\mathrm{h} / 2 \pi)^2}{\mathrm{Gm}_p \mathrm{~m}^2 \mathrm{e}}\)
Putting the standard values we get
r0 = \(\frac{\left(6.6 \times 10^{-34} / 2 \pi\right)^2}{6.67 \times 10^{-11} \times 1.67 \times 10^{-27} \times\left(9.1 \times 10^{-31}\right)^2}\)
= 1.2 × 1029 metre.
This is much greater than the estimated size of the whole universe!
Question 13.
Obtain an expression for the frequency of radiation emitted when a hydrogen atom de- excites from level n to level (n – 1). For large n, show that this frequency equals the classical frequency of revolution of the electron in the orbit.
Answer:
The frequency v of the emitted radiation when a hydrogen atom de-excites from level n to level (n – 1) is
E = hv = E2 – E1
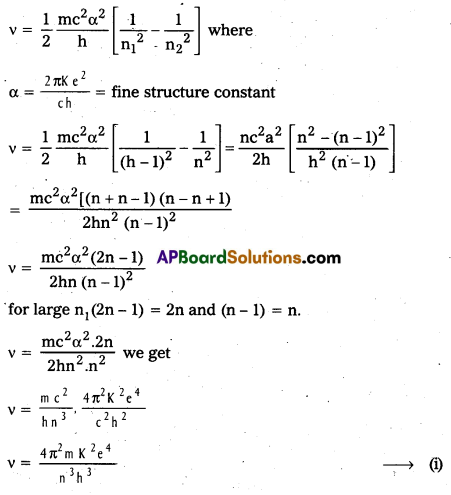
In Bohr’s Atomic model, velocity of electron in nth orbit is v = \(\frac{\mathrm{nh}}{2 \pi \mathrm{mr}}\)
and radius of nth orbital is v = \(\frac{n^2 h^2}{4 \pi^2 m K e^2}\)
Frequency of revolution of electron v = \(\frac{\mathrm{V}}{2 \pi \mathrm{r}}\) = \(\frac{\mathrm{nh}}{2 \pi \mathrm{mr}}\)
and radius of nth orbital is r = \(\frac{n^2 h^2}{4 \pi^2 m K e^2}\)
Frequency of revolution of electron
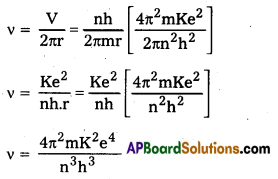
which is the same as (i) .
Hence for large values of n1 classical frequency of revolution of electron in nth orbit is the same as the frequency of radiation emitted when hydrogen atom de-excites from level (n) to level (n – 1)
![]()
Question 14.
Classically, an electron can be in any orbit around the nucleus of an atom. Then what determines the typical atomic size ? Why is an atom not, say, thousand times bigger than its typical size ? The question had greatly puzzled Bohr before he arrived at his famous model of the atom that you have learnt in the text. To simulate what he might well have done before his discovery, let us play as follows with the basic constants of nature and see if we can get a quantity with the dimensions of length that is roughly equal to the known size of an atom (~ 1010m).
a) Construct a quantity with the dimensions of length from the fundamental constants e, me, and c. Determine its numerical value.
b) You will find that the length obtained in (a) is many orders of magnitude smaller than the atomic dimensions. Further, it involves c. But energies of atoms are mostly in non- relativistic domain where c is not expected to play any role. This is what may have suggested Bohr to discard c and look for ‘something else’ to get the right atomic size. Now, the ‘Planck’s constant h had already made its appearance elsewhere. Bohr’s great insight lay in recognising that h, me, and e will yield the right atomic size. Construct a quantity with the dimension of length from h, me, and e and confirm that its numerical value has indeed the correct order of magnitude.
Answer:
a) Using fundamental constants e, me and c, we construct a quantity which has the dimensions of length. This quantity is \(\left(\frac{\mathrm{e}^2}{4 \pi \varepsilon_0 \mathrm{~m}_{\mathrm{e}} \mathrm{c}^2}\right)\)
Now \(\frac{\mathrm{e}^2}{4 \pi \varepsilon_0 \mathrm{~m}_{\mathrm{e}} \mathrm{c}^2}\) = \(\frac{\left(1.6 \times 10^{-19}\right)^2 \times 9 \times 10^9}{9.1 \times 10^{-31}\left(3 \times 10^8\right)^2}\) = 2.82 × 10-15m
This is of the order of atom sizes.
b) However when we drop c and use hc, me and e to construct a quantity which has dimensions of length the quantity we obtain is
\(\frac{4 \pi \varepsilon_0(\mathrm{~h} / 2 \pi)}{\mathrm{m}_{\mathrm{e}} \mathrm{e}^2}\)
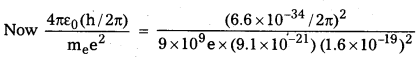
= 0.53 × 10-10m
This is of the order of atom sizes.
Question 15.
The total energy of an electron in the first excited state of the hydrogen atom is about -3.4 eV
a) What is the kinetic energy of the electron in this state ?
b) What is the potential energy of the electron in this state ?
c) Which of the answers above would change if the choice of the zero of potential energy is changed ?
Answer:
We know kinetic energy of electron = \(\frac{K Z e^2}{2 r}\)
and P.E of electron = \(\frac{-\mathrm{KZe}^2}{\mathrm{r}}\)
P.E. = -2 (kinetic energy).
In this calculation electric potential and hence potential energy is zero at infinity.
Total energy = PE + KE = -2KE + KE = -KE
a) In the first excited state total energy = -3.4eV
∴ K.E = -(-3.4eV) = + 3.4 eV
b) P. E of electron in this first excited state = -2KE = -2 × 3.4 = -6.8eV.
c) If zero of potential energy is changed, KE does not change and continues to be +3.4 eV However, the P.E. and total energy of the state would change with the choice of zero of potential energy.
Question 16.
If Bohr’s quantisation postulate (angular momentum = nh/2π) is a basic law of nature, it should be equally valid for the case of planetary motion also. Why then do we never speak of quantisation of orbits of planets around the sun ?
Answer:
Bohr’s quantisation postulate is in terms of Plank’s constant (h), But angular momenta associated with planetary motion are = 1070 h (for earth). In terms of Bohr’s quantisation posulate this will correspond to n = 107. For such large values of n the differences in successive energies and angular momenta of the quantised levels are so small, that the levels can be considered as continuous and not discrete.
Question 17.
Obtain the first Bohr’s radius and the ground state energy of a muonic hydrogen atom [i.e., an atom in which a negatively charged muon (µ–) of mass about 207me orbits around a proton].
Answer:
A muonic hydrogen is the atom in which a negatively charged muon of mass about 207 me revolves around a proton.
In Bohr’s atom model as, r ∝ \(\frac{1}{\mathrm{~m}}\)
\(\frac{\mathrm{r}_\mu}{\mathrm{r}_{\mathrm{e}}}\) = \(\frac{\mathrm{m}_{\mathrm{e}}}{\mathrm{m}_\mu}\) = \(\frac{\mathrm{m}_{\mathrm{e}}}{207 \mathrm{~m}_{\mathrm{e}}}\) = \(\frac{1}{207}\)
Here re is radius of first orbit of electron in hydrogen atom = 0.53A = 0.53 × 10-10m.
rm = \(\frac{\mathrm{r}_{\mathrm{e}}}{207}\) = \(\frac{0.53 \times 10^{-10}}{207}\) = 2.56 × 10-13m
Again in Bohr’s atomic model
E ∝ m
∴ \(\frac{\mathrm{E}_\mu}{\mathrm{E}_{\mathrm{e}}}\) = \(\frac{\mathrm{m}_\mu}{\mathrm{m}_{\mathrm{e}}}\) = \(\frac{207 \mathrm{~m}_{\mathrm{e}}}{\mathrm{m}_{\mathrm{e}}}\), Eμ = 207Ee
As ground state energy of electron in hydrogen atom is Ee = -13.6 eV
Eμ = 207(-13.6)eV = -2815.2eV
= -2.8152KeV.